An equilateral prism of refractive index μ=4√3 is kept in a medium of refractive index μ1. Consider a light ray to be normally incident on one of the refracting faces. The diagram shows variation of magnitude of angle of deviation (β) with respect to μ1.
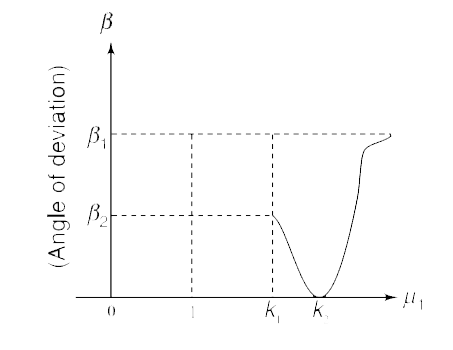
(a) Find value of k2.
(b) Find value of k1.
The correct Answer is:(a) 4√3 (b) 83
Step by step video, text & image solution for An equilateral prism of refractive index mu = (4)/(sqrt(3)) is kept in a medium of refractive index mu_(1). Consider a light ray to be normally incident on one of the refracting faces. The diagram shows variation of magnitude of angle of deviation (beta) with respect to mu_(1). (a) Find value of k_(2). (b) Find value of k_(1). by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - ARIHANTCHAPTER - GEOMETRICAL OPTICSEXERCISE - Level 3
9 Videos
- GEOMETRICAL OPTICSBOOK - ARIHANTCHAPTER - GEOMETRICAL OPTICSEXERCISE - Level 3
9 Videos
- ELECTROSTATICSBOOK - ARIHANTCHAPTER - ELECTROSTATICSEXERCISE - Level 3
59 Videos
- MAGNETIC EFFECT OF CURRENTBOOK - ARIHANTCHAPTER - MAGNETIC EFFECT OF CURRENTEXERCISE - Magnetic effect of Current
45 Videos
Similar Questions
Knowledge Check
A lens made of material of Refractive index μ2 is surrounded by a medium of Refractive Index μ1. The focal length f is related as
Af∝(μ2−μ1)Bf∝μ1(μ2−μ1)Cf∝(μ2+μ1)Df∝1(μ2+μ1)
A right angled triangular prism of refractive index μ1 is kept floating on a liquid (surface) having refractive index μ2. The condition such tat light incident normally passes through base of prism as shown in figure.Aμ1=Cos2θ/CosθBμ2=Cos2θ/CosθCμ1=Sin2θ/CosθDμ2=Sin2θ/SinθA convex lens made up of material of refractive index μ1, is immersed in a medium of refractive index μ2 as shown in the figure. The relation between μ1 and μ2 is
 Aμ1<μ2Bμ1>μ2Cμ1=μ2Dμ1=√μ2
Aμ1<μ2Bμ1>μ2Cμ1=μ2Dμ1=√μ2
A prism of refractive index √2 has refractive angle 60∘ . Find the angle of incidence so that a ray minimum deviation.
View SolutionAn equilateral glass prism of refractive index 1.5 is immersed in water. Find the angle of deviation produced when a ray of light is incident at 42∘ on one face of the prism.
(Refractive index of water = 1.33)
View SolutionA concave lens having a material of refractive index μ1 is kept in a medium of R.I. μ2, where μ1<μ2. A parallel beam of light is incident on the lens. The concave lens will act as
View SolutionThere is an equilateral prism of material of refractive index 1.5. Light ray is incident normally on its surface. What will be the angle of deviation of ray ?
View SolutionLight travels from a medium of refractive index μ1 to another of refractive index μ2(μ1>μ2). For total internal reflection of light, which is NOT true?
View Solution

