The end-point P of a line-segment PQ is against 4cm mark and the end-point Q is against the mark indicating 14.8 cm on a ruler. What is the length of the segment PQ?
To find the length of the line segment PQ, we can follow these steps:
1. Identify the positions of points P and Q on the ruler:
- Point P is at the 4 cm mark.
- Point Q is at the 14.8 cm mark.
2. Determine the length of the segment PQ:
- The length of the segment PQ can be calculated by finding the difference between the positions of points Q and P.
- This can be expressed mathematically as:
PQ=Q−P
3. Substitute the values into the equation:
- Substitute the values of Q and P:
PQ=14.8cm−4cm
4. Perform the subtraction:
- Calculate the difference:
PQ=14.8−4=10.8cm
5. State the final answer:
- The length of the line segment PQ is 10.8cm.
Final Answer:
The length of the segment PQ is 10.8cm.
- Point P is at the 4 cm mark.
- Point Q is at the 14.8 cm mark.
- The length of the segment PQ can be calculated by finding the difference between the positions of points Q and P.
- This can be expressed mathematically as:
PQ=Q−P
- Substitute the values of Q and P:
PQ=14.8cm−4cm
- Calculate the difference:
PQ=14.8−4=10.8cm
- The length of the line segment PQ is 10.8cm.
The length of the segment PQ is 10.8cm.
Topper's Solved these Questions
Similar Questions
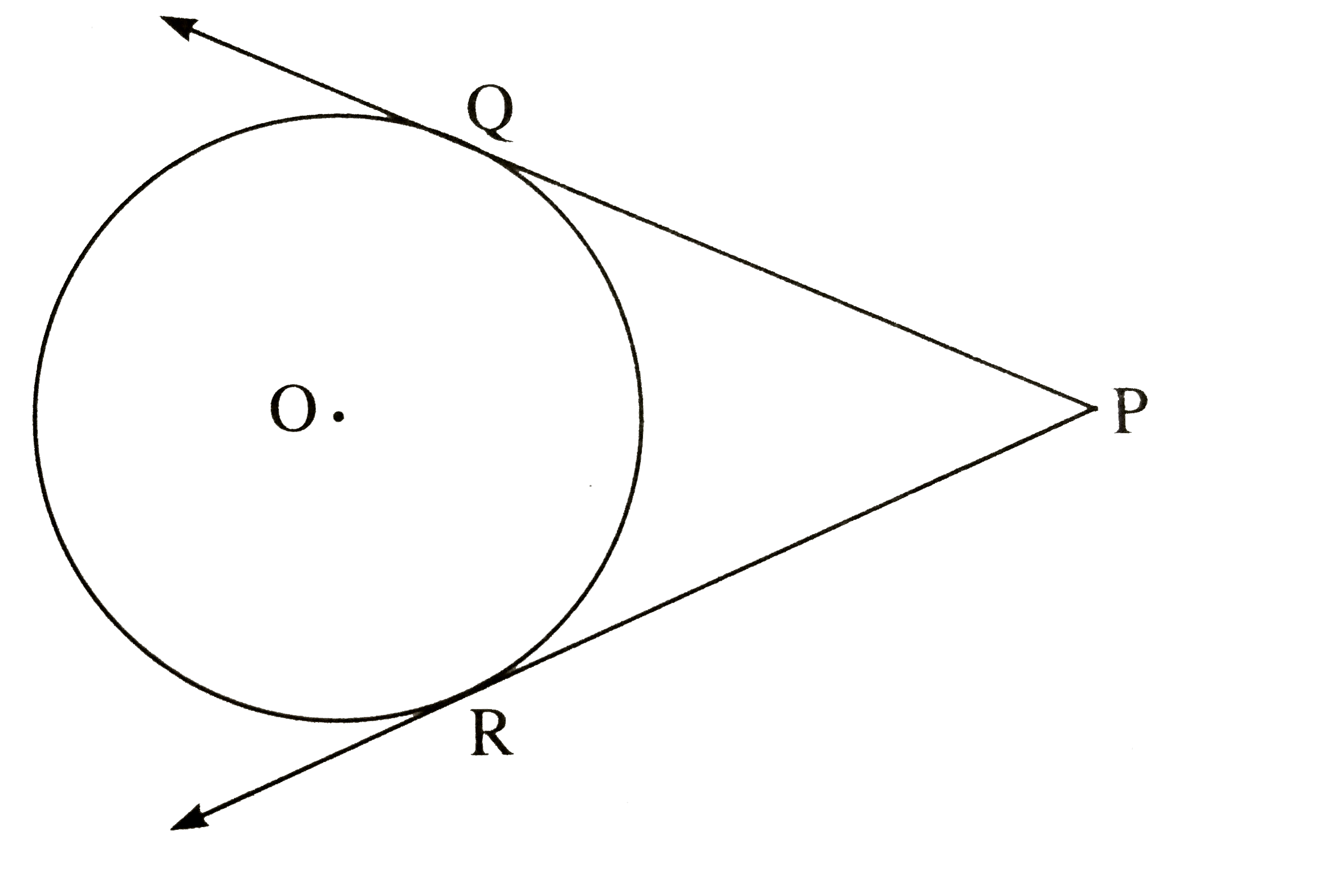
In the figure , PQ and PR are tangents drawn from and external point P to the circle with centre O. Q and R are the points of contact. If PQ = 5 cm then what is the length of segment PR ? Why ?
View SolutionThe middle point of the segment of the straight line joining the points (p,q)and(q,−p) is (r2,s2). What is the length of the segment?
View Solution
Knowledge Check
The middle point of the segment of the straight line joining the points (p,q) and (q,-p) is (r/2 , s/2) . What is the length of the segment ?
A[(s2+r+2)1/2]/2B[(s2+r2)1/2]/4C(s2+r2)1/2Ds+rIf a perpendicular is drawn to a line segment MN at N and point P is marked on perpendicular, then
A¯¯¯¯¯¯¯MP⊥¯¯¯¯¯¯NPB¯¯¯¯¯¯¯¯MN∣∣¯¯¯¯¯¯NPC¯¯¯¯¯¯¯¯MN∣∣¯¯¯¯¯¯¯MPD¯¯¯¯¯¯¯¯MN⊥¯¯¯¯¯¯NPThe length of the diagonal BD of the parallelogram ABCD is 18 cm. If PandQ are the centroid of the ΔABCandΔADC respectively then the length of the line segment PQ is
A4 cmB6 cmC9 cmD12 cm
Let E be the ellipse x216+y29=1. For For any three distinct points P,QandQ' on E, let M(P,Q) be the mid-point of the line segment joining P and Q, and M(P,Q') be the mid-point of the line segment joining P and Q'. Then the maximum possible value of the distance between M(P,Q)andM(P,Q'), as P,QandQ' vary on E, is ___ .
View SolutionIf the mid-point of the line segment between the axes of a line is (p,q) then find the equation of the line.
View SolutionDraw a line segment of length 6 cm. Find a point P on it which divides it in the ratio 3 : 4.
View SolutionDraw a line segment of length 7 cm. Find a point P on it which divides it in the ratio 3:5.
View SolutionMark against the correct answer:
In the adjoining figure, the point P lies
View Solution
