Express sin320∘ in terms of θR.
Video Solution
Text Solution
θR=360∘−320∘=40∘

Since the sine is negative in quadrant IV, sin320∘=−sin40∘
|
Updated on:21/07/2023
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
If sin37∘=z, express sin74∘ in terms of z.
A2z√1−z2B2z2+1C2zD2z2−1 - Question 2 - Select One
Express cos320∘ as a function of an angle between 0∘and90∘
I. cos40∘
II. sin50∘
III. cos50∘AI onlyBII onlyCIII onlyDI and II
cos20θ in terms of sin5θ.
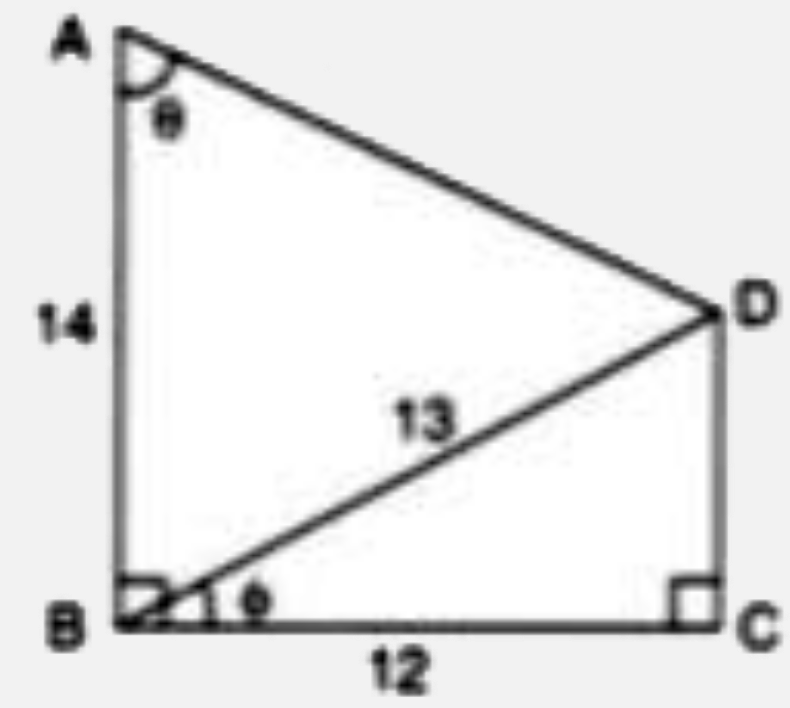
View SolutionUsing the measurements given in the following figure :
Write an expression for AD in terms of θ
 View Solution
View SolutionExpress sin5π2 in terms of cos5π,
View SolutionFor any θε(π4,π2) the expression 3(sinθ−cosθ)4+6(sinθ+cosθ)2+4sin6θ equals:
View SolutionThe expression nsin2θ+2ncos(θ+α) sinαsinθ+cos2(α+θ) is independent of θ , the value of n is
View Solutionsin100 in terms of functions of 5θ,
View Solution

