Express cos130∘ in terms of θR.
To express cos130∘ in terms of the reference angle θR, we can follow these steps:
Step 1: Identify the Quadrant
The angle 130∘ lies in the second quadrant, where cosine values are negative.
Step 2: Calculate the Reference Angle
The reference angle θR is calculated by subtracting the given angle from 180∘:
θR=180∘−130∘
θR=50∘
Step 3: Express Cosine in Terms of the Reference Angle
Since cos is negative in the second quadrant, we can express cos130∘ in terms of the reference angle:
cos130∘=−cosθR
Substituting θR:
cos130∘=−cos50∘
Final Expression
Thus, we can express cos130∘ in terms of the reference angle θR:
cos130∘=−cosθR
---
The angle 130∘ lies in the second quadrant, where cosine values are negative.
The reference angle θR is calculated by subtracting the given angle from 180∘:
θR=180∘−130∘
θR=50∘
Since cos is negative in the second quadrant, we can express cos130∘ in terms of the reference angle:
cos130∘=−cosθR
Substituting θR:
cos130∘=−cos50∘
Thus, we can express cos130∘ in terms of the reference angle θR:
cos130∘=−cosθR
Topper's Solved these Questions
Similar Questions
Express cot200∘ in terms of θR.
View SolutionExpress sin320∘ in terms of θR.
View SolutionExpress cotA in terms of cos2A
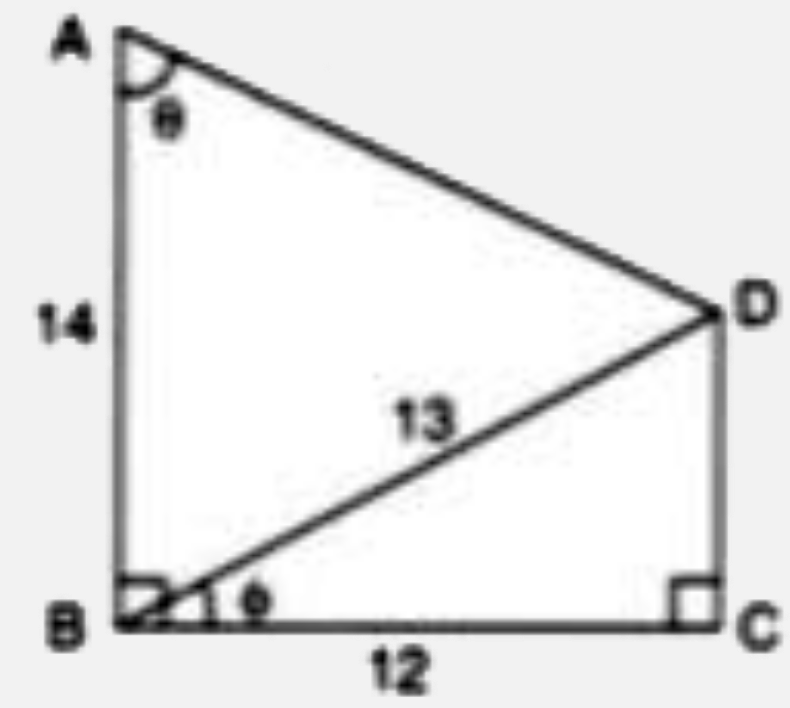
View SolutionUsing the measurements given in the following figure :
Write an expression for AD in terms of θ
 View Solution
View Solutioncos20θ in terms of sin5θ.
View SolutionRepresent cos6θ in terms of cosθ.
View SolutionWrite cos 4θ in terms of cosθ.
View Solution.Express cos6α in terms of cos3α.
View SolutionExpress cos6α in terms of cos3α.
View Solutioncos2θ in terms of cos4θ,
View Solution

