A capacitor of capacity C0 is conneted to a battery of emfV0 When steady state is attained a dielectric slab of dielectric constant K is slowly introduced in the capacitor to fill the capacitor completely. Mark the correct statement(s), in final stady state.
Magnitude of induced charge on the each surface of slab is C0V0(K−1)
Electric force due to induced charges on any plate is zero.
Force of attraction between plates of capacitor is K(C0V0)22∈0A
Field due to induced charges in dielectric slab is (K−1)C0V0∈0A
The correct Answer is:A, B, D
Charged induced on the surface of dielectric slab
=kC0V0(1−1k)=(k−1)C0V0
(B)−(k−1)C0V0+(k−1)C0V0
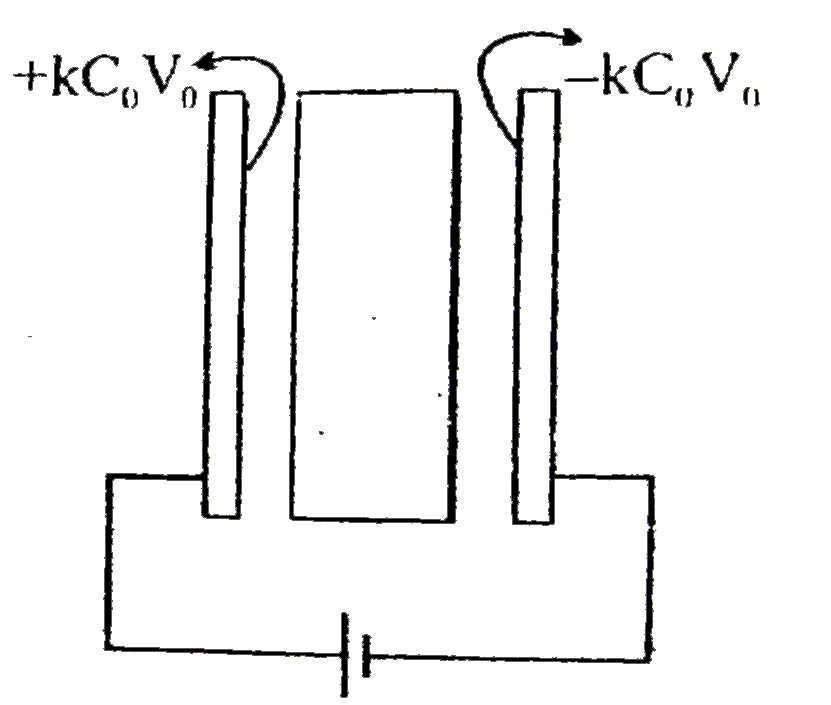
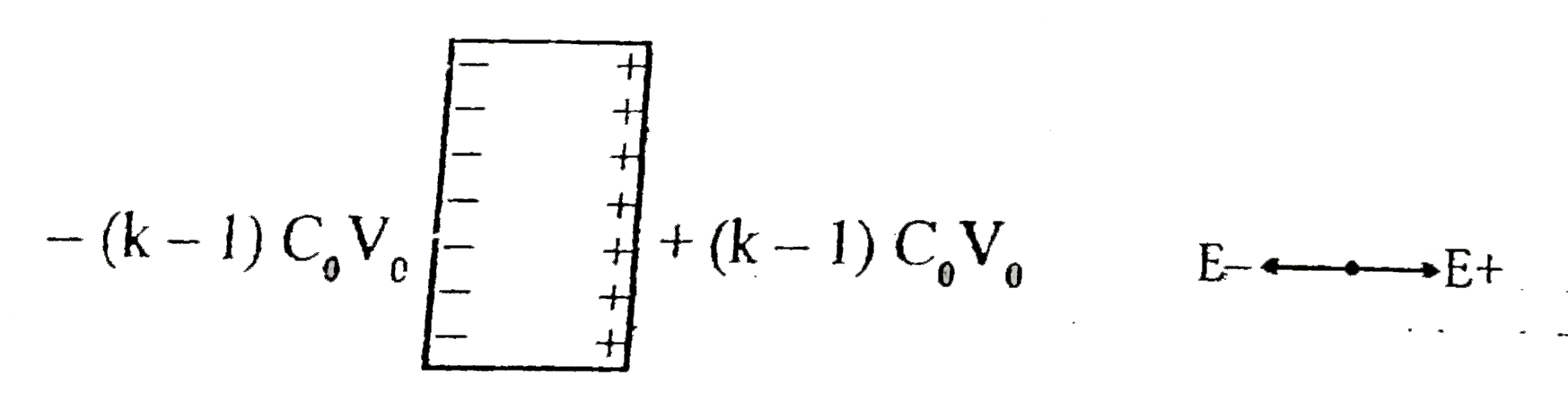
Resultant field is zero due to charge on both the surfaces.
(C) Force of attraction =(kC0V02∈0A(kC0V0)=(k2C0V02∈0A
(D) (k−1)C0V0∈0A
Topper's Solved these Questions
- BOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Subjective107videos
MASTER PRACTICE PROBLEM
- BOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Additional topic70videos
MASTER PRACTICE PROBLEM
- BOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Reasoning75videos
MASTER PRACTICE PROBLEM
- BOOK - BANSALCHAPTER - FLUID MECHANICSEXERCISE - PYQS AIEEE10videos
FLUID MECHANICS
- BOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question32videos
SEMICONDUCTORS
Similar Questions
A capacitor of capacitance 'C' is connected with a cell of 'E' e.m.f. When it is fully charged, a dielectric medium of dielectric constant 'k' is introduced in it find it's final charge if cell is kept connected with it
View SolutionA capacitor of capacitance 200μF is connected to a 200V battery. Dielectric material of dielectric constant 2 is introduce in between the plates of the capacitor. Find change in energy stored in the process.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A capacitor is connected to a battery of voltage V. Now a di-electric slab of di-electric constant k is completely inserted between the plates, then the final charge on the capacitor will be : (If initial charge is q0)
Aε0AdVBkε0AdVCε0AkdADzero - Question 2 - Select One
A parallel plate capacitor has a capacity c. If a medium of dielectric constant K is introduced between plates, the capacity of capacitor becomes
ACKBCK2CK2CDKC - Question 3 - Select One
A parallel plate capacitor having capacitance C farad is connected with a battery of emf V volts. Keeping the capacitor cannected with the battery, a dielectric slab of dielectric constant K is inserted between the plates. The dimensions of the slab are such that it fills the space between the capacitor plates. Then,
Acharge the capacitor plates remains the sameBcharge on the plates increases K timesCpotential difference between the plates decreases to V/KDall of these
A parallel plate capacitor of capacity 5μF and plate separation 6cm is connected to a 1V battery and is charged. A dielectric of dielectric constant 4 and thickness 4cm is introduced into the capacitor. The additional charge that flows into the capacitor from the battery is.
View SolutionA parallel capacitor of capacitance C is charged and disconnected from the battery. The energy stored in it is E. If a dielectric slab of dielectric constant 6 is inserted between the plates of the capacitor then energy and capacitance will become.
View SolutionA parallel plate capacitor of capacitance C0 is charged with a charge Q0 to a potential difference V0 and the battery is then disconnected Now a dielectric slab of dielectric constant k is inserted between the plates ofcapacitor. The dimensions of the slab are such that it completely fills the space between the plates, then :
View SolutionA parallel plate capacitor of capacity 5μF and plate separation 6cm is connected to a 1V battery and is charged. A dielectric of dielectric constant 4 and thickness 4cm is introduced into the capacitor. The additional charge that flows into the capacitor from the battery is.
View SolutionThe plates of a capacitor are connected to a source of emf and the energy stored is U0. When dielectric material dielectric constant K is introduced between the plates filling the whole space, the energy stored becomes U Now.
View Solution

