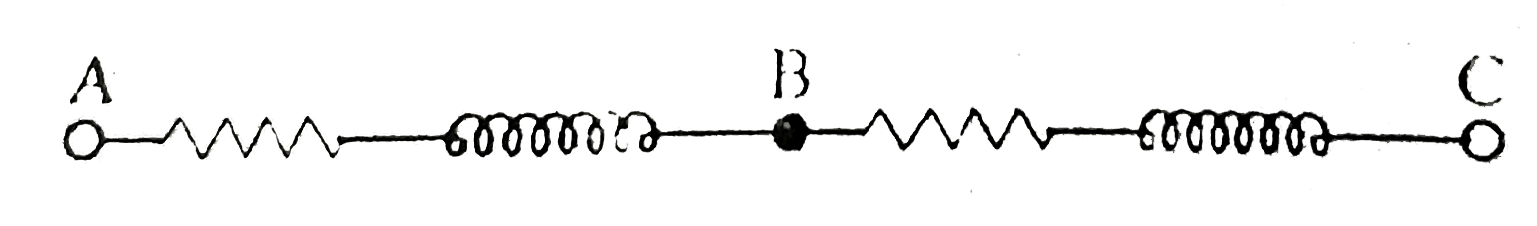
If an AC voltage 100 V is applied between points A and B, then current of 1 A and phase difference between current and voltage will be Δϕ=37∘ . IF the same voltage is applied between points B and C, then current of 5 A and Δϕ=53∘ What will be the impedance (∈Ω) in the chain, if the same voltage is applied between points A and C? Round off to nearest integer.
Video Solution
Text Solution
The correct Answer is:0119
tanϕ=wL1R1=34
wL1=34R1
1=100√916R21+R21
R1=80ΩωL1=60Ω
wL2R2=43⇒wL2=43R2
5=100√169R22+R22⇒R2=12Ω,WL2=16Ω
z=√(wL1+wL2)2+(R1+R2)2=119Ω
|
Topper's Solved these Questions
- MASTER PRACTICE PROBLEMBook:BANSALChapter:MASTER PRACTICE PROBLEMExercise:Additional topic
Explore 70 Videos
- MASTER PRACTICE PROBLEMBook:BANSALChapter:MASTER PRACTICE PROBLEMExercise:Multiple objective
Explore 131 Videos
- FLUID MECHANICSBook:BANSALChapter:FLUID MECHANICSExercise:PYQS AIEEE
Explore 10 Videos
- SEMICONDUCTORSBook:BANSALChapter:SEMICONDUCTORSExercise:CBSE Question
Explore 32 Videos
Similar Questions
Knowledge Check
In an AC circuit XC=XL. The phase differe3nce between the current and voltage will be
A0BπCπ/2Dπ/4An Ac voltage V=V0sin100t is applied to the circuit, the phase difference between current and voltage is found to be π4, then
 .AR=100Ω,C=1μFBR=1Ω,C=10μFCR=410kΩ,L=1HDR=1kΩ,L=10H
.AR=100Ω,C=1μFBR=1Ω,C=10μFCR=410kΩ,L=1HDR=1kΩ,L=10HThe phase difference between the current and voltage of a LCR series a.c. circuit at resonance is
A0Bπ2Cπ4Dπ
Find the phase difference between voltage and current.
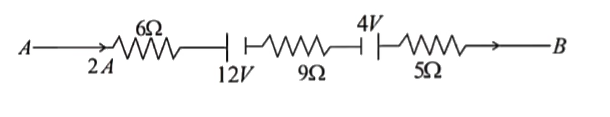
View SolutionThe voltage drop between the points A and B in the given figure is
View SolutionIn an a.c. circuit average power consumed depends on the phase difference between current and voltage in the circuit.
View SolutionIn an AC circuit voltage applied is e=220 sin 100 t. if the impedance is 110Ω and phase difference between the current and voltage is 60∘ the power consumption is equal to
View SolutionThe phase difference between the current and voltage of LCR circuit in series combination at resonance is
View Solution
