A block B is floating in the river. The position of the block is as shown in figure at time t=0. Flow speed of river is 2m/s. A stone is thrown from point O at time t=0 with a velocity →V=(v1ˆj+v2ˆj+20)ˆkm/s.
(a) Find v1, v2 such that the stone hits the block.
(b) If the speed of throw is kept fixed, what is the maximum value of time t at which it is possible to throw the stone to bit the block.
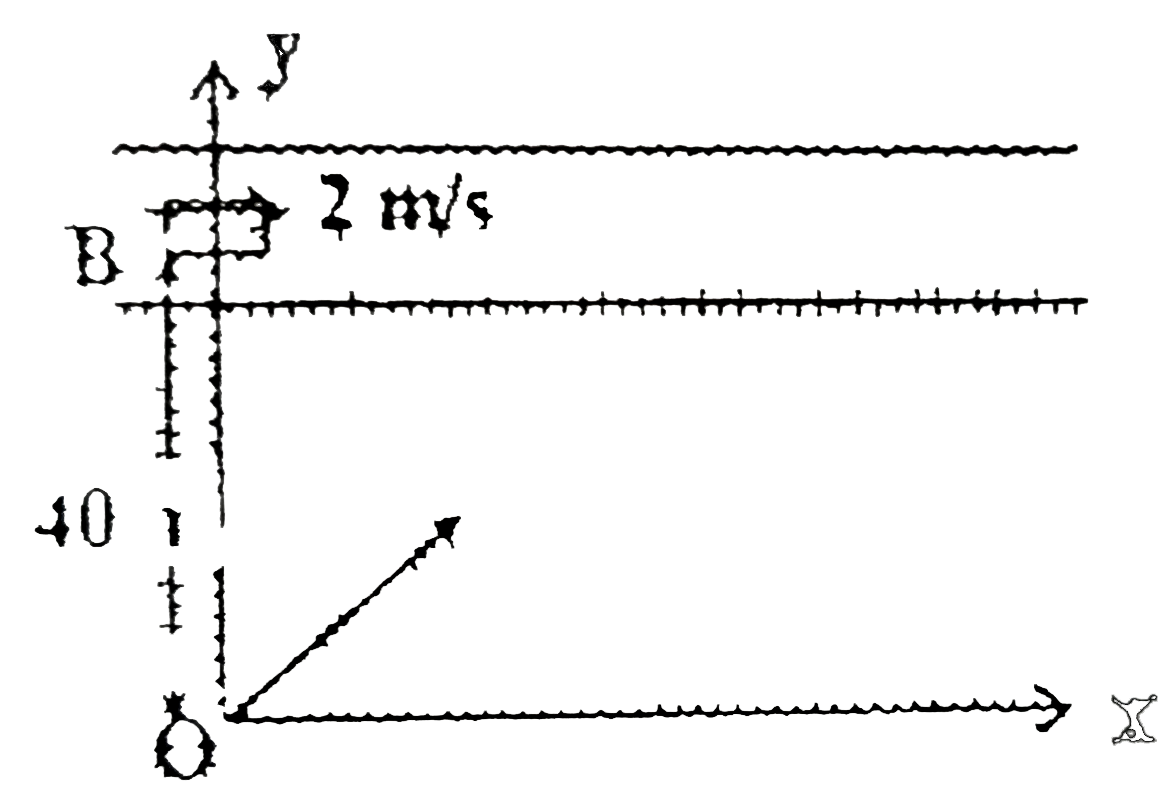
(a) Position vectors of the block and the stone are
→rB=(2t)ˆi+(40)ˆj
→rS=v1tˆi+v2tˆj+(20t−12gt2)ˆk
When the stone hits the block
2t=v1t , 40m=v2t , 20t−12gt2=0
v1=2m/s , v2=40tm/s , t=4s
⇒v2=10m/s.
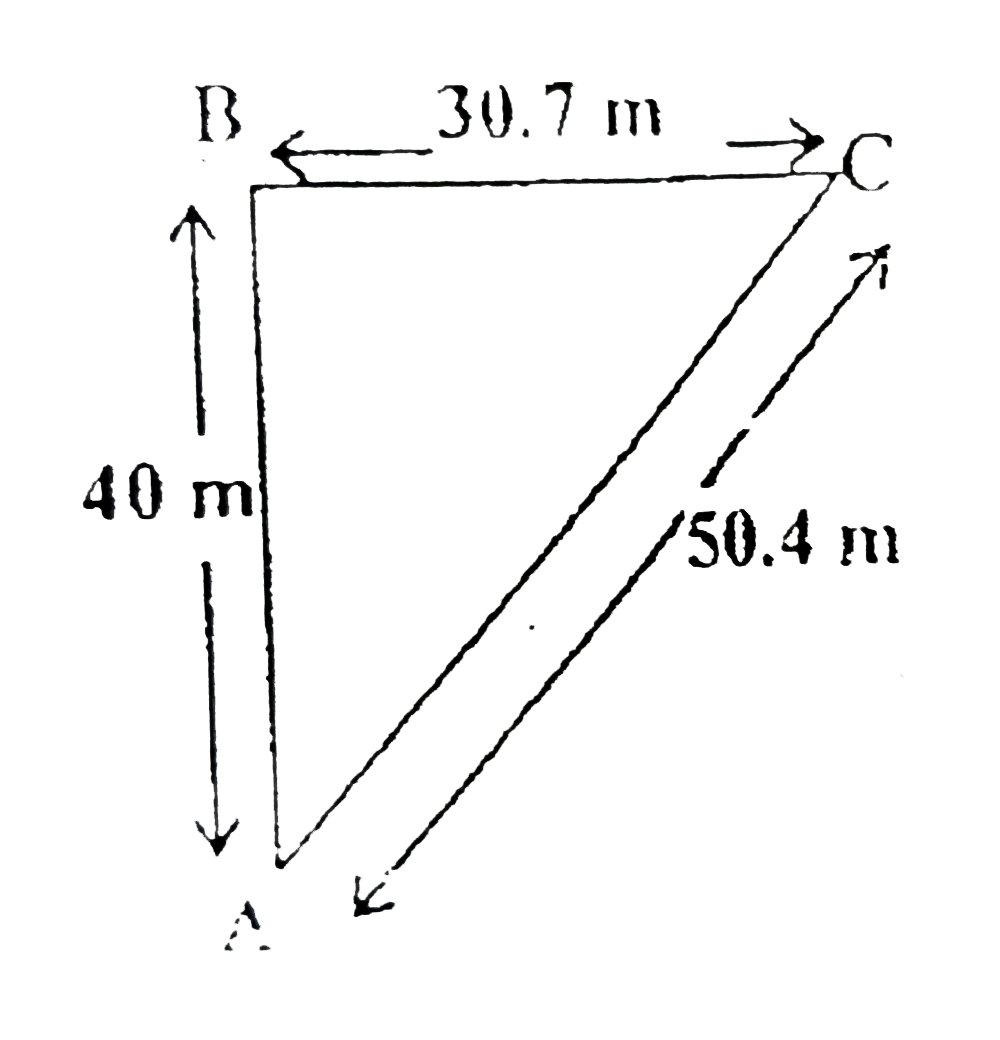
(b) For the same speed of throw, the maximum range
Rmax=v2g=v21+v22+202g=50410=50.4m
⇒BC=30.7m.
Time taken by the stone to reach C=30.72=15.35s
Time of fligh' of the stone =2(v/√2)g=√2√50410=3.17s
Maximum value of t=15.35−3.17=12.18s.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
A block of the mass of 1 kg is moving on the x -axis. A force F acting on the block is shown. The veloity of the block at time t = 2 s is −3ms−1. What is the speed of the block at time t = 4 s?
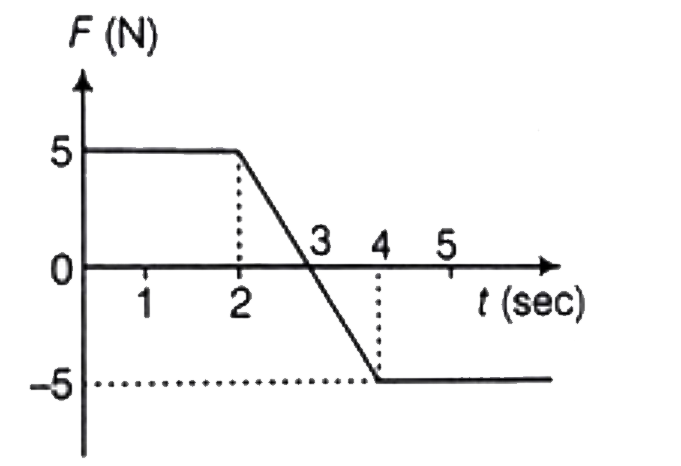 A8ms−1B2ms−1C3ms−1D5ms−1
A8ms−1B2ms−1C3ms−1D5ms−1 - Question 2 - Select One
A block of mass m in floating in a river flowing with a velocity of 2m/s.A boat is moving behind the block with a velocity of 5m/s with respect to the block as shown.From the boat a stone is thrown with a velocity →v=v1ˆi−vˆj+v3ˆk with respect to the river such that it hits the block.If v1:v2:v3=2√3:2:3 then the velocity of the stone with respect to the ground is (g=10m/s2)
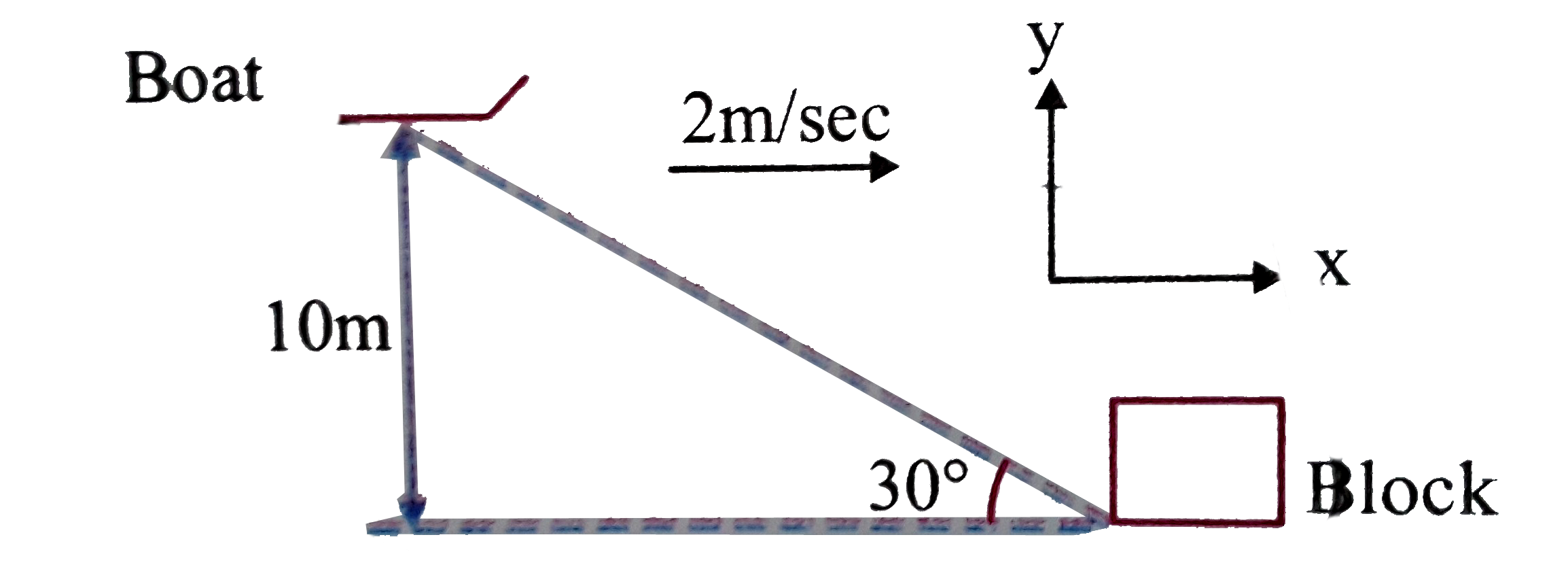 A10ˆi−10√3ˆj+5√3ˆkB12ˆi−10√3ˆj+5√3ˆkC10ˆi−10ˆj+5√3ˆkD10√3ˆi−10√3ˆj+5√3ˆk
A10ˆi−10√3ˆj+5√3ˆkB12ˆi−10√3ˆj+5√3ˆkC10ˆi−10ˆj+5√3ˆkD10√3ˆi−10√3ˆj+5√3ˆk - Question 3 - Select One
A cubical block of side a is moving with velocity v on a horizontal smooth plane as shown. It hits a ridge at point O. The angular speed of the block after it hits O is:
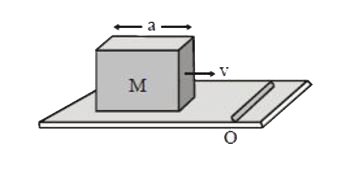 A3v//4aB3v//2aC√3v√2aDZero
A3v//4aB3v//2aC√3v√2aDZero
A cubical block of side a is moving with velocity v on a horizontal smooth plane as shown. It hits a ridge at point O. The angular speed of the block after it hits O is:
 View Solution
View SolutionTwo particle are moving with velocity →v1=ˆi−2tˆj+ˆkm/s and →v2=4tˆi+tˆj+3ˆkm/s respectively. Time at which they are moving perpendicular to each other is ____________ (sec). (Round off to nearest integer).
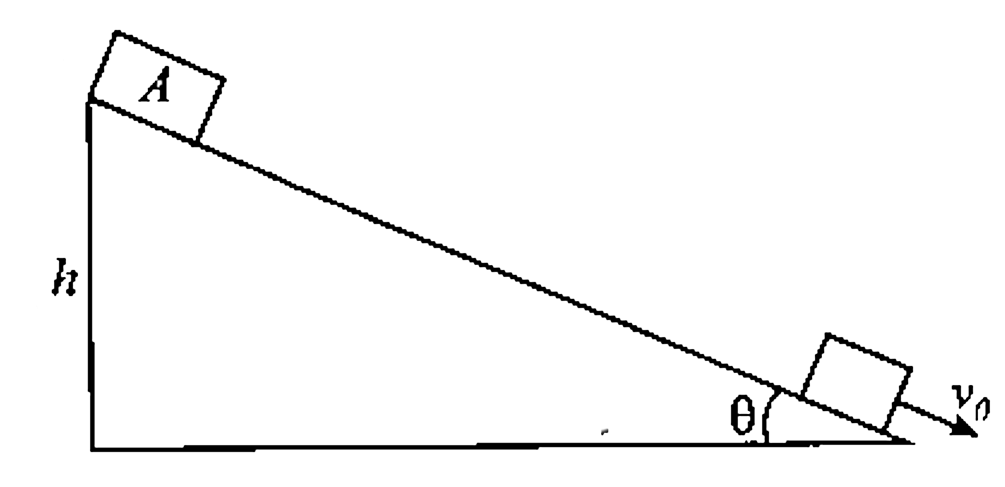
View SolutionA block of A is released from rest from the top of wedge block of height h shown in figure-4.55. If velocity of block when it reaches the bottom of inclive is v0, find the time os sliding.
 View Solution
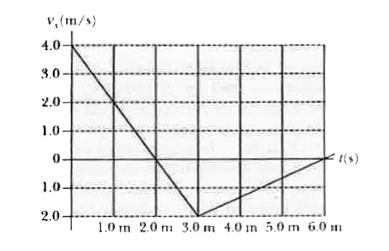
View Solutionvx is the velocity of a particle moving along the x-axis as shown in the figure. If x=2.0m at t=1.0s, what is the position of the particle at t=6.0s ?
 View Solution
View SolutionIn the arrangement shown in figure the ratio of velocity V1&V2 of block (1)&(2) is
 View Solution
View Solution

