Doubtnut Promotions Sticky

Which of the following statements can be possible cases in one//two dimensional motion ?

A

A body has zero velocity and is still accelerating.

B

The velocity of an object reverses direction when acceleration is constant.

C

An object's speed goes on increasing even magnitudes of its acceleration decreases.

D

None of these

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

(A) When the body is projected vertically up, at the highest point its speed becomes zero whereas it is accelerating downwards withg=9.8m/s2.
(B) When a body is projected up, the velocity at the highest point reverses its direction whereas the acceleration of the body remains constant, that is vevg.
(C) Acceleration means, increasing speed (magnitude of velocity). Therefore, the speed of a particel increases with an acceleration. When the acceleration is decreased the speed of the particle goes on increasing till the acceleration reduces to zero.

|
Updated on:21/07/2023
Class 11PHYSICSRECTILINEAR MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    Which statements can be possible cases in one/two dimensional motion:

    AA body has zero velocity and still be accelerating
    BThe velocity of an object reverses direction when acceleration is constant
    CAn object be increasing in speed as its acceleration decreases
    DNone of these
  • Question 2 - Select One

    Which of the following graphs cannot possibly represent one dimensional motion of a particle?
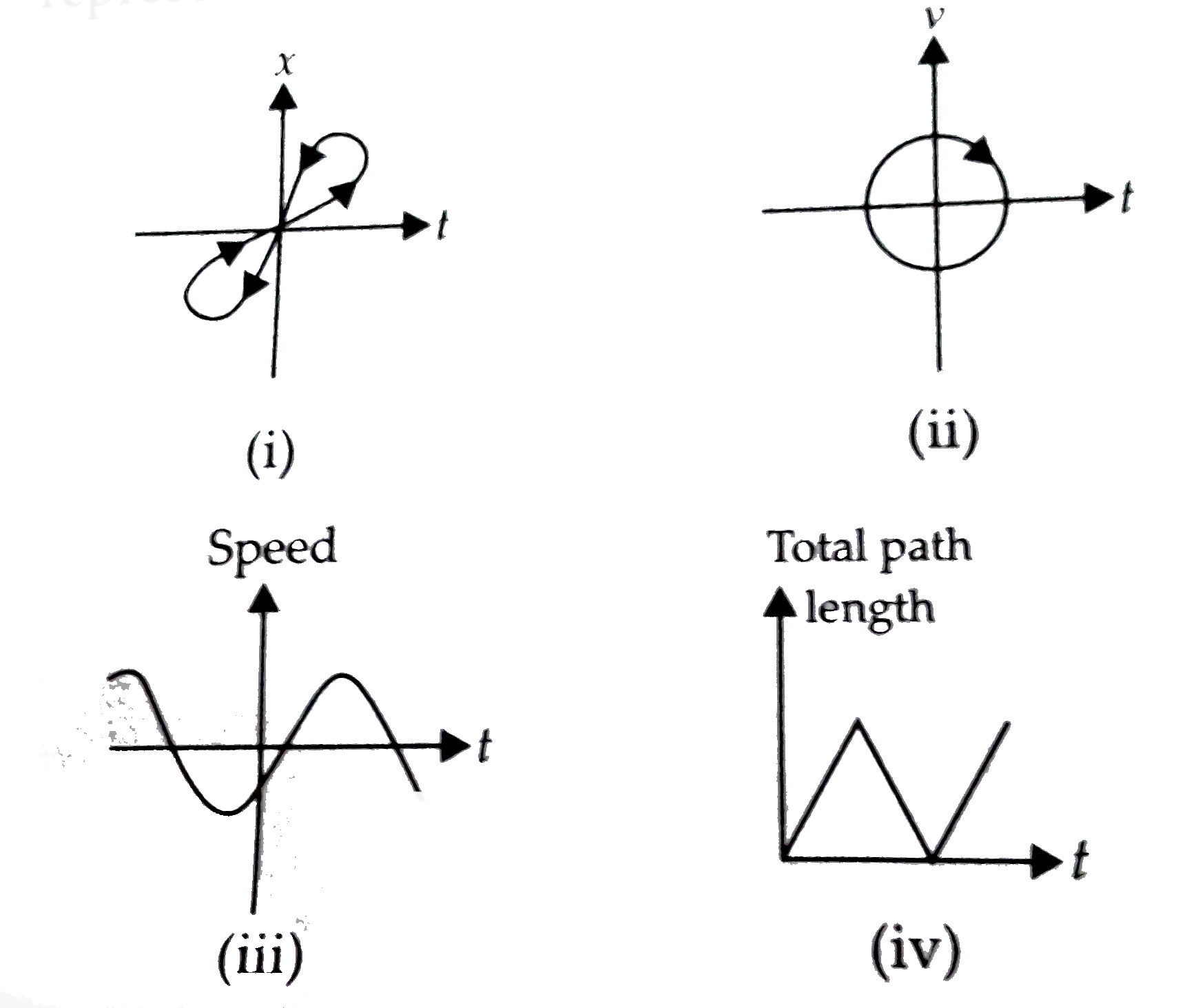    ocr_image

    A(i) and (ii)
    B(ii) and (iii)
    C(i), (ii) and (iii)
    DAll four
  • Question 3 - Select One

    Which one of the following statements is correct? In the case of

    Ashearing stress there is charge in volume.
    B hydraulic stress there is no change in shape.
    Cshearing stress there is no change in shape.
    D hydraulic stress there is no change in volume.