Pressure below the free surface of a liquid is in excess of atmosphere pressure. Datermine its density and specific gravity.
The correct Answer is:
To solve the problem step by step, we will follow these instructions:
Given:
- Pressure at a depth of 3 m below the free surface of a liquid, P=15kN/m2 (which is equal to 15×103N/m2)
- Acceleration due to gravity, g=10m/s2
- Depth, h=3m
Step 1: Understand the relationship between pressure, density, gravity, and height.
The pressure at a certain depth in a fluid can be expressed by the formula:
P=P0+ρgh
Where:
- P is the pressure at depth,
- P0 is the atmospheric pressure (which we can consider as 0 for this problem since we are given excess pressure),
- ρ is the density of the liquid,
- g is the acceleration due to gravity,
- h is the depth below the surface.
Step 2: Substitute the known values into the equation.
Since we are given that P0=0 (considering excess pressure), we can simplify the equation:
P=ρgh
Substituting the known values:
15×103=ρ×10×3
Step 3: Solve for the density ρ.
Rearranging the equation to solve for ρ:
ρ=15×10310×3
Calculating the right-hand side:
ρ=15×10330=15,00030=500kg/m3
Step 4: Calculate the specific gravity.
Specific gravity (SG) is defined as the ratio of the density of the liquid to the density of water. The density of water is approximately 1000kg/m3.
SG=ρDensity of water=5001000=0.5
Final Answers:
- Density of the liquid, ρ=500kg/m3
- Specific gravity, SG=0.5
- Pressure at a depth of 3 m below the free surface of a liquid, P=15kN/m2 (which is equal to 15×103N/m2)
- Acceleration due to gravity, g=10m/s2
- Depth, h=3m
The pressure at a certain depth in a fluid can be expressed by the formula:
P=P0+ρgh
Where:
- P is the pressure at depth,
- P0 is the atmospheric pressure (which we can consider as 0 for this problem since we are given excess pressure),
- ρ is the density of the liquid,
- g is the acceleration due to gravity,
- h is the depth below the surface.
Since we are given that P0=0 (considering excess pressure), we can simplify the equation:
P=ρgh
Substituting the known values:
15×103=ρ×10×3
Rearranging the equation to solve for ρ:
ρ=15×10310×3
Calculating the right-hand side:
ρ=15×10330=15,00030=500kg/m3
Specific gravity (SG) is defined as the ratio of the density of the liquid to the density of water. The density of water is approximately 1000kg/m3.
SG=ρDensity of water=5001000=0.5
- Density of the liquid, ρ=500kg/m3
- Specific gravity, SG=0.5
Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - FLUID MECHANICSEXERCISE - Exercise- 1 PART - I Section (B)3videos
FLUID MECHANICS
- BOOK - RESONANCECHAPTER - FLUID MECHANICSEXERCISE - Exercise- 1 PART - I Section (C)6videos
FLUID MECHANICS
- BOOK - RESONANCECHAPTER - FLUID MECHANICSEXERCISE - Board Level Exercise18videos
FLUID MECHANICS
- BOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - Exercise52videos
ELECTROSTATICS
- BOOK - RESONANCECHAPTER - FULL TEST 1EXERCISE - Exercise30videos
FULL TEST 1
Similar Questions
There is an air bubble of radius 2.0 mm in a liquid of surface tension 0.070Nm−1 and density 103kgm−3 The bubble is at a depth of 12.0 cm below the free surface of liquid. By what amount is the pressure inside the bubble is greater then the atmospheric pressure ? Use g=10m/s2.
View SolutionAn air bubble of radius 2 mm is at a depth of 5 cm below the free surface of a liquid of surface tension 0.06 N/m and density 1000 kg/m3. The pressure inside the bubble is greater than the atmospheric pressure by n×140N/m2. Find the value of n.
View Solution
Knowledge Check
- Question 1 - Select One
The pressure at depth h below the surface of a liquid of density ρ open to the atmosphere is
Agreater than the atmospheric pressure by ρghBless than the atmospheric pressure by ρghCequal to the atmospheric pressureDincreases exponentially with depth - Question 2 - Select One
The absolute pressure at a depth h below the surface of a liquid of density ρ is [Given Pa = atmospheric pressure, g = acceleration due to gravity]
AρghBPa+ρghCPa−ρghDPa2ρgh - Question 3 - Select One
What is the pressure energy of a liquid of mass m and density ρ?
APmρBPρCmρDPmρ
The U-tube acts as a water siphon. The bend in the tube is 1m above the water surface. The tube outlet is 7m below the water surface. The water issues from the bottom of the siphon as a free jet at atmospheric pressure. Determine the speed of the free jet and the minimum absolute pressure of the water in the bend. Given atmospheric pressure =1.01×105N/m2,g=9.8m/s2 and density of water =103kg/m3
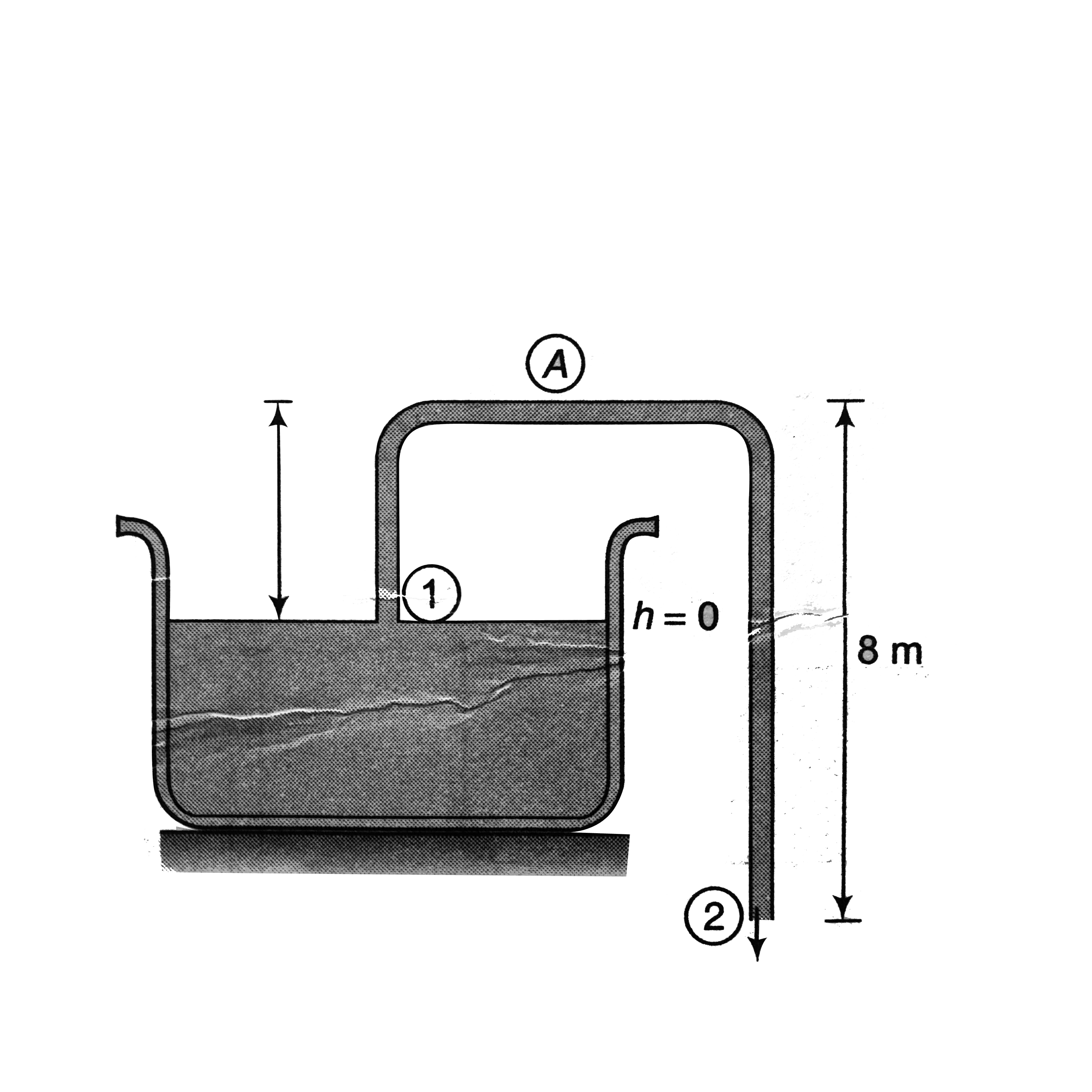 .View Solution
.View SolutionWhat is the pressure on a swimmer 10m below the surface of lake? g=10ms−2, atmospheric pressure = 1.01×105Pa
View SolutionWhat will be the total pressure inside a spherical air bubble of radius 0.2 mm at a depth of 2m below the surface of a liquid of density 1.1g/cm3 and surface tension 50dyne/cm. Atmospheric pressure is 1.01×105Nm−2.
View SolutionIf pressure at the half depth of a lake is equal to 34 times the pressure at its bottom, then find the depth of the lake. [Take g = 10 m/s2]
View SolutionAt a depth of 5 m below the free surface of a liquid, an air bubble of radius 2 mm is formed. The pressure inside the bubble will be (The surface tension of water is 20×10−8 N/m.)
View Solution

