Question

A pair of stars rotates about a common centre of mass. One of the stars has a mass M which is twice as large as the mass m of the other. Their centres are a distance apart, d being compared to the size of either star.
(a) Derive an expression for the period of rotation of the stars about their common centre of mass in terms of d, m, G.
(b) Compare the angular momentum of the two stars about their common centre of mass by calculating the ratio $L_m/L_M$.
(c ) Compare the kinetic energies of the two stars by calculating th eratio $K_m/K_M$.

Answer

(a) `T=(2pi d^(3//2))/(sqrt(3Gm))`, (b) 2 (c ) 2

Explanation

They will rotate about their c.m. position of centre of mass
`0=(2m(-x)+m(d-x))/(2m+m)`
`x=(d)/(3)`

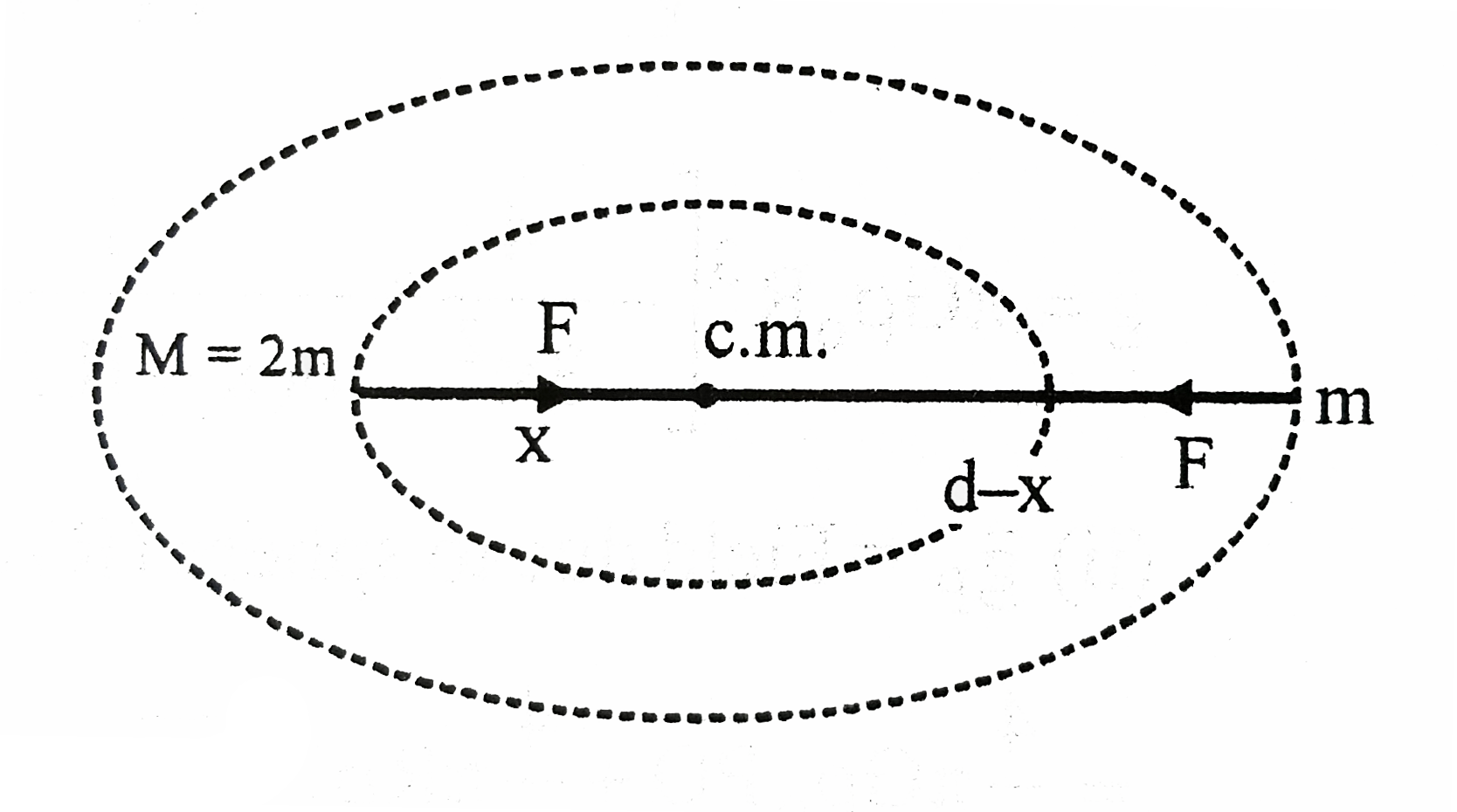
(a) These force of atraction will provide required centripetal force
`((2m)v_(M)^(2))/(d//3)=(G2m.m)/(d^(2)) rArr v_(M)=sqrt((1)/(3)(GM)/(d))`
`T = (2pi(d//3))/(v_(M)) rArr T = (2pi d)/(3). sqrt((3d)/(GM))`
`T = 2pi sqrt((d^(3))/(3GM)) rArr T = (2pi d^(3//2))/(sqrt(3Gm))`