Two spherical planets P and Q have the same uniform density $ρ,$ masses $M_{p} and M_{Q}$ and surface areas A and 4A respectively. A spherical planet R also has uniform density $ρ$ and its mass is $(M_{P} + M_{Q}) .$ The escape velocities from the plantes P,Q and R are $V_{P} V_{Q} and V_{R}$ respectively. Then

A

$V_{Q} > V_{R} > V_{P}$

B

$V_{R} > V_{Q} > V_{P}$

C

$V_{R} / V_{P} = 3$

D

$V_{P} / V_{Q} = \frac{1}{2}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B, D

$\begin{matrix} \frac{P}{ρ} & \frac{Q}{ρ} & \frac{R}{ρ} M & 8 M & 9 M A & 4 A R & 2 R & 3^{\frac{2}{3}} R \end{matrix}$
$V_{P} = \sqrt{\frac{G M}{R}}$
$V_{Q} = \sqrt{\frac{G 8 M}{2 R}} = 2 \sqrt{\frac{G M}{R}} = 8^{1 / 3} \sqrt{\frac{G M}{R}}$
$∴ \frac{V_{P}}{V_{Q}} = \frac{1}{2}$
$V_{R} = \sqrt{\frac{G (9 M)}{3^{2 / 3} R}} = \sqrt{3^{4 / 3} \frac{G M}{R}} = 3^{\frac{2}{3}} \sqrt{\frac{G M}{R}} = 9^{1 / 3} \sqrt{\frac{G M}{R}}$
$∴ V_{R} > V_{Q} > V_{P}$

|

Updated on:21/07/2023

Class 11 PHYSICS GRAVITATION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The escape velocities of the two planets, of densities $ρ_{1}$ and $ρ_{2}$ and having same radius, are $v_{1}$ and $v_{2}$ respectively. Then
A $\frac{v_{1}}{v_{2}} = \frac{ρ_{1}}{ρ_{2}}$
B $\frac{v_{2}}{v_{1}} = \frac{ρ_{2}}{ρ_{1}}$
C $\frac{v_{1}}{v_{2}} = {(\frac{ρ_{1}}{ρ_{2}})}^{2}$
D $\frac{v_{1}}{v_{2}} = \sqrt{\frac{ρ_{1}}{ρ_{2}}}$
Question 2 - Select One or More
The masses of two spherical planets A and B are $M_{A}$ and $M_{B}$ , surface areas are S and 9 S respectively. The mass of a spherical planet C is $(M_{A} + M_{B})$ . If the densities of all the planets A, B, C are same, then the escape velocities $V_{A}$ , $V_{B}$ , $V_{C}$ from the surface of these planets follow:
A $V_{C}$ gt $V_{B}$ gt $V_{A}$
B $V_{B}$ = 3 $V_{A}$
C $V_{C}$ lt $V_{B}$ lt $V_{A}$
D $V_{C}$ gt $V_{B}$ lt $V_{A}$
Question 3 - Select One
Three particales $P, Q$ and $R$ are placedd as per given Masses of $P, Q$ and $R$ are $\sqrt{3} m \sqrt{3} m$ and m respectively The gravitational force on a fourth particle 'S' of mass m is equal to
.
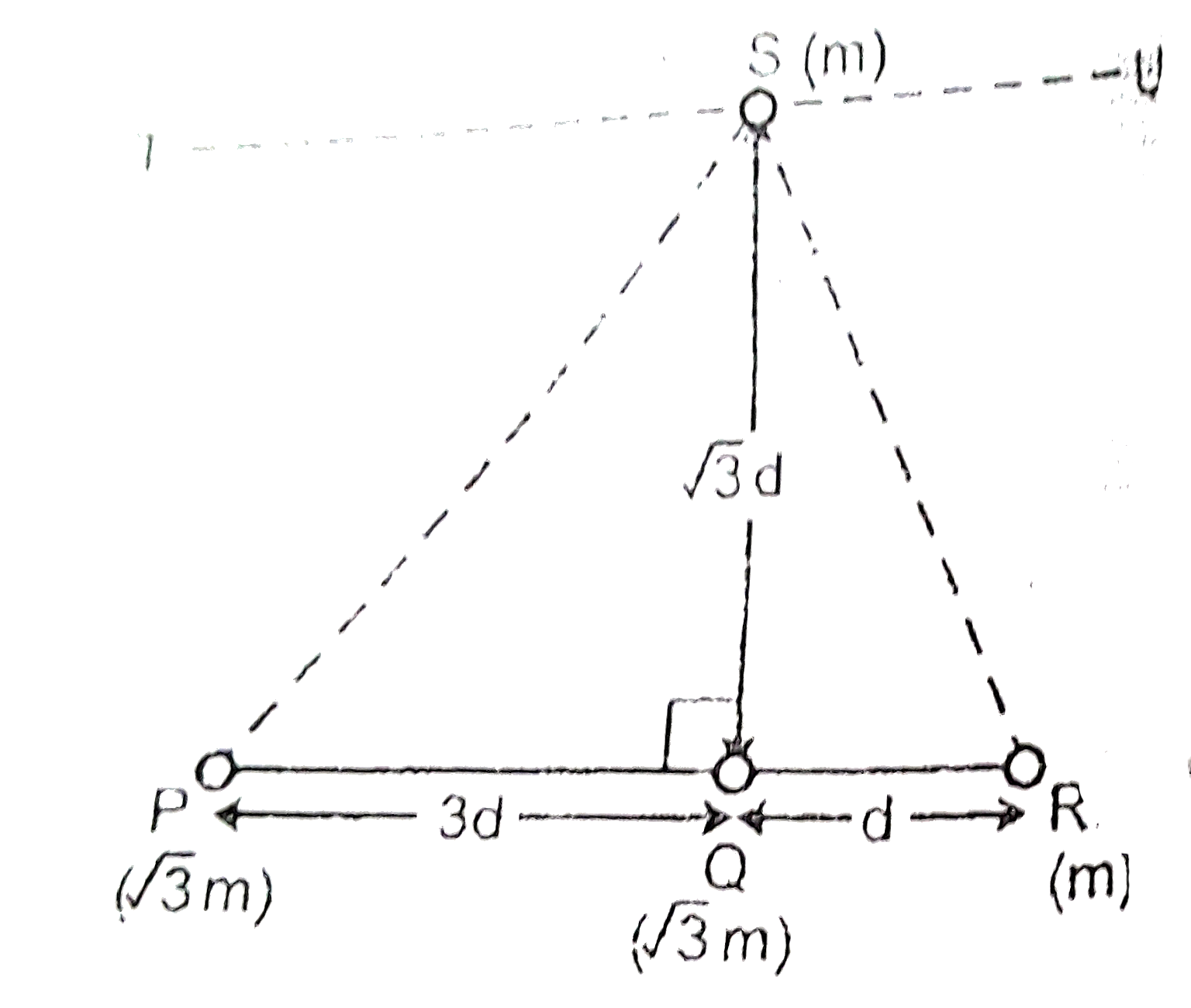A $\frac{\sqrt{3} G M^{2}}{2 d^{2}}$ in ST direction only
B $\frac{\sqrt{3} G M^{2}}{2 d^{2}}$ in SQ direction and $\frac{\sqrt{3} G M^{2}}{2 d^{2}}$ in SU direction
C $\frac{\sqrt{3} G M^{2}}{2 d^{2}}$ in SQ directiononly
D $\frac{\sqrt{3} G M^{2}}{2 d^{2}}$ in SQ direction and $\frac{\sqrt{3} G M^{2}}{2 d^{2}}$ in ST direction .

BANSAL-GRAVITATION-EXERCISE -4 Section - A

Home
Ask Doubt
Profile