Find the equation of trajectory of a particle whose velocity components are
vx=2x+1,vy=2y+3
Given that particle starts from rest from origin.
vx=2x+1,
dxdt=2x+1
∫x0dx2x+1=∫t0dt
12ln(2x+1)=t ....(1)
vy=2y+3
∫y0dy2y+3=∫t0dt
12ln (2y+3)3=t ...(2)
from (1) and (2)
12ln(2x+1)=12ln(2y+3)3
2x+1=(2y+3)3
y=3x
Topper's Solved these Questions
- UNIT DIMENSION, VECTOR & BASIC MATHSBook:BANSALChapter:UNIT DIMENSION, VECTOR & BASIC MATHSExercise:Solved Example
Explore 17 Videos
- UNIT DIMENSION, VECTOR & BASIC MATHSBook:BANSALChapter:UNIT DIMENSION, VECTOR & BASIC MATHSExercise:PRACTICE EXERCISE
Explore 4 Videos
- KINETIC THEORY OF GASESBook:BANSALChapter:KINETIC THEORY OF GASESExercise:Section-B
Explore 13 Videos
Similar Questions
In the figure a graph is plotted which shows the variation of the x- component of a force that acts on a particle moving along the x-axis, with x. The particle starts from rest from the origin. Then
View SolutionThe potential energy of a particle of mass 5 kg moving in the x−y plane is given by U=(−7x+24y)J, where x and y are given in metre. If the particle starts from rest, from the origin, then the speed of the particle at t=2s is
View Solution
Knowledge Check
Path traced by a moving particle in space is called trajectory of the particle. Shape of trajectiry is decided by the forces acting on the particle. When a coordinate system is associated with a particle motion, the curve equation in which the particle moves [y=f(x)] is called equation of trajectory. It is just giving us the relation among x and y coordinates of the particle i.e. the locus of particle. To find equation of trajectory of a particle, find first x and y coordinates of the particle as a function of time eliminate the time factor.
The position vector of car w.r.t. its starting point is given as →r=atˆi−bt2ˆj where a and b are positive constants. The locus of a particle is:-Aa2y+bx2=0Ba2y=bx2Cy=ba2Day2=b2xVelocity of a particle varies with time as v=atˆi+2ht2ˆj. If the particle starts from point (0,c), the trajectory of the particle is
Ay=bx3/2a+cBy=4√2b3(xa)3/2+cCy=4√2b3(xa)3/2−cDy=bx3/2a−cStarting from rest, the acceleration of a particle is a=2(t−1). The velocity (i.e.v=∫ a dt ) of the particle at t=10 s is :-
A15 m/sB25 m/sC5 m/sD80 m/s
A particle P is to be projected from a fixed point A on the ground with a fixed speed u. Another particle Q is also to be projected from a point B which is directly above the point A. The trajectory of the particle Q touches all possible trajectories (for different angle of projection) of the particle P in the vertical x−y plane. Find the equation of trajectory of the particle Q. (Take the point A as origin, horizontal axis x− axis and vertical axis y−axis Consider the figure.)
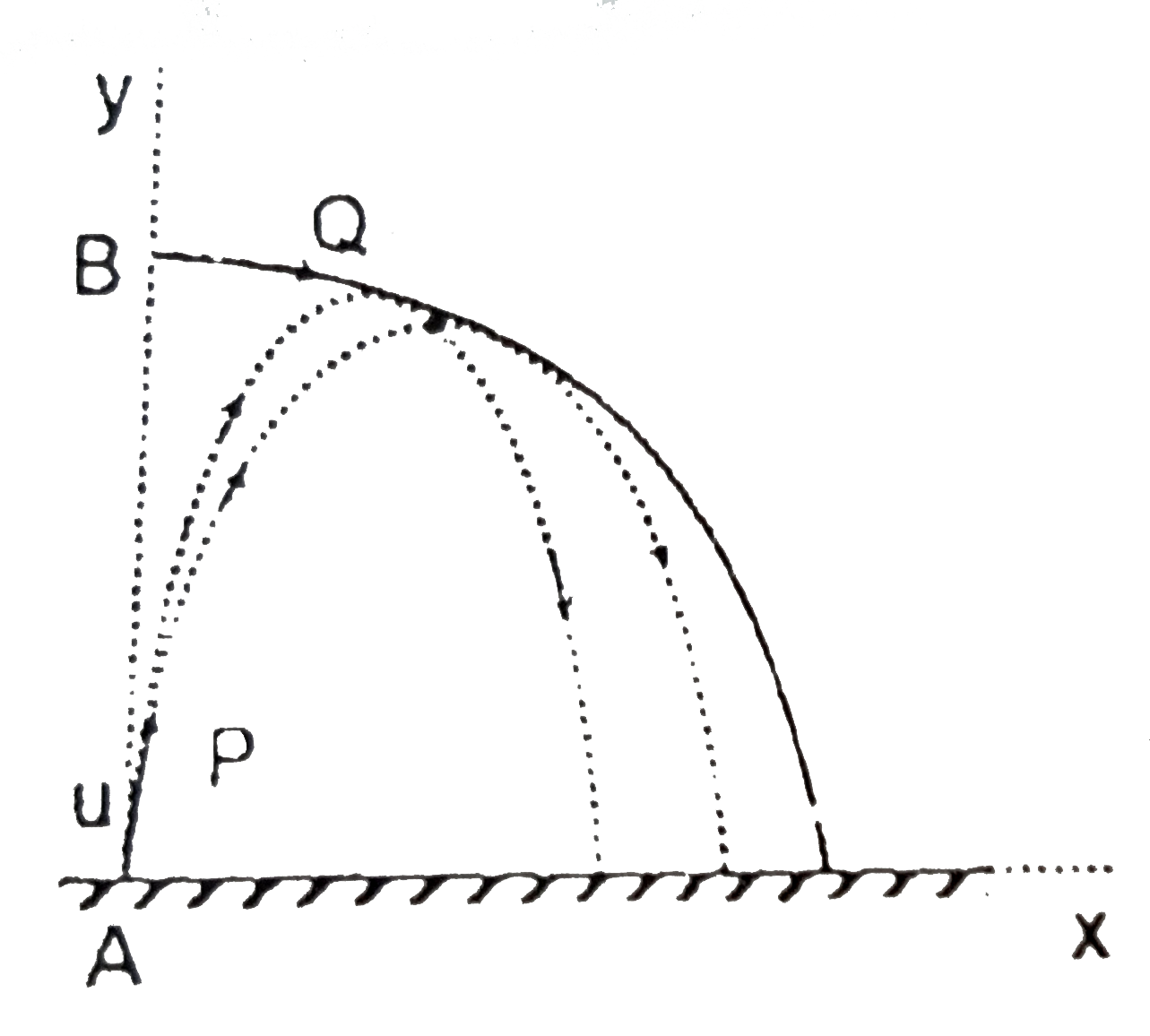
View SolutionA particle moves in the x-y plane with constant acceleration α directed along the negative direction of the y-axis.the equation of the trajectory of the particle is y=ax-bx2, where a and b are constants. Find the velocity of the particle when it passes through the origin.
View SolutionInstantaneous velocity of a particle moving in +x direction is given as v=3x2+2. At t=0, particle starts from origin. Find the average velocity of the particle between the two points p(x=2) and Q(x=4) of its motion path.
View SolutionThe potential energy of a particle of mass 5 kg moving in the x−y plane is given by U=(−7x+24y)J, where x and y are given in metre. If the particle starts from rest, from the origin, then the speed of the particle at t=2s is
View SolutionA particle is moving in the xy plane on a sinusoidal course determined by y = A sin kx, where k and A are constants. The X component of the velocity of the particle is constant and is equal to v0 and the particle was at origin at time t=0. Find the magnitude of the acceleration of the particle when it is at point having x co ordinate x=π2k.
View Solution