A particle $P$ is to be projected from a fixed point $A$ on the ground with a fixed speed $u$ . Another particle $Q$ is also to be projected from a point $B$ which is directly above the point $A$ . The trajectory of the particle $Q$ touches all possible trajectories (for different angle of projection) of the particle $P$ in the vertical $x - y$ plane. Find the equation of trajectory of the particle $Q$ . (Take the point $A$ as origin, horizontal axis $x -$ axis and vertical axis $y -$ axis Consider the figure.)

Text Solution

Verified by Experts

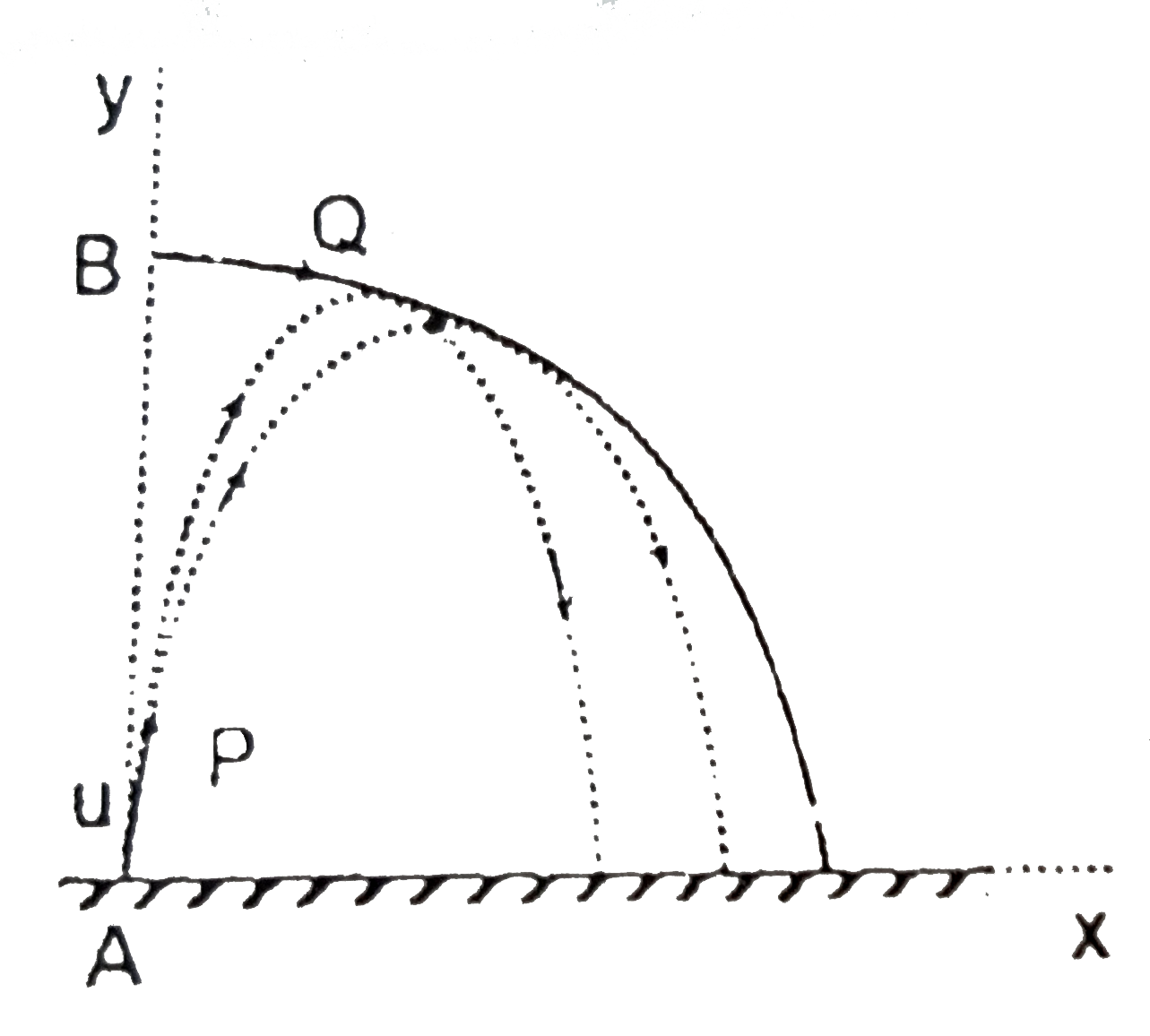
In order for the trahectory of $Q$ to touch that of $P$ when the latter is projected vertically upward, $Q$ must be projected horizontally from the highest point. To visualize this, consider the trajectories of $P$ as the angle of projection of $P$ ( $θ$ ,say), approaches $90^{\circ}$ (i.e. for vertical projection).Let $Q$ be projected horizontally from $B$ with a speed $v$ , where $A = \frac{u^{3}}{2 g}$ . The point of impact of $Q$ on the horizontal plane represents the point of maximum range for $P$ along the horizontal.
$O C = \frac{u^{2}}{g} = v \sqrt{\frac{2 h}{g}}$ , where $h = \frac{u^{2}}{2 g}$
i.e., $v = u$
The trajectory of $Q$ is
$\frac{u^{2}}{2 g} - y = \frac{1}{2} {gt}^{2}$ . where $t = x / v (v \equiv u)$

A particle is projected vertically upward with velocity $u$ from a point $A$ , when it returns to the point of projection .
AIts average speed is $u / 2$ .
BIts average velocity is zero.
CIts displacement is zero.
DIts average speed is $u$ .
A particle is projected from point A on the ground at an angle $θ$ with horizontal. Another particle is projected from point B simultaneously from height $4 H$ above point A with same velocity A and B are in the same vertical plane, where H is the maximum height attained by the particle A, Both the particle collide at the same time on the ground. Find the angle at which particle B is projected with hoizontal.
A $θ$ upwards
B $θ$ downwards
Czero
DNone of these
A particle is projected from the ground with an initial speed of v at an angle $θ$ with horizontal. The average velocity of the particle between its point of projection and highest point of trajectroy is :
A $\frac{v}{2} \sqrt{1 + 2 {cos}^{2} θ}$
B $\frac{v}{2} \sqrt{1 + 2 {cos}^{2} θ}$
C $\frac{v}{2} \sqrt{1 + 3 {cos}^{2} θ}$
D $v cos θ$