A mass m is put atop of a mass M. The mass M vibrates horizontally without friction with the amplitude A = 4 cm at the end of a horizontal Hookean spring. Find the minimal coefficient of friction such that m is not moving with respect to M. Take k = 1000 N/m and m=2 kg, M = 6 kg.

0.5
0.4
0.3
information insufficient
The correct Answer is:A
Force by spring Kx=(m+M)a
for x=A=4cm, Maximum acceleration a=μg to move together, 1000×4/100=(2+6)μ×10 on solving μ=0.5
Topper's Solved these Questions
Similar Questions
A block of mass m is kept on a horizontal table. If the static friction coefficient is μ., find the frictional force acting on the block.
View SolutionA block of mass m is kept on a horizontal table. If the static friction coefficient is μ., find the frictional force acting on the block.
View Solution
Knowledge Check
A block of mass 50 kg slides over a horizontal distance of 1m. If the coefficient of friction between their surface is 0.2, then work done against friction is (take g=9.8m/s2) :
A98 JB72 JC56 JD34 JA force F accelerates a block of mass m on horizontal surface. The coefficient of friction between the contact surface is . The acceleration of m will be -
AF−μmgMBzeroCmay be (A) or (B)DNone of theseThe mass m is placed on a body of mass M. There is no friction. The force F is applied on M on it moves with acceleration a. Then, the force on the top body is
 AFBmaCF - maDNone of these
AFBmaCF - maDNone of these
A block of mass 50 kg slides over a horizontal distance of 1m. If the coefficient of friction between their surface is 0.2, then work done against friction is (take g=9.8m/s2) :
View SolutionA heavy mass m slides down a triangular prism of mass M . The prism lies on a horizontal surface and can move along it without friction . What will be the direction of motion of m if the inclination of the hypotenuse of the prism is α with the horizontal ?
View SolutionA skater of mass m standing on ice throws a stone of mass M =2m with a velocity of v=5m/s in horizontal direction. If the coefficient of friction between the skater and the ice is 0.5 , find the distance over which the skater will move ( take g=10ms−2)
View SolutionA body A mass m1=1kg and body B of mass m2=4.1kg. The body A perform free vertical harmonic oscillations with the amplitude 1.6cm and frequency 25Hz. Neglecting the mass of the spring , find the maximum and m inimum value of force that the system exerts on the hearing surface.
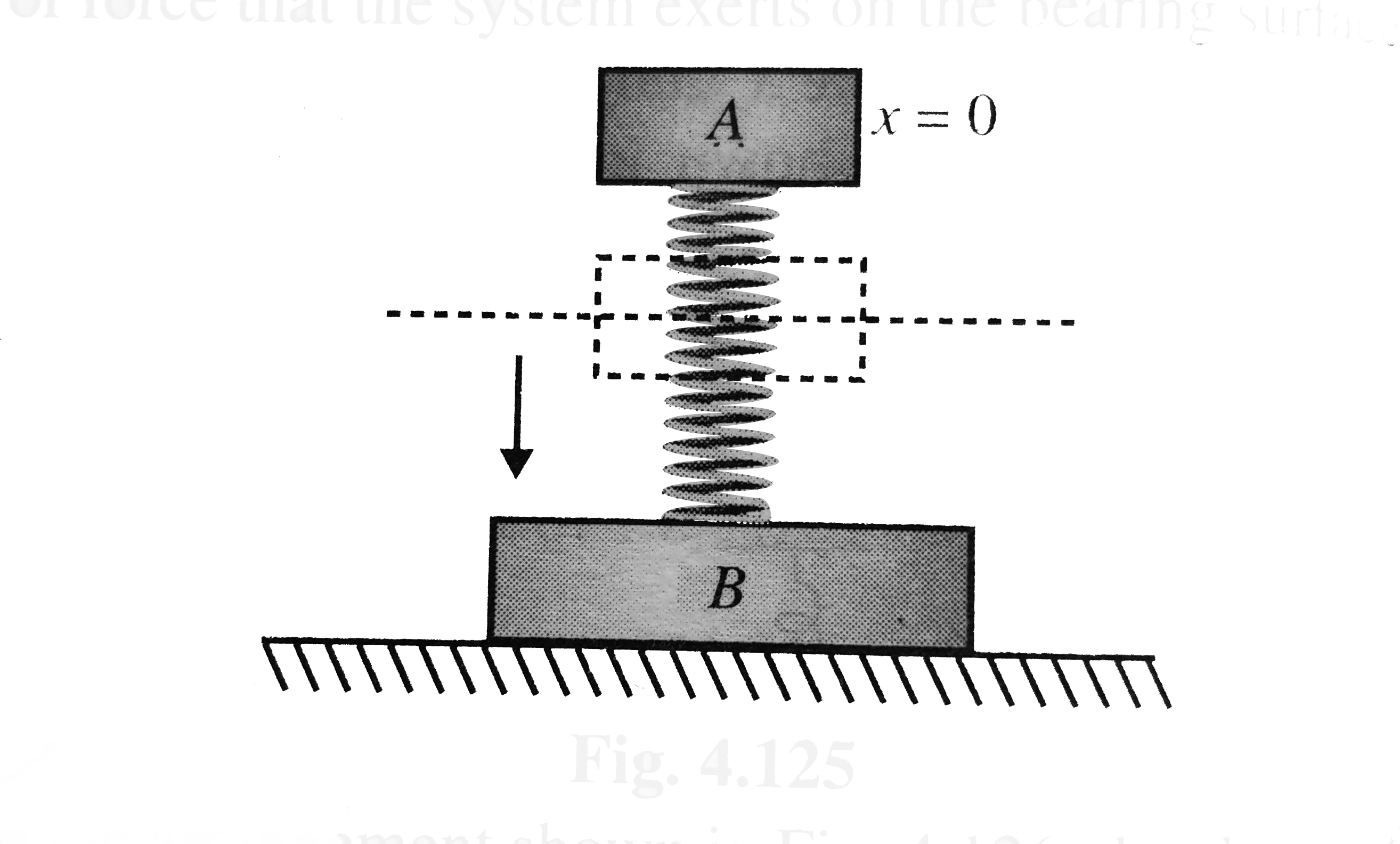
View SolutionA mass m, connected to a horizontal spring performs SHM with amplitude A. While mass m, is passing through mean position, another mass m, is placed on it so that both the masses move together with amplitude A,. The ratio of
View Solution
