The displacement of an oscillator is given by
x=asinωt+bcosωt
where a, b and ω, are constant. Then -
motion is simple harmonic but not periodic
motion is periodic but not simple harmonic
motion is simple harmonic as well as periodic
motion is neither simple harmonic nor periodic
The correct Answer is:C
Sum of 2 periodic function is a periodic function with same period.
Topper's Solved these Questions
Similar Questions
Knowledge Check
Current in an ac circuit is given by I=3sinωt+4cosωt, then
Arms value of current is 5A.Bmean value of this current in any one half period will be (6/π).CIf voltage applied is V=Vmsinωt, then the circuit may be containing resistance and capacitance only.DIf voltage applied is V=Vmsinωt, then the circuit may be contain resostamce and inductance only.The equation of displacement of a harmonic oscillator is x =3sinωt+4 cosωtamplitude is
A2B5C7D12A particle moves in xy plane according to the law x = a sin ωtand y = a(1 – cosωt) where a and ω are constants. The particle traces
Aa parabolaBa straight line equallyinclined to x and y axesCa circleDa distance proportional to time
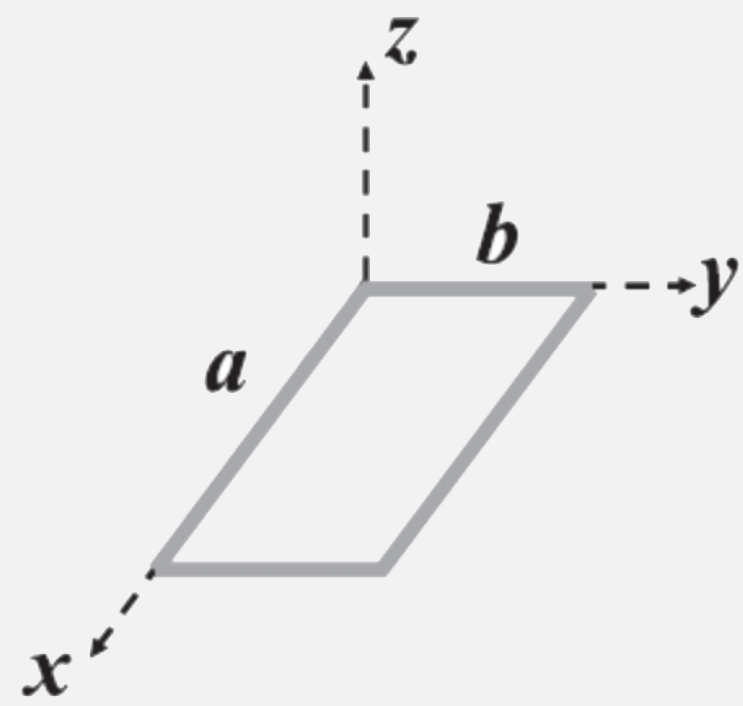
A rectangular wire loop with length a and width b lies in the xy-plane as shown. Within the loop, there is a time dependent magnetic field given by →B=c[(xcosωt)ˆi+(ysinωt)ˆk]. Here , c and ω are constants. The magnitude of emf induced in the loop as function of time is
View SolutionThe displacement of a particle is given at time t, by:
x=Asin(−2ωt)+Bsin2ωt Then,
View SolutionThe motion of a particle is given by x=Asinωt+Bosωt. The motion of the particle is
View SolutionThe motion of a particle is given by
y=asinωt+bsinωt
The motion of the particle is
View SolutionFor a particle moving in the x-y plane, the x and y coordinates are changing as x=a sin ωt and y=a(1−cosωt), where 'a' and ω constants. Then, what can be inferred for the trajectory of the particle ?
View Solution