In Fig. 4.30, two identical parallel plate air capacitors are connected to a battery. At first the key S is closed and then it is opend. The space between the two capacitors are now filled up with a dielectric, having dielectric constant 3. Determine the ratio of the energy stored in the two capacitors before and after insertion of the dielectric?
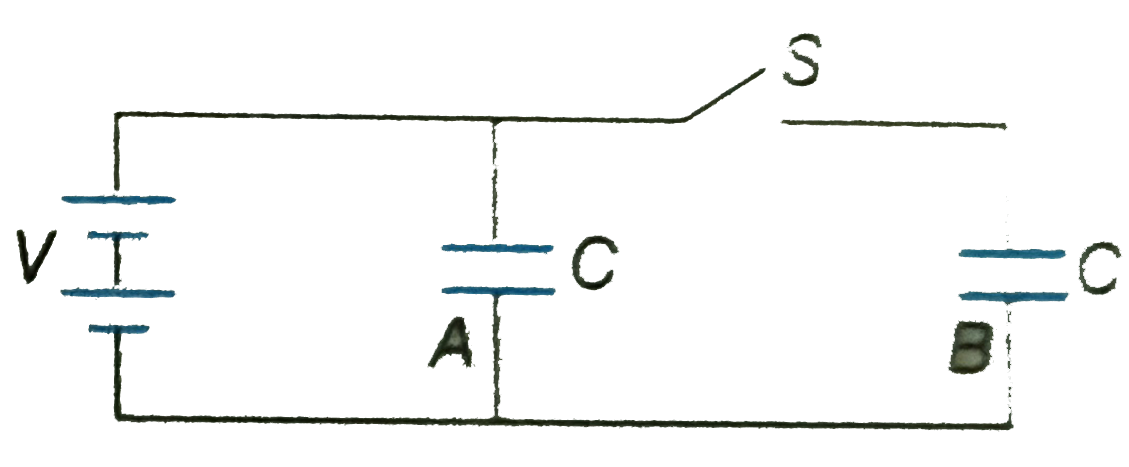
When the key was closed, potential of each capacitor was equal. Let this value of potential be V.
Energy of the capacitor A=12CV2
and energy of the capacitor B=12CV2
Total energy =12CV2+12CV2=CV2
When the key is opened and the intervening space is filled up with the dielectric of each capacitor becomes 3C.
Since the capacitor A is still connected to the battery, its potential remains at V.
Energy of the capacitor A
=12×3C×V2=32CV2
Total charge of the capacitor B,Q = CV
This charge of B remains unaltered even after opening the key and inserting the dielectric.
Energy of B=12⋅Q23C=12C2V23C=CV26
Energy of the two capacitors =32CV2+12CV2=53CV2
The required ratio =CV2(53)CV2=35.
Topper's Solved these Questions
- CAPACITANCE AND CAPACITORBOOK - CHHAYA PUBLICATIONCHAPTER - CAPACITANCE AND CAPACITOREXERCISE - SECTION RELATED QUESTIONS
30 Videos
- CAPACITANCE AND CAPACITORBOOK - CHHAYA PUBLICATIONCHAPTER - CAPACITANCE AND CAPACITOREXERCISE - HIGHER ORDER THINKING SKILL
39 Videos
- ATOMIC NUCLEUSBOOK - CHHAYA PUBLICATIONCHAPTER - ATOMIC NUCLEUSEXERCISE - CBSE SCANNER
14 Videos
- COMMUNICATION STSTEMBOOK - CHHAYA PUBLICATIONCHAPTER - COMMUNICATION STSTEMEXERCISE - CBSE SCANNER
28 Videos
Similar Questions
Two identical parallel plate capacitors A and B are connected to a battery of V volts with the switch S closed . The switch is now opened and the free space between the plates of the capacitors is filled with dielectric of dielectric constant K . Find the ratio of the total electrostatic energy stored in both capacitors before and after the introduction of the dielectric .
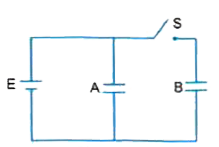
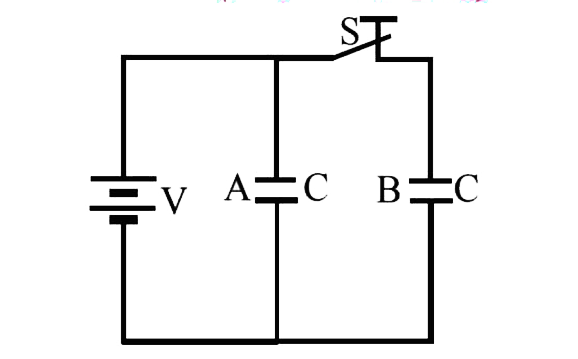
View SolutionThe figure shows two identical parallel plate capacitors connected to a battery with the switch S closed. The switch is now opened and the free space between the plates of the capacitors is filled with a dielectric of dielectric constant(or relative permittivity) 3. Find the ratio of the total electrostatic energy stored in both capacitors before and after the introduction of the dielectric.
View Solution
Knowledge Check
Two parallel plate air filled capacitors each of capacitance C, are joined in series to a battery of emf V. The space between the plates of one of the capacitors is then completely filled up with a uniform dielectric having dielectric constant K. The quantity of charge which flows through the battery is -
ACV2(K−1K+1)BCV2(K+1K−1)CCV(K−1K+1)DCV(K+1K−1)
The figure shows two identical parallel plate capacitors connected to a battery with the switch S closed. The switch is now opened and the free space between the plates of the capacitors is filled with a dielectric of dielectric constant(or relative permittivity) 3. Find the ratio of the total electrostatic energy stored in both capacitors before and after the introduction of the dielectric.

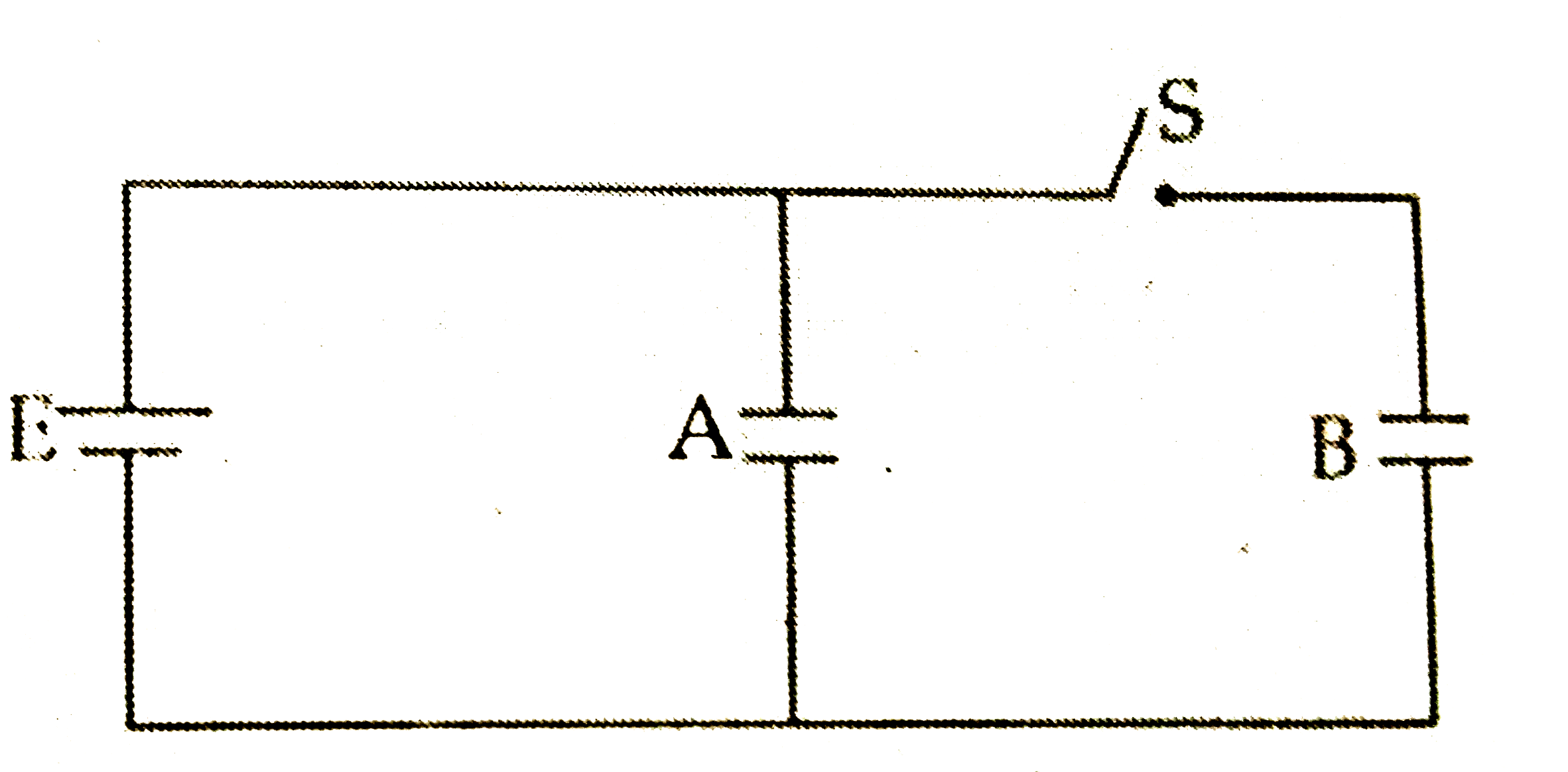
View SolutionTwo identical parallel plate capacitors A and B are
connected to a battery of V volts with the switch S
closed . The switch is now opend and the free
space between the plantes of the capacitors is filled
with a dielectric of dielectric constant K. Find the
rario of the total electrostatic energy stored in both
capacitors before and after the introduction of the dielectric.
View SolutionTwo parallel plate capacitors, each of capacitance 40 muF, are connected is series. The space between the plates of one capacitor is filled with a dielectric material of dielectric constant K =4. Find the equivalent capacitacne of the system.
View SolutionTwo parallel plate capacitors, each of capacitance 40 muF, are connected is series. The space between the plates of one capacitor is filled with a dielectric material of dielectric constant K =4. Find the equivalent capacitacne of the system.
View SolutionA cell of voltage V0 is connected across a capacitor of capacitance C. Now the space between the plates is filled with a material of dielectric constant K. Find ratio of charge appear on the plates of capacitor before and after filling.
View SolutionA parallel plate capacitar, of capacitance, 20μF, is connected to a 100 V supply. After some time, the battery is disconnected , and the space, between the plates of the capacitor is filled with a dielectric, of dielectric constant 5. Calculate the energy stored in the capacitar (i) before (ii)after dielectric has been put in between its plates.
View SolutionA parallel plate capacitar, of capacitance, 20μF, is connected to a 100 V supply. After some time, the battery is disconnected , and the space, between the plates of the capacitor is filled with a dielectric, of dielectric constant 5. Calculate the energy stored in the capacitar (i) before (ii)after dielectric has been put in between its plates.
View Solution
