Calculate the pressure at a depthe 'h' in a liquid.

Text Solution

Verified by Experts

1. Let us consider a container which contains a liquid in it of density $' ρ'$
2. Consider cylindrical column of height 'h' from the surface of the liquid of cross sectional area "A".
3. The volume of the liquid column V = Ah
$Mass = Volume \times density$
$\Rightarrow m = A h ρ$
$Weight W = m g = A h ρ g$
From Newton's law the force on the liquid column is zero, because it is at rest.
4. The forces acting on the water column are
a. Weight (W), vertically down
b. Force on the surface due to atmospheric pressure $(P_{\circ} A)$ acting vertically down.
c. Froce on the botton surface of the column due to static pressure of liquid (PA), acting vertically up.
5. From Newton's law we get
$P A = P_{\circ} A + W \Rightarrow P A = P_{\circ} A + h ρ g A \Rightarrow P A = A (P_{\circ} + h ρ g)$
$∴ P = P_{\circ} + h ρ g$
P = Pressure at depth 'h' from the surface of the liquid
$P_{\circ}$ = Atmospheric pressure
$∴$ The pressure inside the liquid at a constant depth is constant depth is constant.

Updated on:21/07/2023

Calculate the pressure inside a small air bubble of radus $r$ situated at a depth $h$ below the free surface of liquids of densities $ρ_{1}$ and $ρ_{2}$ and surface tennsions $T_{1}$ and $T_{2}$ . The thickness of the first and second liquids are $h_{1}$ and $h_{2}$ respectively. Take atmosphere pressure $= P_{0}$ .

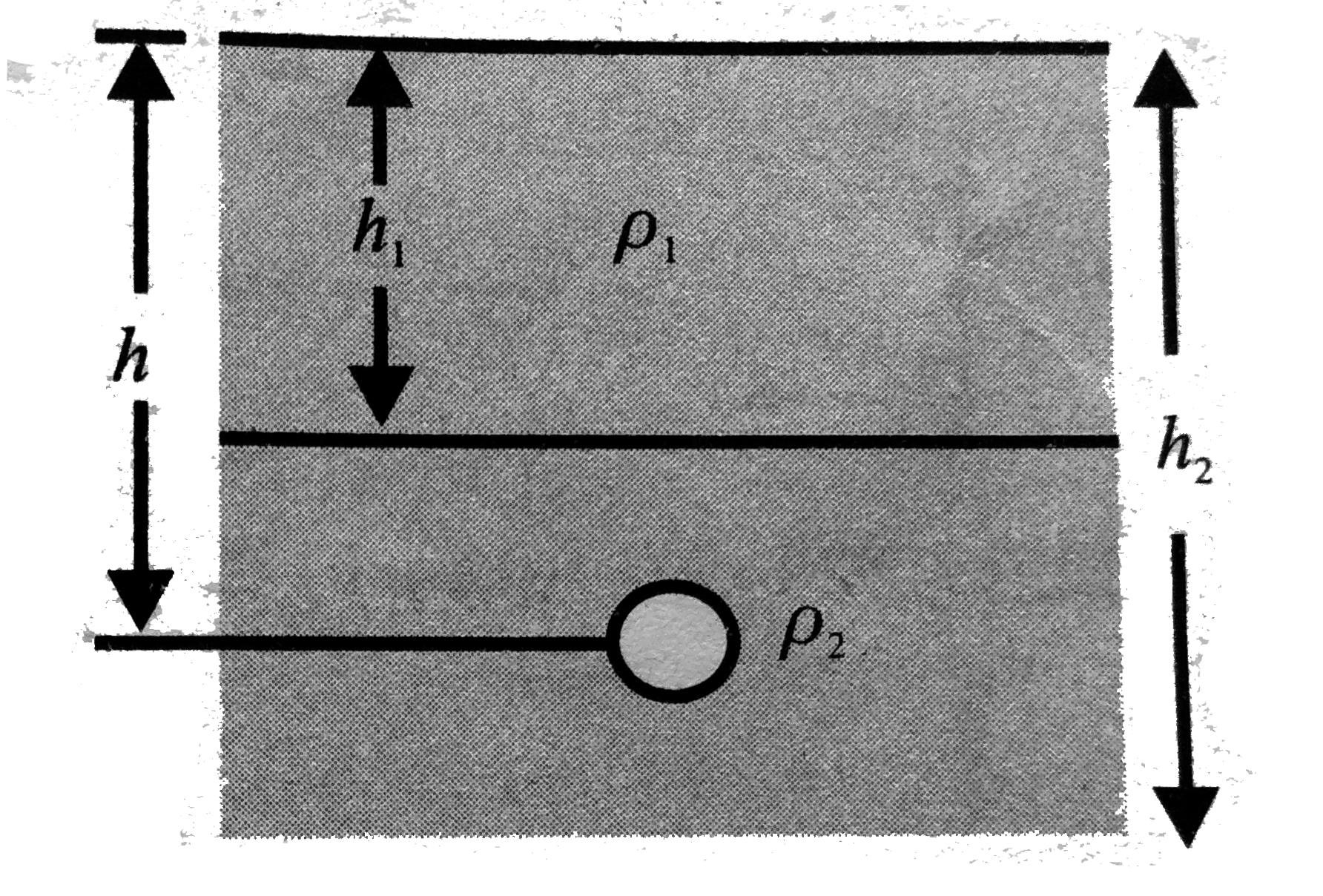A $P_{0} + ρ_{1} g h_{1} + ρ_{2} g (h - h_{1}) - \frac{2 T_{2}}{r}$
B $P_{0} + ρ_{1} g h_{1} + ρ_{2} g (h - h_{1}) + \frac{2 T_{2}}{r}$
C $P_{0} - ρ_{1} g h_{2} + ρ_{2} g (h - h_{1}) + \frac{2 T_{2}}{r}$
Dnone of these
The absolute pressure at a depth h below the surface of a liquid of density $ρ$ is [Given $P_{a}$ = atmospheric pressure, g = acceleration due to gravity]
A $ρ g h$
B $P_{a} + ρ g h$
C $P_{a} - ρ g h$
D $P_{a} 2 ρ g h$
For a liquid in an open cantainer, the total pressure at any depth depends on:
AAtmospheric pressure
Bliwuid density
Cacceleration due to gravity
Dall of the precending