A particle of mass m is hanging vertically by an ideal spring of force constant K . If the mass is made to oscillate vertically, its total energy is
Maximum at extreme position
Maximum at mean position
Minimum at mean position
Same at all position
The correct Answer is:D
To solve the problem regarding the total energy of a particle of mass m hanging vertically by an ideal spring of force constant K and oscillating vertically, we can follow these steps:
Step 1: Understanding the System
The particle is attached to a spring and is allowed to oscillate vertically. The forces acting on the particle include the gravitational force and the spring force.
Step 2: Define the Energy Types
In this system, we have two main types of energy:
- Potential Energy due to Gravity (PE_gravity): This is given by PEgravity=mgh, where h is the height of the mass from a reference point.
- Potential Energy of the Spring (PE_spring): This is given by PEspring=12kx2, where x is the displacement from the spring's natural length.
Step 3: Total Mechanical Energy
The total mechanical energy E in the system is the sum of the gravitational potential energy and the spring potential energy:
E=PEgravity+PEspring
Step 4: Energy at Different Positions
- At the Mean Position: The particle has maximum kinetic energy and minimum potential energy. The total energy is still conserved.
- At the Extreme Positions: The particle has maximum potential energy (either gravitational or spring) and zero kinetic energy.
Step 5: Conservation of Energy
Throughout the oscillation, the total energy remains constant. It transforms between kinetic energy and potential energy but the total energy does not change.
Conclusion
Thus, the total energy of the system is the same at all positions during the oscillation. Therefore, the answer to the question is that the total energy is the same at all positions.
Final Answer
The total energy is the same at all positions.
---
The particle is attached to a spring and is allowed to oscillate vertically. The forces acting on the particle include the gravitational force and the spring force.
In this system, we have two main types of energy:
- Potential Energy due to Gravity (PE_gravity): This is given by PEgravity=mgh, where h is the height of the mass from a reference point.
- Potential Energy of the Spring (PE_spring): This is given by PEspring=12kx2, where x is the displacement from the spring's natural length.
The total mechanical energy E in the system is the sum of the gravitational potential energy and the spring potential energy:
E=PEgravity+PEspring
- At the Mean Position: The particle has maximum kinetic energy and minimum potential energy. The total energy is still conserved.
- At the Extreme Positions: The particle has maximum potential energy (either gravitational or spring) and zero kinetic energy.
Throughout the oscillation, the total energy remains constant. It transforms between kinetic energy and potential energy but the total energy does not change.
Thus, the total energy of the system is the same at all positions during the oscillation. Therefore, the answer to the question is that the total energy is the same at all positions.
The total energy is the same at all positions.
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - ERRORLESS CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Time period and frequency
18 Videos
- SIMPLE HARMONIC MOTIONBOOK - ERRORLESS CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - simple pendulum
61 Videos
- SIMPLE HARMONIC MOTIONBOOK - ERRORLESS CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Acceleration of simple Harmonic motion
21 Videos
- ROTATIONAL MOTIONBOOK - ERRORLESS CHAPTER - ROTATIONAL MOTIONEXERCISE - Practice Problems (Problems based on motion of connected mass)
10 Videos
- SURFACE TENSIONBOOK - ERRORLESS CHAPTER - SURFACE TENSIONEXERCISE - Exercise
214 Videos
Similar Questions
A particle of mass m is attached to three identical massless springs of spring constant k as shown in the m 135 figure. The time period of vertical oscillation of the particle is

View SolutionA flat spiral spring of force constant K is loaded with mass M and oscillates vertically with a time period T. Then the mass suspended to the free end is
View Solution
Knowledge Check
A particle of mass m is attached to three identical springs of spring constant k as shwon in figure. The time period of vertical oscillation of the particle is
 A2π√mkB2π√m2kC2π√m3kDπ√mk
A2π√mkB2π√m2kC2π√m3kDπ√mkA particle of mass 'm' is attached to three identical springs A,B and C each of force constant 'K' as shown in figure. If the particle of mass 'm' is pushed slightly against the spring 'A' and released the period of oscillations is
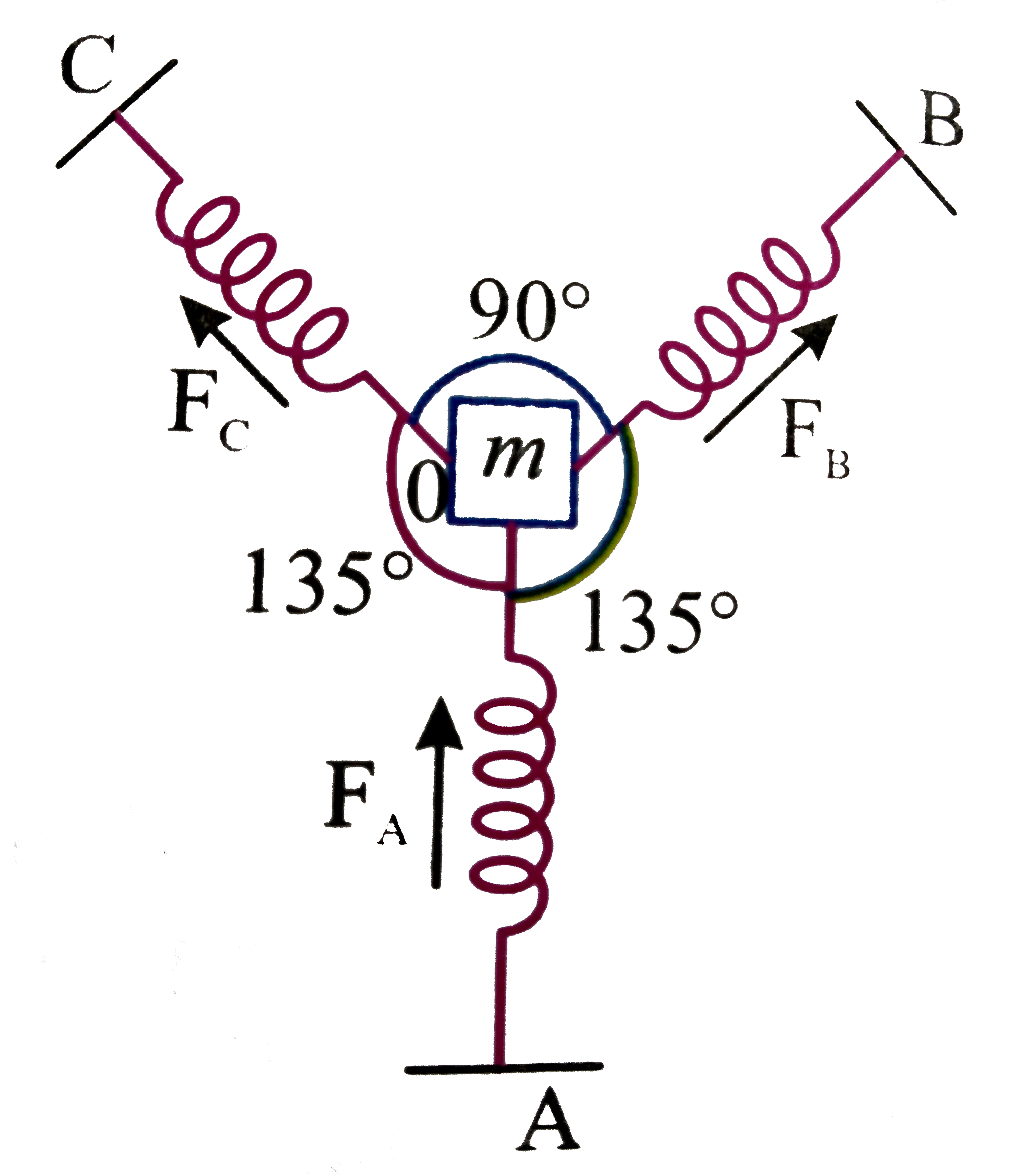 AT=2π√mKBT=2π√2mKCT=2π√m2KDT=π√mK
AT=2π√mKBT=2π√2mKCT=2π√m2KDT=π√mKThe period of oscillation of a mass M, hanging from a spring of force constant k is T. When additional mass m is attached to the spring, the period of oscillation becomes 5T/4. m/M =
A9:16B25:16C25:9D16:9
A body of mass 'm' is suspended to an ideal spring of force constant 'k'. The expected change in the position of the body due to an additional force 'F' acting vertically downwards is
View SolutionA load of mass M is attached to the bottom of a spring of mass 'M/3' and spring constant 'K'. If the system is set into oscillation, the time period of oscillation is
View SolutionA simple harmonic oscillator consists of a particle of mass m and an ideal spring with spring constant k. The particle oscillates with a time period T. The spring is cut into two equal parts. If one part oscillates with the same particle, the time period will be
View SolutionA ball of mass mkg hangs from a spring of spring constant k. The ball oscillates with a period of T seconds if the ball is removed, the spring is shortened(w.r.t. length in mean position) by
View SolutionThe frequency f of vibrations of a mass m suspended from a spring of spring constant k is given by f=Cmxky , where C is a dimensionnless constant. The values of xandy are, respectively,
View Solution
