MNOP is a parallelogram. Q and R are point on sides MN and ON respectively. (△PRM)=12cm2, find ar(△POQ)
ΔPRM and parallelogram MNOP have same base and R is a point on side ON of the parallelogram.
∴ar(MNOP)=2∗ar(PRM)=2∗12=24cm2
Now, ΔPOQ and parallelogram MNOP have same base PO and Q is a point on side MN of the parallelogram.
∴ar(MNOP)=2∗ar(ΔPOQ)
⇒ar(ΔPOQ)=242=12cm2
Similar Questions
Knowledge Check
In the given figure, MNOP is a parallelogram. PM is extended to Z. OZ intersects MN and PN at Y and X respectively. MY = YN. If OX = 27 cm. and XY = 18 cm., then what is the length (in cm.) of YZ?
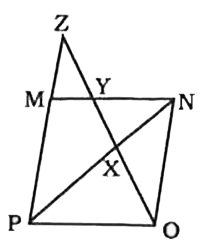 A21.4B22.5C23.8D24.5
A21.4B22.5C23.8D24.5
Let ABCD be a parallelogram of area 124cm2. If E and F are the mid-points of sides AB and CD respectively, then find the area of parallelogram AEFD.
View SolutionABCD is a parallelogram and O is the point of intersection of its diagonals. If ar(△AOD)=4cm2 find ar(△AOB)
View SolutionLet ABCD be a parallelogram of area 124cm2 If E and F are the mid-points of the sides AB and CD respectively then find the area of the parallelogram AEFD
View SolutionLet ABCD be a parallelogram of area 124 cm2. If E and F are the mid-points of sides AB and CD respectively, then find the area of parallelogram AEFD
View SolutionIn the given figure, ABCDEF is a regular hexagon of side 12cm. P, Q and R are the mid points of the sides AB, CD and EF respectively. What is the area (in cm2) of triangle PQR ?
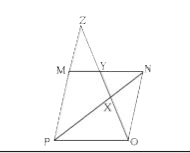
View SolutionIn the given figure, MNOP is a parallelogram. PM is extended to Z. OZ intersects MN and PN at Y and X respectively. If OX=27cmandXY=18cm, then what is the length (in cm) of YZ?
View Solution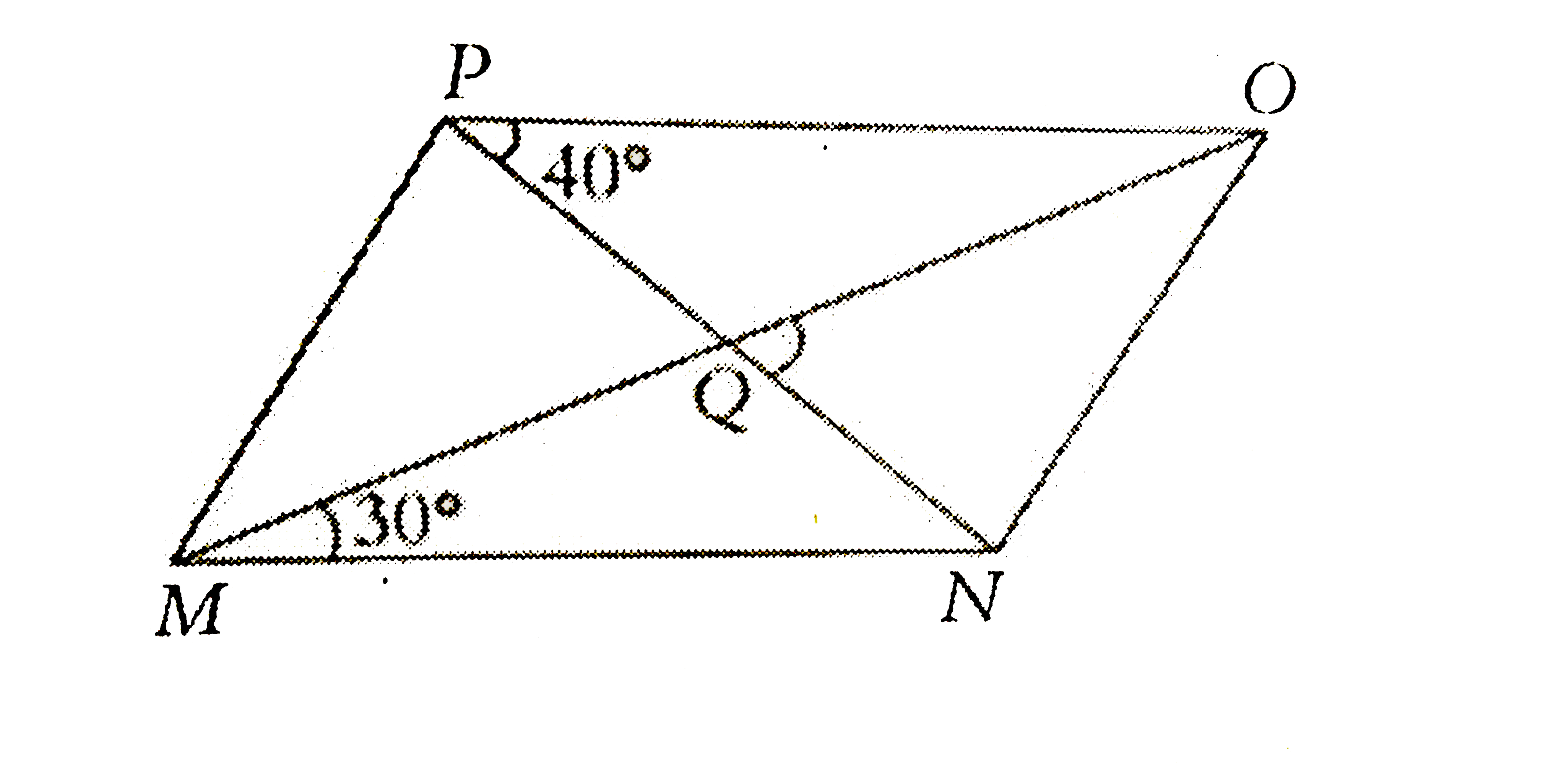
In the figure above, MNOP is a parallelogram, diagonals MO and PN intersect at Q, ∠OPQ=40∘and∠OMN=30∘. Find ∠OQN.
View Solution
