Two men each of mass stand on the rim of a horizontal circular disc, diametrically opposite to each other. The disc has a mass and is free to rotate about a vertical axis passing through its centre of mass. Each man start simultaneously along the rim clockwise and reaches their original starting points on the disc. The angle turned through by the disc with respect to the ground (in radian) is:
The correct Answer is:A
Topper's Solved these Questions
- RIGID BODY MOTIONBook:ANURAG MISHRAChapter:RIGID BODY MOTIONExercise:COMPREHENSION_TYPE
Explore 29 Videos
- RIGID BODY MOTIONBook:ANURAG MISHRAChapter:RIGID BODY MOTIONExercise:MATCH THE COLUMN
Explore 12 Videos
- RIGID BODY MOTIONBook:ANURAG MISHRAChapter:RIGID BODY MOTIONExercise:SCQ_TYPE
Explore 83 Videos
- IMPULSE AND MOMENTUMBook:ANURAG MISHRAChapter:IMPULSE AND MOMENTUMExercise:matching
Explore 3 Videos
- UNIT AND DIMENSIONSBook:ANURAG MISHRAChapter:UNIT AND DIMENSIONSExercise:Matching Type Problem
Explore 4 Videos
Similar Questions
Two men each of mass m stand on the rim of a horizontal circular disc, diametrically opposite to each other. The disc has a mass M and is free to rotate about a vertical axis passing through its centre of mass. Each mass start simultaneously along the rim clockwise and reaches their original starting positions on the disc. The angle turned through by disc with respect to the ground (in radian) is
View SolutionTwo men, each of mass 75kg, stand on the rim of a horizontal large disc, diametrically opposite to each other. The disc has a mass 450kg and is free to rotate about its axis. Each man simultaneouslt start along the rim clockwise with the same speed and reaches their original starting points on the disc. Find the angle turned through by the disc with respect to the ground.
View Solution
Knowledge Check
Two men each of mass m stand on the rim of a horizontal circular disc, diametrically opposite to each other. The disc has a mass M and is free to rotate about a vertical axis passing through its centre of mass. Each mass start simultaneously along the rim clockwise and reaches their original starting positions on the disc. The angle turned through by disc with respect to the ground (in radian) is
A8mπ4m+MB2mπ4m+MCmπM+mD4mπ2M+mA disc is free to rotate about a smooth horizontal axis passing through its centre of mass. A particle is fixed at the top of the disc. A slight push is given to the disc and it starts rotating. During the process.
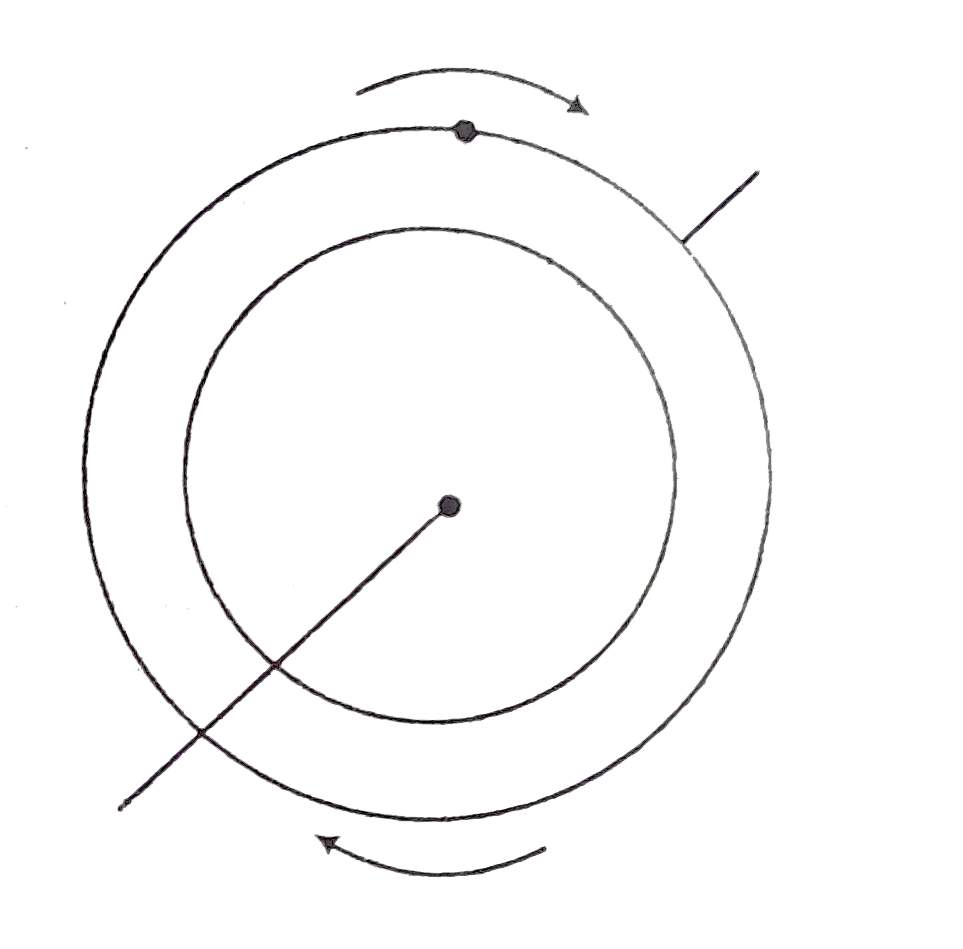 Aonly mechanical energy is conservedBonly angular momentum (about the axis of rotation) is conservedCboth mechanical energy and angular momentum are conservedDNeither the mechanical energy nor the angular momentum are conserved.
Aonly mechanical energy is conservedBonly angular momentum (about the axis of rotation) is conservedCboth mechanical energy and angular momentum are conservedDNeither the mechanical energy nor the angular momentum are conserved.
A disc is free to rotate about a smooth horizontal axis passing through its centre of mass. A particle is fixed at the top of the disc. A slight push is given to the disc and it starts rotating. During the process.

View SolutionA man of mass m stands on the edge of a horizontal unifom disc of mass M and radius R whichis capable of rotating freely about a stationary vertical axis passing through its center. At a certain moment the man starts moving along the edge of the disc, he shifts over angle θ relative to the disc and then stops. Find the angle through which the disc rotates.
View SolutionA horizontal disc is rotating about a vertical axis passing through its centre. If an insect moves from centre to rim then the angular momentum of the system
View SolutionA horizontal disc is rotating about a vertical axis passing through its centre. If an insect moves from centre to rim then the angular momentum of the system
View SolutionA horizontal disc is rotating about a vertical axis passing through its centre. If an insect moves from centre to rim then the angular momentum of the system
View SolutionA man of mass m1 stands on the edge of a horizontal uniform disc of mass m2 and radius R which is capable of rotating freely about a stationary vertical axis passing through its centre . The man walks along the edge of the disc through angle θ relative to the disc and then stops . find the angle through which the disc turned the time the man stopped .
[2m1θ2m1+m2]
View Solution
