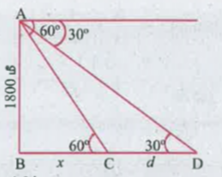
An aeroplane at an altitude of 1800m finds that two boats are selling towards it in the same direction. The angles of depressionn of the boats as obversed from the aeroplane are 60∘and30∘ respectively. Find the distance between the two boats. (√3=1.732).Solution in Tamil
The correct Answer is:2078.4 m
Step by step video, text & image solution for An aeroplane at an altitude of 1800m finds that two boats are selling towards it in the same direction. The angles of depressionn of the boats as obversed from the aeroplane are 60^(@) and 30^(@) respectively. Find the distance between the two boats. (sqrt(3)=1.732). by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TRIGONOMETRYBOOK - SURA PUBLICATIONCHAPTER - TRIGONOMETRYEXERCISE - EXECRISE 5.4
9 Videos
- TRIGONOMETRYBOOK - SURA PUBLICATIONCHAPTER - TRIGONOMETRYEXERCISE - EXECRISE 5.5
15 Videos
- TRIGONOMETRYBOOK - SURA PUBLICATIONCHAPTER - TRIGONOMETRYEXERCISE - EXECRISE 5.2
9 Videos
- SURAS MODEL QUESTION PAPER-2019BOOK - SURA PUBLICATIONCHAPTER - SURAS MODEL QUESTION PAPER-2019EXERCISE - Part-IV
3 Videos
Similar Questions
From the top of tree of height 13m the angle of elevation and depression of the top and bottom of another tree are 45∘and30∘ respectively. Find the height of the seconds tree. (√3=1.732).
View SolutionThe value of sin2θ+11+tan2θ is equal to
View SolutionFind the angle of elevation of the top of a tower from a point on the ground, which is 30 m away the foot of a tower of height 10√3m.
View SolutionConstruct a △PQR in which the base PQ=4.5 cm, ∠R=45∘ and the median from R to RG is 6cm.
View Solutionमध्य समुद्र में दो नाव विपरीत दिशाओं से एक प्रकाश-ग्रह की तरफ आ रही हैं | दोनों नावों से प्रकाश-ग्रह के शिखर का उन्नयन कोण क्रमशः 30∘ तथा 45∘ हैं | यदि दोनों नावों के बीच की दूरी 100 मीटर हो,तो प्रकाश-ग्रह की ऊँचाई ज्ञात कीजिए |
View Solution15 मीटर ऊच एक पुल से एक नाव का अवनमन कोण 30∘ है पुल के आधार से नाव की दूरी ज्ञात कीजिए।
View SolutionAn observer flying in an aeroplane at an altitude of 900 m observes two ships in front of him, which are in the same direction at an angles of depression of 60∘ and 30∘respectively. Find the distance between the two ships.
View Solution1800மீ உயரத்தில பறக்கும் ஒரு விமானத்திலிருந்து ஒரே திசையில் விமானத்தை நோக்கிச் செல்லும் இருபார்க்கப்படுகிறது. விமானத்திலிருந்து இரு படகுகளை முறையே 60∘ மற்றும்30∘ இறக்கக் கோணங்களில் உற்று நோக்கினால், இரண்டு படகுகளுக்கும் இடைப்பட்டத் தொலைவைக் காண்க. (√3=1.732)
View SolutionThe angles of elevation of an aeroplane from two places 10 km apart are found to be 60∘and30∘ respectively. Find the height of the aeroplane.
View SolutionAn army pilot flying an aeroplane at an altitude of 1800 m observes two ships sailing towards it in the same direction and immediately reports it to the navy chief. The angle of depression of the ships as observed from the aeroplane is 60∘ and 30∘ respectively.
[a] Find the distance between two ships.
[b] What value has the pilot shown?
View Solution300 मी ऊँचे टॉवर के शिखर पर खड़ा प्रेक्षक , एक ही दिशा में तैरने वाले दो नावों का अवनमन कोण क्रमशः 60∘ तथा 30∘ मापता है। दोनों नावों के बीच की दुरी होगी।
View SolutionTwo boats approach a light house in mid-sea from opposite directions. The angles of elevation of the top of the light house from two boats are 300and450 respectively. If the distance between two boats is 100m, find the height of the light house.
View SolutionAn aeroplane at an altitude of 1200 metres finds that two ships are sailing towards it in the same direction. The angles of depression of the ships as observed from the aeroplane are 60o and 30o respectively. Find the distance between the two ships.
View Solution200 मी ऊँची एक शिखर की चोटी से प्रेक्षण के दक्षिण में स्थित दो नावों के अवनमन कोण 60∘ एवं 30∘ है दोनों नावों के बीच की दूरी ज्ञात कीजिए।
View SolutionAn observer on the top of a monument 500 m above the sea level, observes the angles of depression of the two boats to be 45∘ and 30∘ respectively. Find the distance between the boats if the boats are-
On the same side of the monument,
View Solution
