In the figure shown surface is frictionless and spring is in natural condition. If x1,x2 and x3 are the maximum compression in spring for elastic, completely inelastic and inelastic (e=0.5) respectively then :
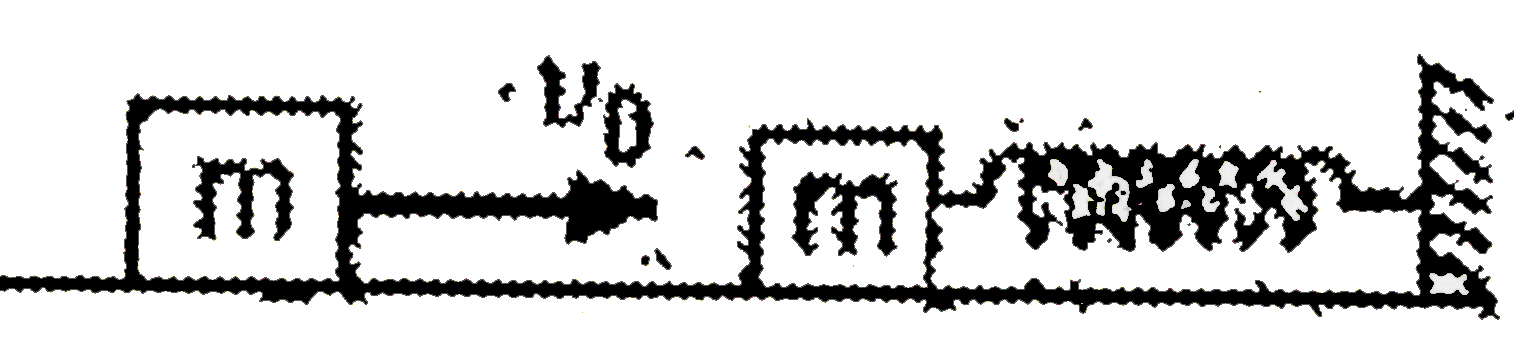
x1>x2>x3
x2>x3>x1
x1>x3>x2
x2>x1>x3
The correct Answer is:C
Step by step video, text & image solution for In the figure shown surface is frictionless and spring is in natural condition. If x_(1), x_(2) " and " x_(3) are the maximum compression in spring for elastic, completely inelastic and inelastic (e=0.5) respectively then : by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- IMPULSE AND MOMENTUMBook:ANURAG MISHRAChapter:IMPULSE AND MOMENTUMExercise:L-2
Explore 21 Videos
- IMPULSE AND MOMENTUMBook:ANURAG MISHRAChapter:IMPULSE AND MOMENTUMExercise:L-3,(P-1)
Explore 5 Videos
- IMPULSE AND MOMENTUMBook:ANURAG MISHRAChapter:IMPULSE AND MOMENTUMExercise:Example
Explore 50 Videos
- FORCE ANALYSISBook:ANURAG MISHRAChapter:FORCE ANALYSISExercise:Matching type
Explore 13 Videos
- RIGID BODY MOTIONBook:ANURAG MISHRAChapter:RIGID BODY MOTIONExercise:MATCH THE COLUMN
Explore 12 Videos
Similar Questions
The spring constant of the spring shown in the figure is 250Nm−1. Find the maximum compression of the spring?

View Solution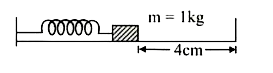
In the figure, the spring is initially compressed by 3 cm and released. The block collides withth wall and rebounds to compress the spring again. The maximum compression in the srping after collision (coefficient of restitution is 0.7) is ........cm (Take √5=2.4,k=104Nm−1)
View Solution
Knowledge Check
The mass in the figure can slide on a frictionless surface. When the mass is pulled out, spring 1 is stretched a distance x1and spring 2 is stretched a distance x2. The spring constants are k1andk2 respectively. Magnitude of spring force pulling back on the mass is
 Ak1x1Bk2x2C(k1x1+k2x2)D0.5(k1+k2)(x1+x2)
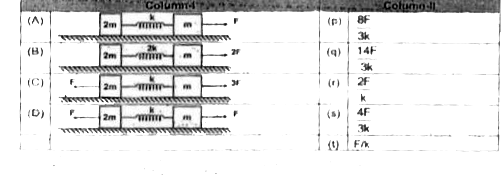
Ak1x1Bk2x2C(k1x1+k2x2)D0.5(k1+k2)(x1+x2)Consider three cases, same spring is attached with 2kg , 3kg and 1kg blocks as shown in figure. If x1 , x2 , x3 be the extensions in the spring in the three cases, then.
 Ax2>x1>x3Bx1>x2>x3Cx1=0 , x3>x2Dx3>x2>x1
Ax2>x1>x3Bx1>x2>x3Cx1=0 , x3>x2Dx3>x2>x1
A block of mass m initially at rest is dropped from a height h on to a spring of force constant k . the maximum compression in the spring is x thenAmgh=12kx2Bmg(h+x)=12kx2Cmgh=12k(x+h)2Dmg(h+x)=12k(x+h)2
In the figure shown, all surfaces are smooth and force constant of spring is 10N/m. Block of mass (2 kg) is attaced with the spring. The spring is compressed by (2m) and then released. Find the maximum distance d travelled by the block over the inclined plane. Take g=10m/s2.
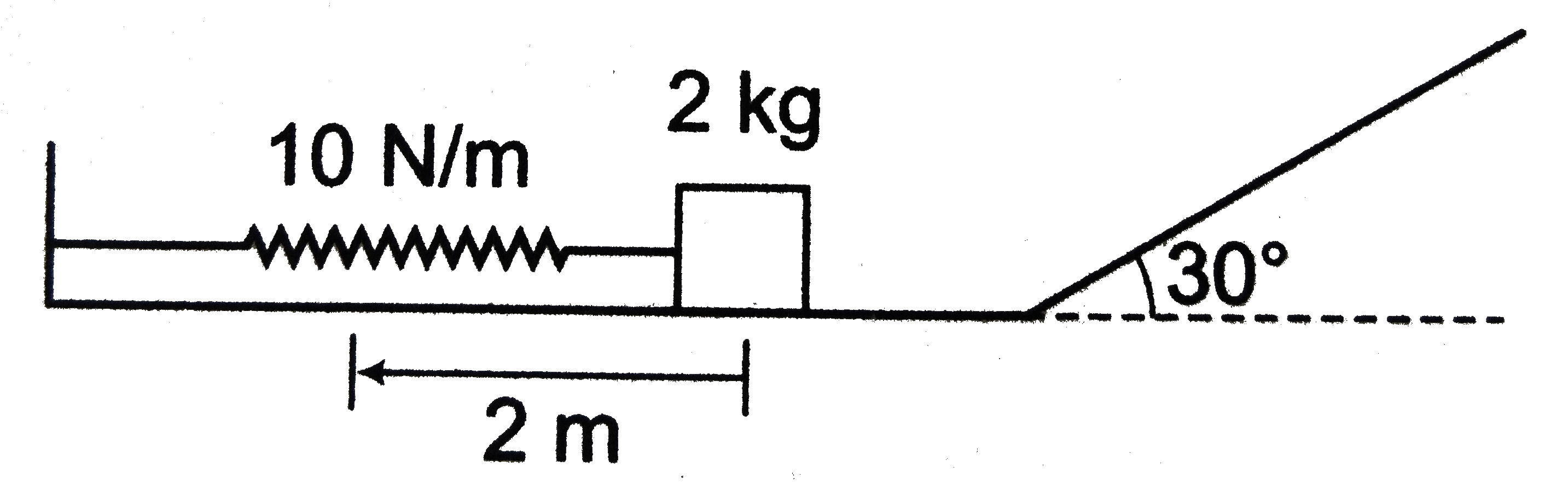 .
.
View SolutionIn the situations shown in the figure surfaces are frictionless. Find the maximum extension of the springs if blocks are initially at rest and springs are initially in natural lengths.
View SolutionTwo blocks 1 and 2 of masses m and 2m respectively are connected by a spring of force constant k. The masses are moving to the right with uniform velocity v each, the heavier mass, leading the lighter one. The spring is of natural length in the motion. Block 2 collides head on with a third block 3 of mass m, at rest, the collision being completely inelastic. Determine the velocity of blocks at the instant of maximum compression of the spring.
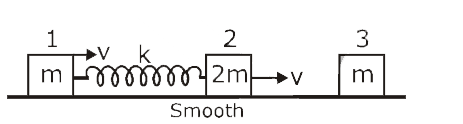
View SolutionA system of wedge and block as shown in figure, is released with the spring in its natural length. All surfaces are frictionless. Maximum elongation in the spring will be
 .
.
View SolutionA block of mass m=25kg on a smooth horizontal surface with a velocity →v=3ms−1 meets the spring of spring constant k=100N/m fixed at one end as shown in figure. The maximum compression of the spring and velocity of block as it returns to the original position respectively are.
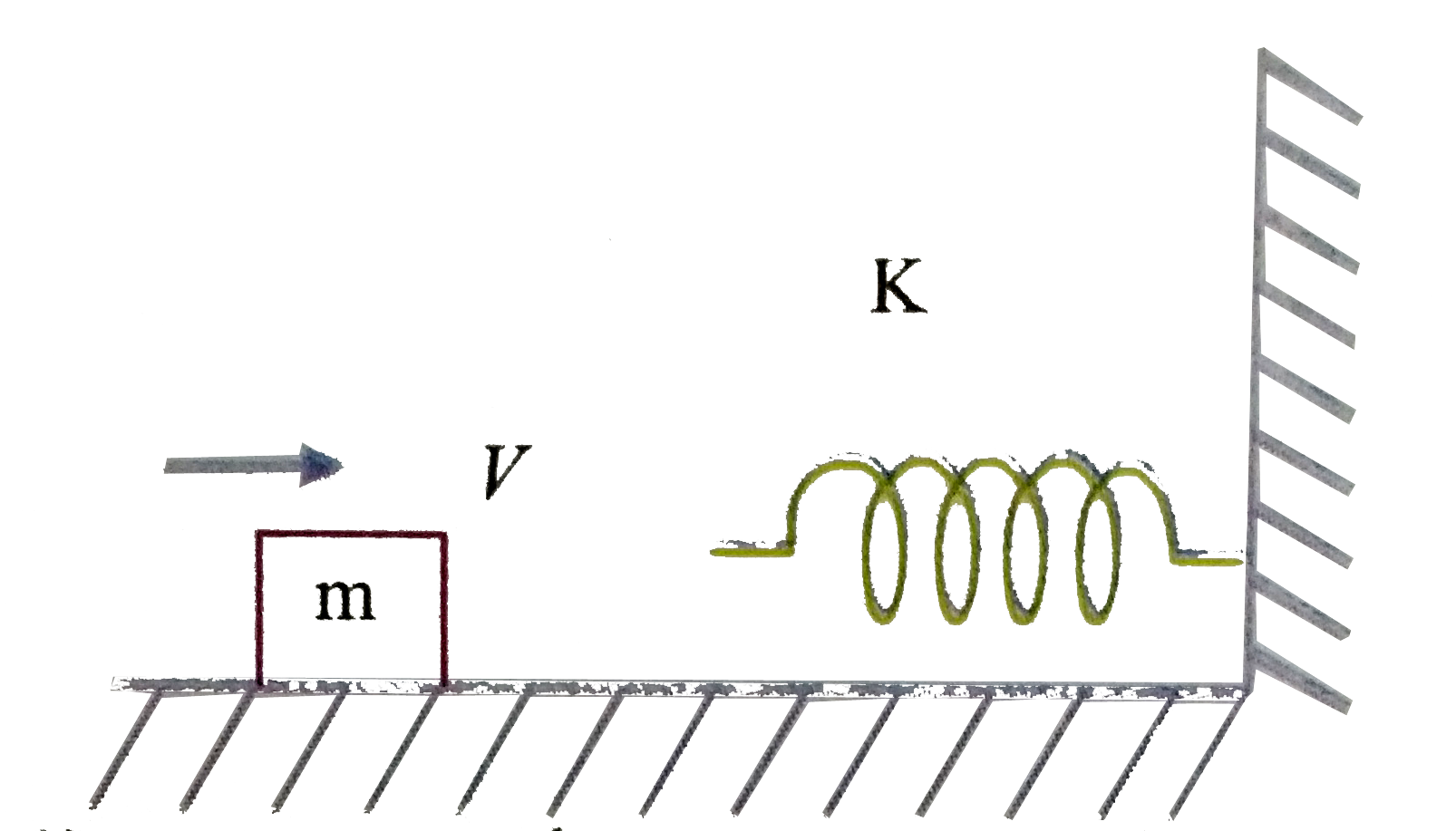 .
.
View Solution
