A point traverses half a circle of radius R during the interval τ.Calculate the following quantities averaged over that time
(a)the mean velocity that is speed ltvgt ,
(b)the modulus of the mean vector lt v gt ,
( c)the modulus of the average acceleration a if its tangential acceleration is constant.
The correct Answer is:< v > =πRτ,l<vevu>l=2Rτ,2πRτ2
Topper's Solved these Questions
Similar Questions
A point traversed 3/4 th of the circle of radius R in time t. The magnitude of the average velocity of the particle in this time interval is
View SolutionA particle A moves along a circle of radius R=50cm so that its radius vector r relative to the point O(figure) rotates with the constant angular velocity ω=0.40rad/s. Find the modulus of the velocity of the particle, and the modulus and direction of its total acceleration.

View Solution
Knowledge Check
A point traversed half a circle of radius r during a time interval t0, its mean speed and magnitude of mean velocity are
Aπrt0,rt0B2πrt0,2rt0Cπrt0,2rt0D2πrt0,rt0A point traversed 3/4 th of the circle of radius R in time t. The magnitude of the average velocity of the particle in this time interval is
AπRtB3πR2tCR√2tDR√2tA particle travels two and a half revolutions of the circle of radius R in time t. The ratio of the average speed of the particle to the magnitude of the average velocity in this time interval is
Aπ2B5π√2C5π2Dπ5√2
A particle A moves along a circle of radius R=50cm so that its radius vector r relative to the point O(figure) rotates with the constant angular velocity ω=0.40rad/s. Find the modulus of the velocity of the particle, and the modulus and direction of its total acceleration.

View SolutionA particle moves on a circle of radius r with centripetal acceleration as function of time as ac=k2rt2, where k is a positive constant. Find the following quantities as function of time at an instant :
(a) The speed of the particle
(b) The tangential acceleration of the particle
( c) The resultant acceleration, and
(d) Angle made by the resultant acceleration with tangential acceleration direction.
View SolutionA particle travels two and a half revolutions of the circle of radius R in time t. The ratio of the average speed of the particle to the magnitude of the average velocity in this time interval is
View SolutionFigur shows the total acceleration and velocity of a particle moving clockwise in a circle of radius 2.5m at a given instant of time. At this instant, find:
(a) the radius acceleration,
(b) the speed of the acceleration,
(c) its tangential acceleration.
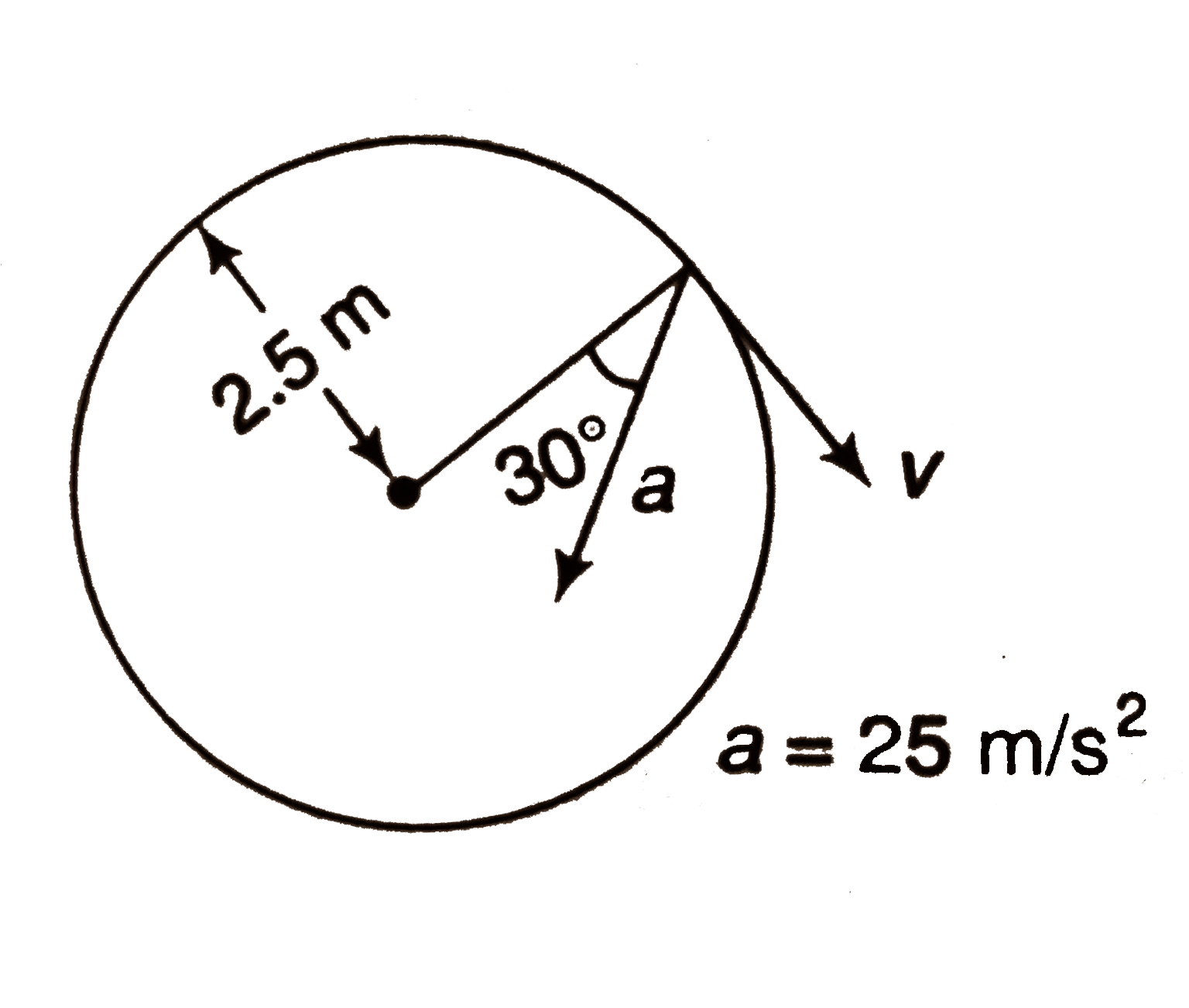
View SolutionFigur shows the total acceleration and velocity of a particle moving clockwise in a circle of radius 2.5m at a given instant of time. At this instant, find:
(a) the radius acceleration,
(b) the speed of the acceleration,
(c) its tangential acceleration.
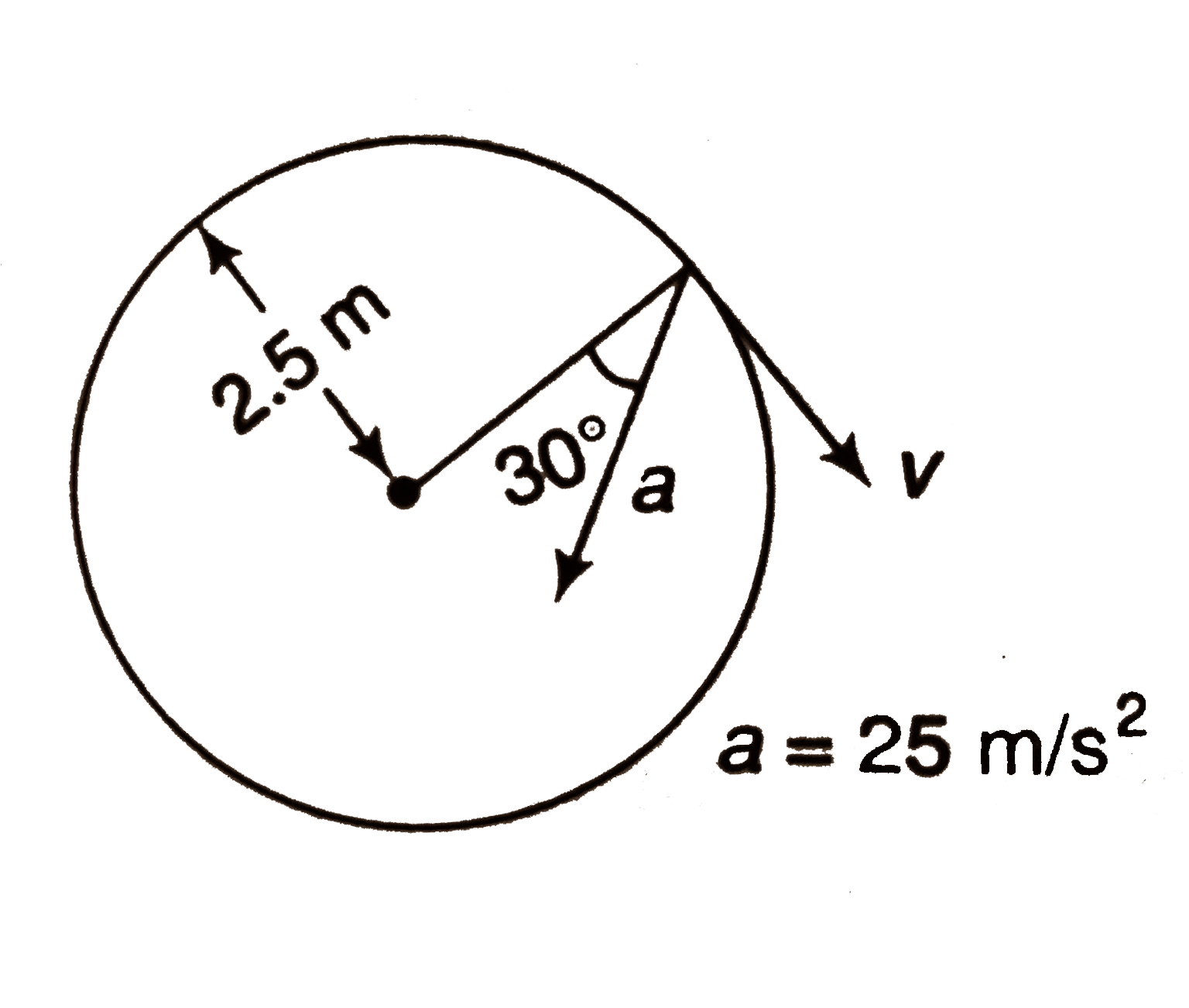
View Solution
