A particle starts from the origin, goes along the X-axis to the pont (20m, 0) and then returns along the same line to the point (-20m,0). Find the distance and displacement of the particle during the trip.
The correct Answer is:A, B, C, D
To solve the problem, we need to find both the distance and displacement of the particle during its trip. Let's break it down step by step.
Step 1: Understand the Motion of the Particle
The particle starts at the origin (0, 0) and moves to the point (20 m, 0) along the X-axis. After reaching (20 m, 0), it returns back along the same line to the point (-20 m, 0).
Step 2: Calculate the Total Distance Traveled
1. From the Origin to (20 m, 0): The particle travels 20 meters.
2. From (20 m, 0) back to the Origin (0, 0): The particle travels another 20 meters.
3. From the Origin (0, 0) to (-20 m, 0): The particle travels 20 meters again.
Now, we can sum these distances:
- Distance from (0, 0) to (20, 0) = 20 m
- Distance from (20, 0) to (0, 0) = 20 m
- Distance from (0, 0) to (-20, 0) = 20 m
Total Distance = 20 m + 20 m + 20 m = 60 m
Step 3: Calculate the Displacement
Displacement is defined as the shortest straight-line distance from the initial position to the final position, along with its direction.
1. Initial Position: (0, 0)
2. Final Position: (-20, 0)
To find the displacement:
- The displacement vector can be calculated as:
Displacement=Final Position−Initial Position=(−20,0)−(0,0)=(−20,0)
The magnitude of the displacement is 20 meters, and since the final position is to the left of the origin, the direction is in the negative X direction.
Final Answers
- Total Distance Traveled: 60 meters
- Displacement: 20 meters in the negative X direction
The particle starts at the origin (0, 0) and moves to the point (20 m, 0) along the X-axis. After reaching (20 m, 0), it returns back along the same line to the point (-20 m, 0).
1. From the Origin to (20 m, 0): The particle travels 20 meters.
2. From (20 m, 0) back to the Origin (0, 0): The particle travels another 20 meters.
3. From the Origin (0, 0) to (-20 m, 0): The particle travels 20 meters again.
- Distance from (0, 0) to (20, 0) = 20 m
- Distance from (20, 0) to (0, 0) = 20 m
- Distance from (0, 0) to (-20, 0) = 20 m
Displacement is defined as the shortest straight-line distance from the initial position to the final position, along with its direction.
2. Final Position: (-20, 0)
- The displacement vector can be calculated as:
Displacement=Final Position−Initial Position=(−20,0)−(0,0)=(−20,0)
- Total Distance Traveled: 60 meters
- Displacement: 20 meters in the negative X direction
Topper's Solved these Questions
- REST AND MOTION : KINEMATICSBOOK - HC VERMACHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Objective 2
10 Videos
- PHYSICS AND MATHEMATICSBOOK - HC VERMACHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Exercises
34 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Exercises
86 Videos
Similar Questions
A particle starts from the origin,goes along the "X" - axis to the point(20m,0) and then returns along the same line to the point (-20m,0 then the displacement of the particle during the trip in negative direction is....(in m )
View Solution(The v−t graph) A particle moving in x-axis is given. Find the distance and displacement of the particle during two seconds from starting.
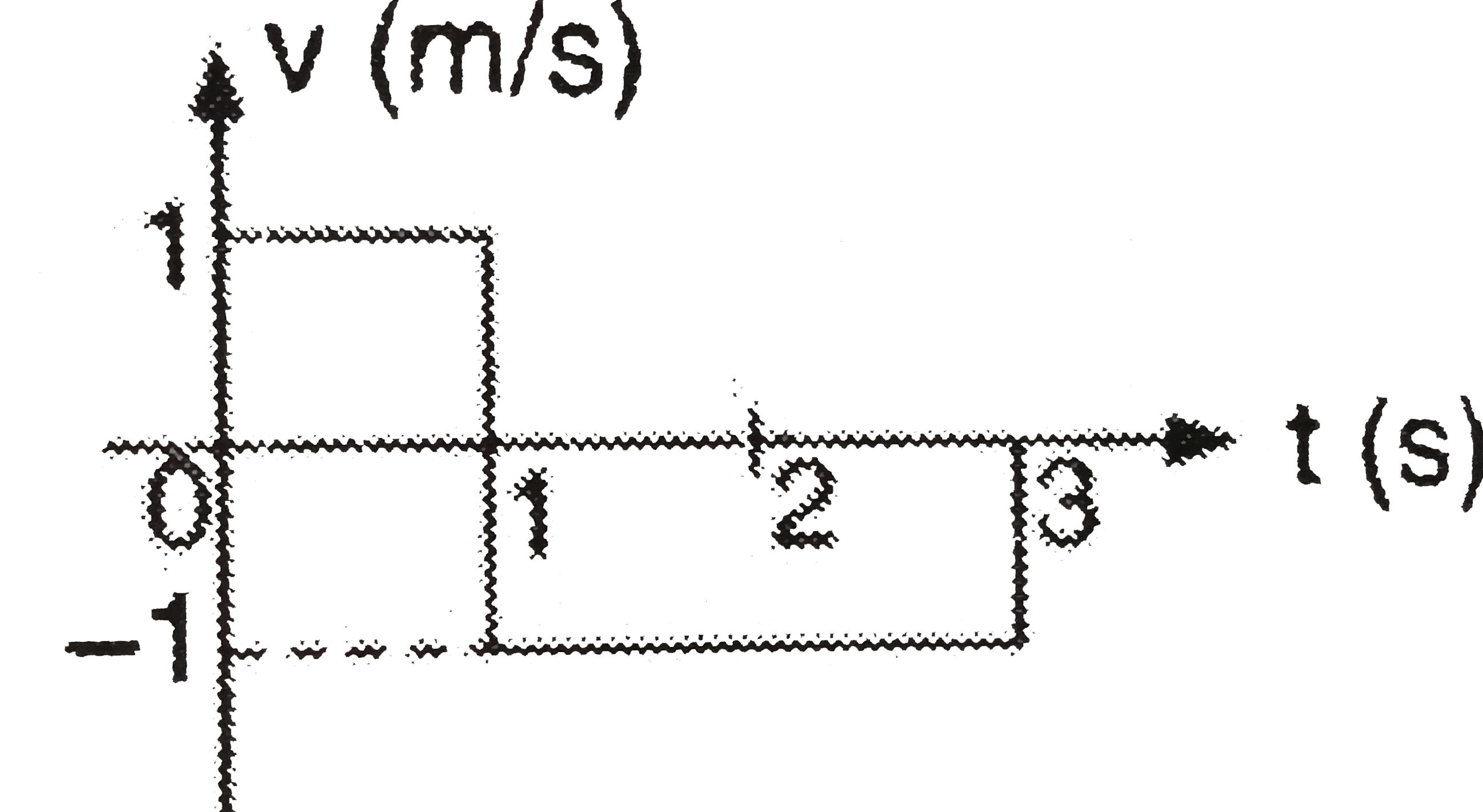
View Solution
Knowledge Check
A particle starts from the origin, goes along x-axis to the point (10 m, 0) and then returns along. The same line to the point (-10 m, 0). The distance and displacement of the particle during the trip are
A20 m, 0B30 m, 10 mC30 m, - 10 mD20 m, 10 mA particle starts from the origin, goes along X-axis to the point (30m, 0) and then returns along the same line to the point (-30m,0). The displacement and distance of the particle during the trip are
A0, 60mB60m,30mC90m, -30mD−30m,90m
A force →F=(4ˆi+5ˆj)N acts on a particle moving in XY plane. Starting from origin, the particle first goes along x-axis to the point (5.0) m and then parallel to the Y-axis to the point (5,4 m. The total work done by the force on the particle is
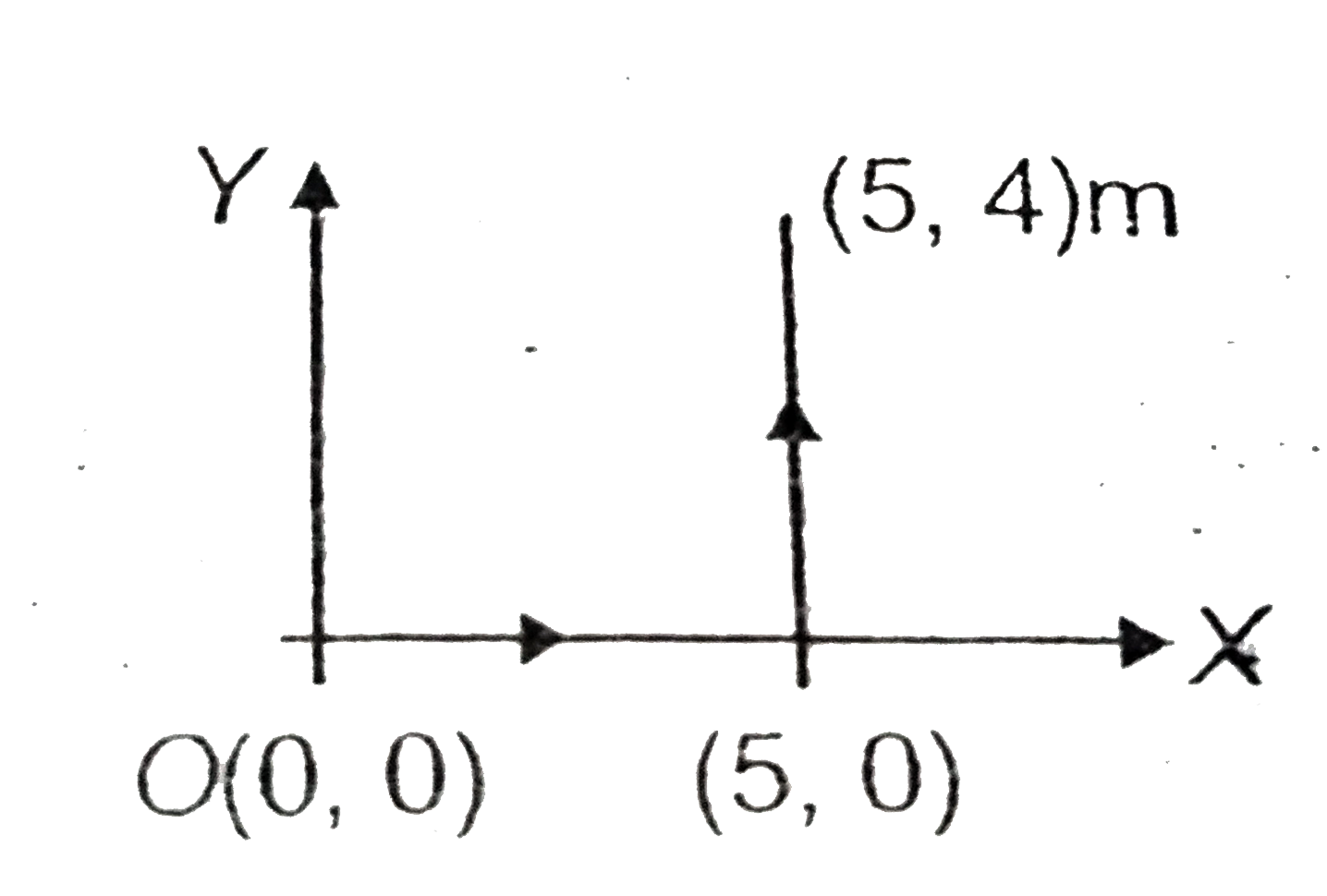
View SolutionA particle start origin moves towares north with 50m then move towards east with 40m and finaly move towards south with 20m. Find distance and displacement.
View SolutionA particle starts from the origin and moves along a stright line If the velocity of the particle at time t seconds 10t cm/s then find the distance traversed during fist seconds after the start.
View SolutionA particle is moving along the x-axis such that s=6t−t2, where s in meters and t is in second. Find the displacement and distance traveled by the particle during time interval t=0 to t=5s.
View SolutionA particle starts at the origin and moves along the x-axis in such a way that its velocity at the point (x, 0) is given by the formula dxdt=cos2πx. Then the particle never reaches the point on
View SolutionThree particles start from the origin at the same time, one with velocity u1 along the x-axis, the second with velocity u2 along the y-axis . Find the velocity of the third particles, along the x=y line so that the three particles may always lie on the same straight line.
View Solution
