A homogeneous chain of length 2l and mass M lies on an absolutely smooth table. A small part of the chain hangs from the table. At the initial moment, the part of the chain lying on the table is held and released, after which the chain begins to slide off the table under the weight of the hanging end. Find the velocity of the chain when the length of the hanging part is equal to x(x<I). Also calculate the acceleration and the force with which it acts on the edge of the table.
The correct Answer is:[x√g2l,xg2l,√2Mg4l2(2l−x)x]
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Advance MCQs
17 Videos
- LINEAR MOMENTUM & ITS CONSERVATIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - LINEAR MOMENTUM & ITS CONSERVATIONEXERCISE - exercise 1.3
3 Videos
- RIGID BODIES AND ROTATIONAL MOTIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - RIGID BODIES AND ROTATIONAL MOTIONEXERCISE - Unsolved Numerical
63 Videos
Similar Questions
A uniform chain of length l and mass m lies on a smooth table. A very small part of this chain hangs from the table. It begins to fall under the weight of hanging part. Find the velocity of chain when the length of hanging part becomes y.
View SolutionA uniform chain of length l and mass m overhangs a smooth table with its two third part lying on the table. Find the kinetic energy of the chain as it completely slips off the table.
View Solution
Knowledge Check
One fourth chain is hanging down from a table work done to bring the hanging part of the chain on the table is ( mass of chain =M and length = L)
AMgL32BMgL16CMgL8DMgL4A uniform chain of mass m & length L is kept on a smooth horizontal table such that Lnportion of the chaing hangs from the table. The work dione required to slowly bringsthe chain completely on the table is
AmgLnBmgL2CmgLn2DmgL2n2
A uniform chain of length l and mass m overhangs a smooth table with its tow third pat lying on the tabl. Find the kinetic energy of the chain s it completely slips off the table.
View SolutionA chain AB of length l is located on a smooth horizontal table so that its fraction of length h hangs freely with end B on the table. At a certain moment, the end A of the chain is set free. With what velocity with this end of the chain slip off the table?

View SolutionOne fourth chain is hanging down from a table work done to bring the hanging part of the chain on the table is ( mass of chain =M and length = L)
View SolutionA uniform chain of mass m & length L is kept on a smooth horizontal table such that Lnportion of the chaing hangs from the table. The work dione required to slowly bringsthe chain completely on the table is
View SolutionA chain of length L and mass M is held on a frictionless table with (1/n)th of its length hanging over the edge. When the chain is released, find the velocity of chain while leaving the table.
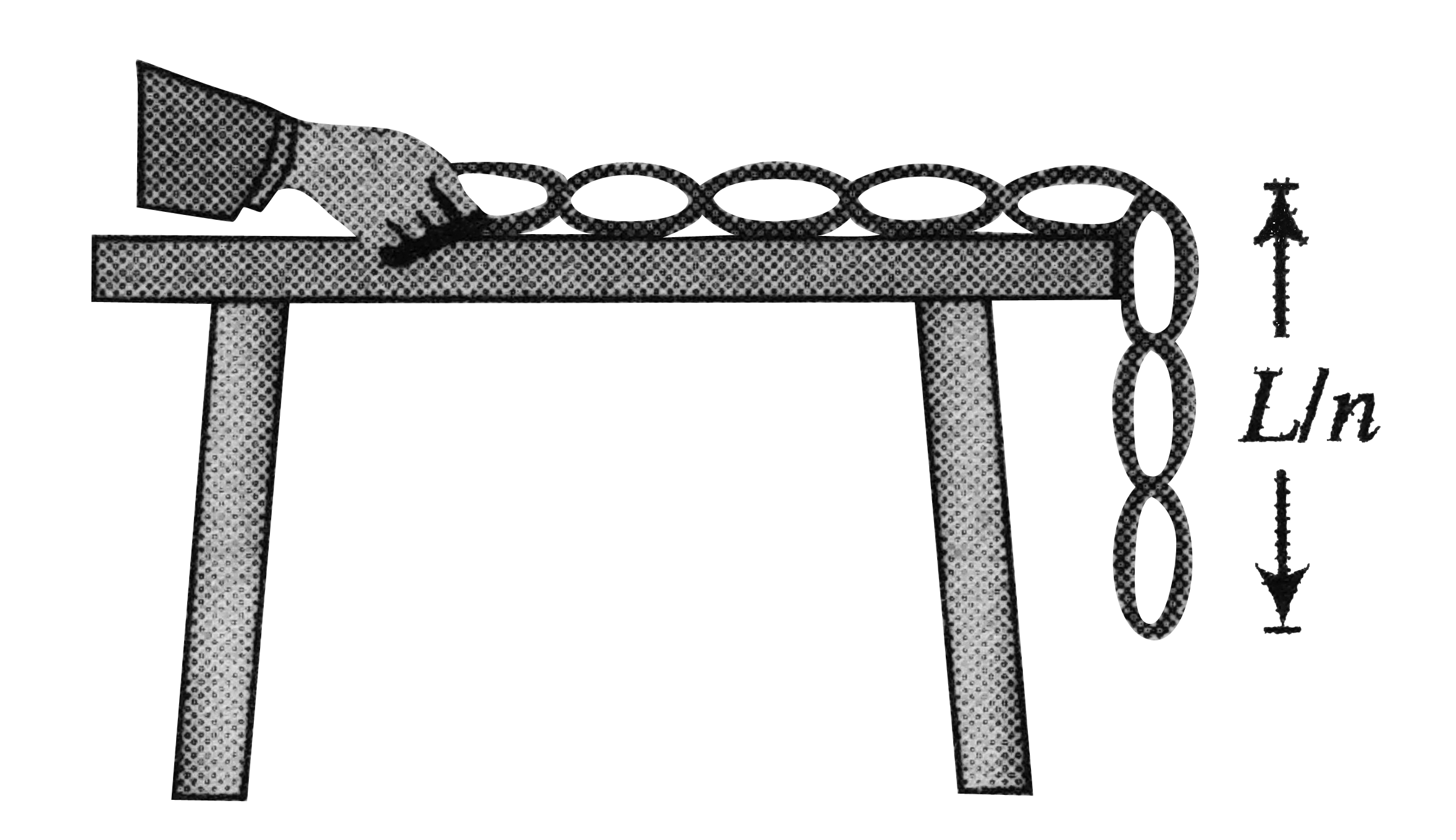
View SolutionA chain of length L and mass M is held on a frictionless table with (1/n)th of its length hanging over the edge. When the chain is released, find the velocity of chain while leaving the table.

View Solution
