One fourth chain is hanging down from a table work done to bring the hanging part of the chain on the table is ( mass of chain M and length L)
The correct Answer is:A
To solve the problem of calculating the work done to bring the hanging part of the chain onto the table, we can follow these steps:
1. Identify the parameters:
- The total mass of the chain is M.
- The total length of the chain is L.
- The length of the chain hanging down from the table is L4.
2. Determine the mass of the hanging part:
- The mass per unit length of the chain is given by ML.
- Therefore, the mass of the hanging part (length L4) is:
mhanging=ML×L4=M4
3. Calculate the force acting on the hanging part:
- The weight of the hanging part, which is the force acting downwards, is:
F=mhanging⋅g=M4⋅g
4. Set up the work done calculation:
- The work done to lift the hanging part of the chain can be calculated using the integral of force over distance. The distance the center of mass of the hanging part moves when lifted onto the table is L8 (since the center of mass of the hanging part is at L8 from the table).
- The work done W is given by:
W=F⋅d=(M4⋅g)⋅(L8)
5. Calculate the work done:
- Substituting the values into the equation:
W=M4⋅g⋅L8=M⋅g⋅L32
6. Final result:
- The work done to bring the hanging part of the chain onto the table is:
W=M⋅g⋅L32
Conclusion:
The work done to bring the hanging part of the chain onto the table is M⋅g⋅L32.
- The total mass of the chain is M.
- The total length of the chain is L.
- The length of the chain hanging down from the table is L4.
- The mass per unit length of the chain is given by ML.
- Therefore, the mass of the hanging part (length L4) is:
mhanging=ML×L4=M4
- The weight of the hanging part, which is the force acting downwards, is:
F=mhanging⋅g=M4⋅g
- The work done to lift the hanging part of the chain can be calculated using the integral of force over distance. The distance the center of mass of the hanging part moves when lifted onto the table is L8 (since the center of mass of the hanging part is at L8 from the table).
- The work done W is given by:
W=F⋅d=(M4⋅g)⋅(L8)
- Substituting the values into the equation:
W=M4⋅g⋅L8=M⋅g⋅L32
- The work done to bring the hanging part of the chain onto the table is:
W=M⋅g⋅L32
The work done to bring the hanging part of the chain onto the table is M⋅g⋅L32.
Similar Questions
A uniform chain of mass m and length l is lying on a horizontal table with one-third of its length hanging over the edge of the table.

What is the speed of the chain, when it just loses contact with the table?
View SolutionA uniform chain of mass m and length l is lying on a horizontal table with one-third of its length hanging over the edge of the table.

If the chain is limiting equlibrium, what is the coefficient of friction for the contact between table and chain?
View SolutionA uniform chain of mass m and length l is lying on a horizontal table with one-third of its length hanging over the edge of the table.

If a gentle push is given to hanging end, it will start moving down. What is the change in gravitational potential energy of the chain, as it just leaves the table?
View SolutionA homogoneous chain of length L lies on a table. The coefficient of friction between the chain and the table is μ. The maximum length which can hang over the table in equilibrium is
View SolutionA uniform chain of length L and mass M overhangs a horizontal table with its two third part on the table. The friction coefficient between the table and the chain is µ. The work done by the friction during the period the chain slips off the table is [−2kμMgL]. Find the value of k.
View SolutionA chain is held on a frictionless table with 1/n th of its length hanging over the edge. If the chain has a length L and a mass M, how much work is required to pull the hanging part back on the table?
View SolutionA man places a chain (of mass m and length l) on a table slowly. Initially, the lower end of the chain just touches the table. The main brings down the chain by length l/2. Work done by the man in this process is
View SolutionA uniform chain of mass m and length l is at the verge of sliding under the effect of gravity of the hanging part. Find the
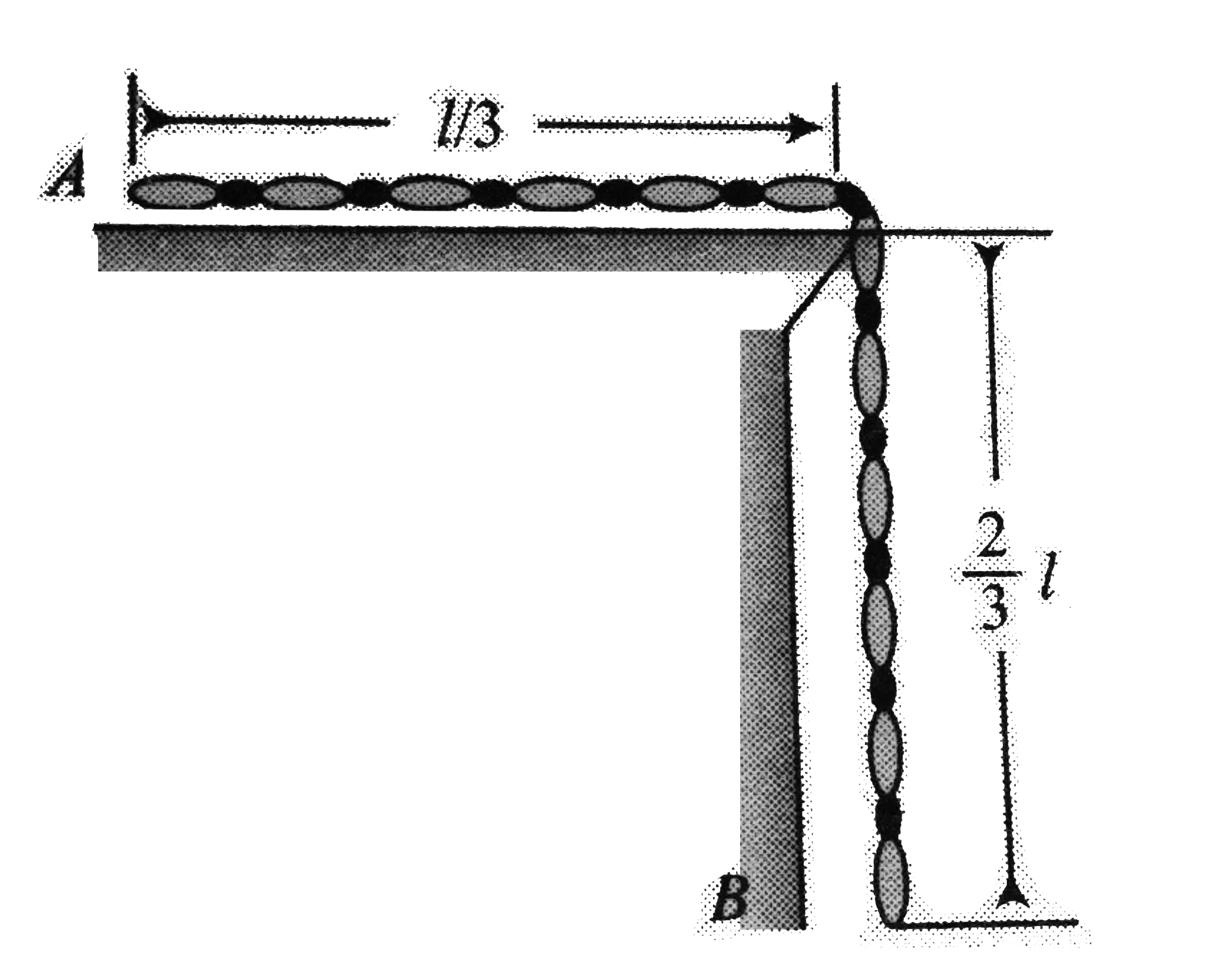
a. coefficient of friction, between chain and table.
b. work done by friction and gravity till the chain leaves the table if the hanging part is pulled gently and released.
c. speed of the chain at the time of leaving the table in part (b) .
d. work done by the external force acting at the end A of the chain in slowly pulling the chain completely onto the table.
View SolutionA chain is lying on a rough table with a fraction 1/n of its length hanging down from the edge of the table. if it is just on the point of sliding down from the table, then the coefficient of friction between the table and the chain is -
View SolutionWhat will be the work done by external agent to slowly hang the lower end of the chain to the peg?
View Solution
