A particle starts moving from rest at with a tangential acceleration of constant magnitude of along a circle of radius 6 m. The value of average acceleration, average velocity and average speed during the first s of motion, are respectively :
None of the above
The correct Answer is:B
To solve the problem, we need to find the average acceleration, average velocity, and average speed of a particle moving in circular motion under constant tangential acceleration. Here are the steps to arrive at the solution:
Step 1: Calculate Average Acceleration
1. Given Data:
- Tangential acceleration at=πm/s2
- Time t=2√3s
- Initial velocity u=0 (starts from rest)
2. Final Velocity Calculation:
Using the formula for final velocity under constant acceleration:
v=u+att
Substituting the values:
v=0+π⋅(2√3)=2π√3m/s
3. Average Acceleration Calculation:
Average acceleration aavg is defined as the change in velocity over time:
aavg=ΔvΔt=v−ut=2π√3−02√3=πm/s2
Step 2: Calculate Average Velocity
1. Angular Displacement Calculation:
The angular acceleration α is given by:
α=atr=π6rad/s2
The angular displacement θ can be calculated using:
θ=12αt2=12⋅π6⋅(2√3)2=12⋅π6⋅12=πrad
2. Linear Displacement Calculation:
The linear displacement s for a circular path can be calculated as:
s=rθ=6⋅π=6πm
3. Average Velocity Calculation:
Average velocity vavg is defined as total displacement divided by total time:
vavg=st=6π2√3=3π√3=√3πm/s
Step 3: Calculate Average Speed
1. Total Distance Calculation:
The total distance traveled during the time t can be calculated using:
d=12att2=12⋅π⋅(2√3)2=12⋅π⋅12=6πm
2. Average Speed Calculation:
Average speed is defined as total distance divided by total time:
vavg,speed=dt=6π2√3=√3πm/s
Final Results
- Average acceleration: πm/s2
- Average velocity: √3πm/s
- Average speed: √3πm/s
- Tangential acceleration at=πm/s2
- Time t=2√3s
- Initial velocity u=0 (starts from rest)
Using the formula for final velocity under constant acceleration:
v=u+att
Substituting the values:
v=0+π⋅(2√3)=2π√3m/s
Average acceleration aavg is defined as the change in velocity over time:
aavg=ΔvΔt=v−ut=2π√3−02√3=πm/s2
The angular acceleration α is given by:
α=atr=π6rad/s2
The angular displacement θ can be calculated using:
θ=12αt2=12⋅π6⋅(2√3)2=12⋅π6⋅12=πrad
The linear displacement s for a circular path can be calculated as:
s=rθ=6⋅π=6πm
Average velocity vavg is defined as total displacement divided by total time:
vavg=st=6π2√3=3π√3=√3πm/s
The total distance traveled during the time t can be calculated using:
d=12att2=12⋅π⋅(2√3)2=12⋅π⋅12=6πm
Average speed is defined as total distance divided by total time:
vavg,speed=dt=6π2√3=√3πm/s
- Average velocity: √3πm/s
- Average speed: √3πm/s
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - JEE Advanced25videos
CIRCULAR MOTION
- BOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - More than One Option is Correct13videos
CIRCULAR MOTION
- BOOK - DC PANDEYCHAPTER - CIRCULAR MOTIONEXERCISE - Subjective Questions2videos
CIRCULAR MOTION
- BOOK - DC PANDEYCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective21videos
CENTRE OF MASS, LINEAR MOMENTUM AND COLLISION
- BOOK - DC PANDEYCHAPTER - COMMUNICATION SYSTEMEXERCISE - Only One Option is Correct27videos
COMMUNICATION SYSTEM
Similar Questions
A particle starts from rest and moves with an acceleration of a={2+|t−2|}m/s2 The velocity of the particle at t=4 sec is
View SolutionA particle starting from rest has a constant acceleration of 4m/s2 for 4 s. It then retards uniformly for next 8 s and comes to rest. Find during the motion of particle (a) average acceleration (b) average speed and (c) average velocity.
View Solution
Knowledge Check
- Question 1 - Select One
A particle starts from rest with constant acceleration. The ratio of space-average velocity to the time average velocity is :-
A1/2B3/4C4/3D3/2 - Question 2 - Select One
A car moving on a straight road accelerated from a speed of 4.1m/s to a speed of 6.9m/s in 5.0 s. Then its average acceleration is
A0.5m/s2B0.6m/s2C0.56m/s2D0.65m/s2 - Question 3 - Select One
A particle moves in a circle with constant tangential acceleration starting from rest. At t = 1/2 s radial acceleration has a value 25% of tangential acceleration. The value of tangential acceleration will be correctly represented by
Aat=RBat=R/2Cat=2RDnone of these
A particle starts from rest and moves with a constant acceleration along a straight path to attain 64 m s−1 in t s. If at t = (t + 3)s the speed is 76 ms−1, then find the acceleration of the particle and 't'.
View SolutionA particle starting from rest moves with constant acceleration. If it takes 5.0 s to reash the speed 18.0 km/h find a. the average velocity during this period, and b. the distance travelled by the particle during this period.
View SolutionA particle is moving with a uniform acceleration 4ms−2 for time 2 second and then 5ms−20 for time 3 seconds. What is the average acceleration of particle during motion.
View SolutionA particle starts moving along a circle of radius (20/π)m with constant tangential acceleration. If the velocity of the parthcle is 50m/s at the end of the second revolution after motion has began, the tangential acceleration in m/s2 is :
View SolutionAcceleration time graph of a particle moving along a straight line is given. Then average acceleration between t=0 & t=4 is :−
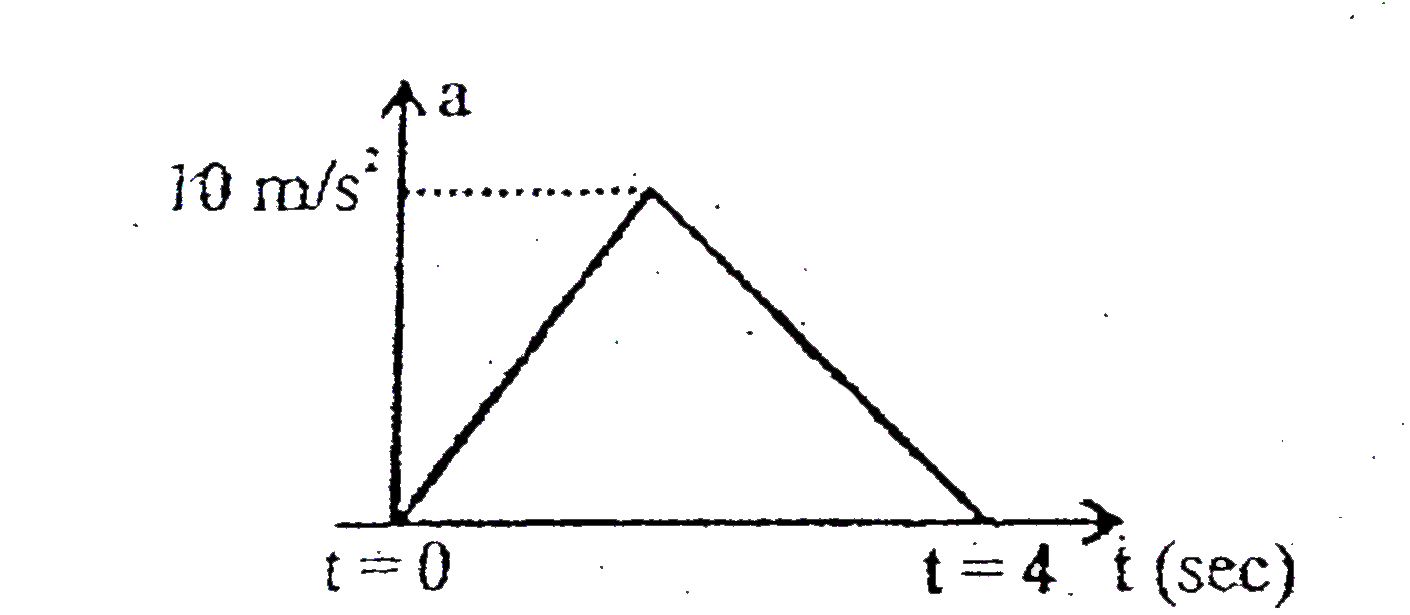 View Solution
View Solution

