There are two point masses A and B, situated at origin and point (5m,0m) respectively . At a certain time vA and vB are respectively the velocities of point masses A and B . Match the situations under Table-1 with their correct option under Table-2
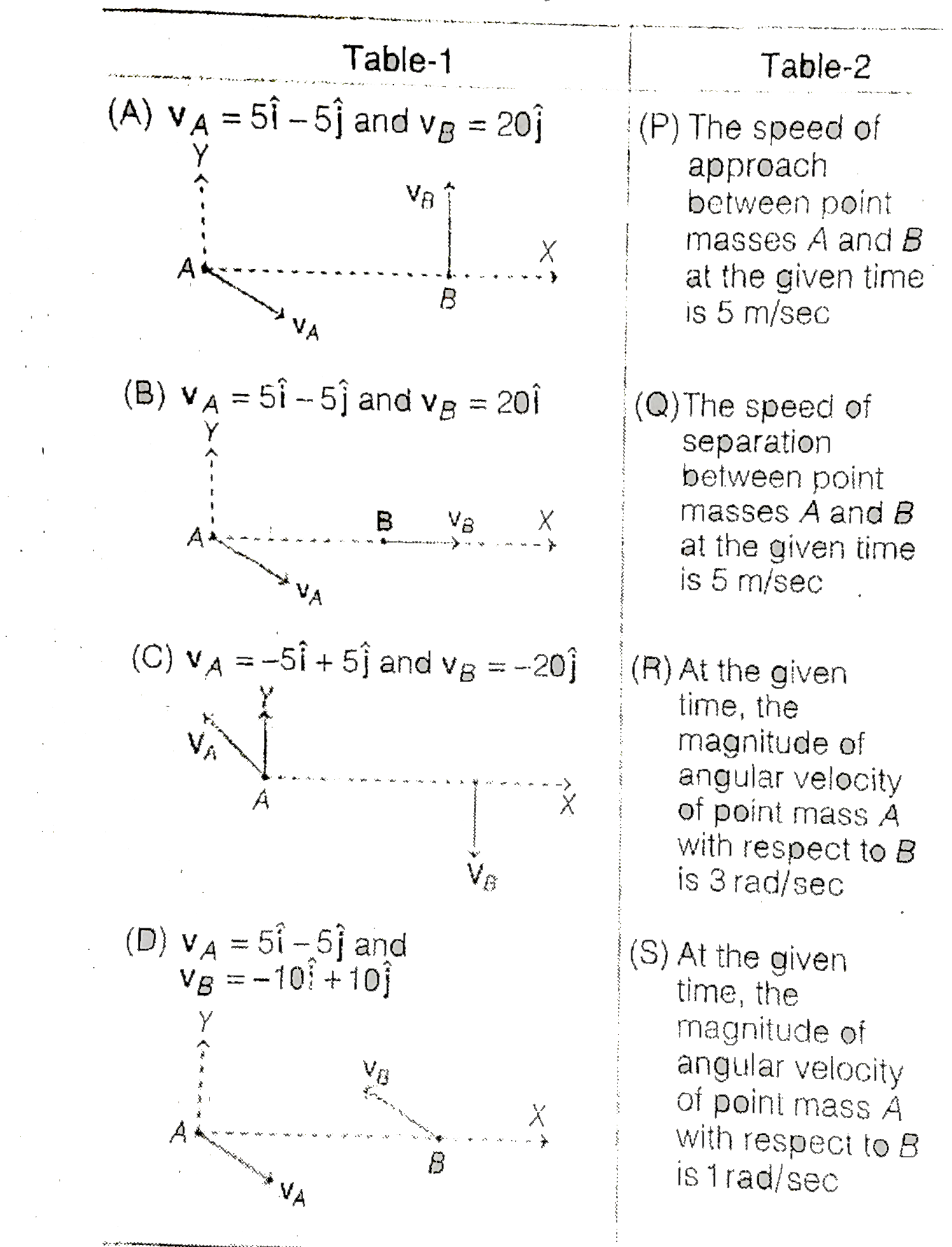
The correct Answer is:(A)P,(B)S,(C)Q,(D)R
Topper's Solved these Questions
- ROTATIONAL MOTIONBOOK - DC PANDEYCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions
17 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEYCHAPTER - ROTATIONAL MOTIONEXERCISE - Comprehension Type Questions
41 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEYCHAPTER - ROTATIONAL MECHANICSEXERCISE - Subjective Questions
2 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEYCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
Similar Questions
Two point charge −q and +q/2 are situated at the origin and the point (a,0,0) respectively. The point along the X-axis where the electic field Vanishes is
View SolutionIn each situation of Table-1, a uniform disc of mass m and radius R rolls on a rough fixed horizontal surface as shown. At, t=0 (initially) the angular velocity of disc is ω0 and velocity of centre of mass of disc is v0 (in horizontal direction). The relation between v0 and ω0 for each situation and also initial sense of rotation is given in Table-1 . Then match the statements in Table-1 with the corresponding results in Table-2.
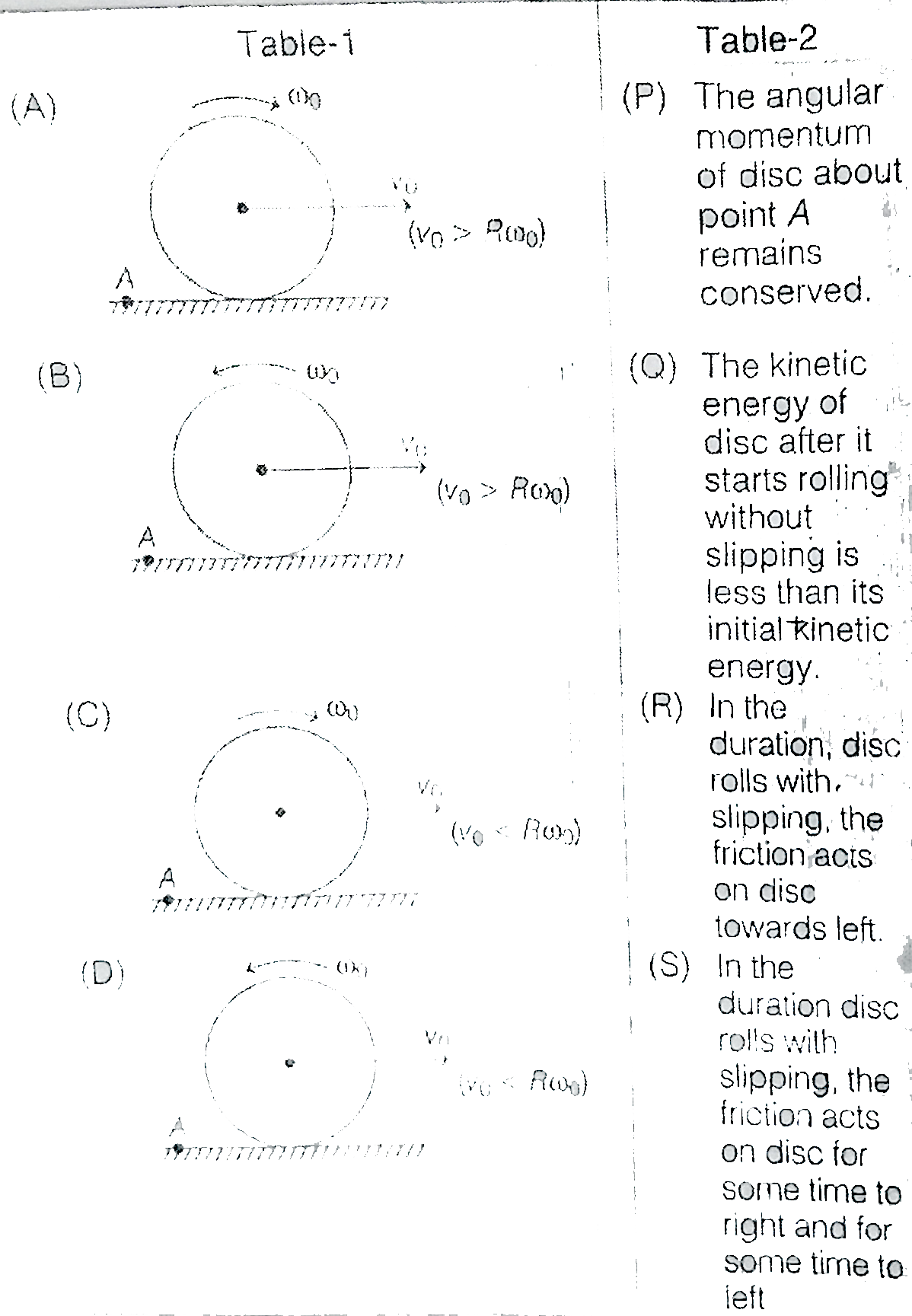
View Solution
Knowledge Check
Two point charges −q and +q/2 are situated at the origin and the point (a,0,0) respectively. The point along the X-axis where the electric field Vanishes is
Ax=a√2Bx=√2aCx=√2a√2−1Dx=√2a√2+1Two particles AandB of masses mAandmB respectively and having the same charge are moving in a plane . The speeds of the particles are vAandvB respectively and the trajectories are as shown in the figure. Then
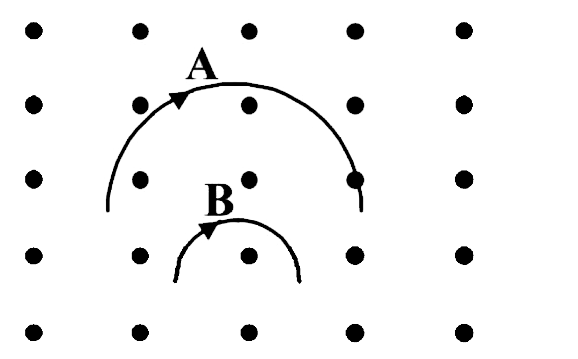 AmAvA<mBvBBmAvA>mBvBCmAvA<mBandvA<vBDmA=mBandva=vB
AmAvA<mBvBBmAvA>mBvBCmAvA<mBandvA<vBDmA=mBandva=vBTwo points masses of 2 kg and 3 kg lie at 5 m and 9 m away from origin O respectively. Find out the distance of centre of mass of these point masses from the origin.
A14.2 mB7.4 mC20 mD6 m
In each situation of Table-1, a uniform disc of mass m and radius R rolls on a rough fixed horizontal surface as shown. At, t=0 (initially) the angular velocity of disc is ω0 and velocity of centre of mass of disc is v0 (in horizontal direction). The relation between v0 and ω0 for each situation and also initial sense of rotation is given in Table-1 . Then match the statements in Table-1 with the corresponding results in Table-2.

View Solutiontwo point charges 2μC and −4μC are situated at points (−2m,0m) and (2m,0m) respectively. Find out potential at point C(4m,0m) and D(0m,√5m).

View Solutiontwo point charges 2μC and −4μC are situated at points (−2m,0m) and (2m,0m) respectively. Find out potential at point C(4m,0m) and D(0m,√5m).

View SolutionTwo particles of mass mA and mB and their velocities are VA and VB respectively collides. After collision they interchanges their velocities, then ratio of mAmB is
View SolutionTwo particles of mass mA and mB and their velocities are VA and VB respectively collides. After collision they interchanges their velocities, then ratio of mAmB is
View Solution
