The motion of the centre of mass is the result of
Attractive forces
Repulsive forces
External forces
Internal forces
The correct Answer is:C
Step by step video, text & image solution for The motion of the centre of mass is the result of by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
Similar Questions
Separation of Motion of a system of particles into motion of the centre of mass and motion about the centre of mass :
(a) Show P=Pi+miV
where pi is the momentum of the ith particle (of mass mi) and pi=mivt Notevi is the velocity of the ith particle relative to the centre of mass.
Also, prove using the definition of the centre of mass ∑pt=0
(b) Show K=K'+1/2MV2
where K is the total kinetic energy of the system of particles. K' is the total kinetic energy of the system when the particle velocities are taken with respect to the centre of mass and MV2/2 is the kinetic energy of the translation of the system as a whole (i.e. of the centre of mass motion of the system). The result has been used in Sec. 7.14.
(c ) Show L=L'+R×MV
where L'=∑r't×p't is the angular momentum of the system about the centre of mass with velocities taken relative to the centre of mass. Remember r'i=ri−R, rest of the notation is the standard notation used in the chapter. Note L' and MR×V can be said to be angular momenta, respectively, about and of the centre of mass of the system of particles.
(d) Show dL'dt=∑r'i×dp'dt
Further, show that
dL'dt=τ'ext
where τext is the sum of all external torques acting on the system about the centre of mass.
(Hint : Use the definition of centre of mass and third law of motion. Assume the internal forces between any two particles act along the line joining the particles.)View SolutionWrite an expression for the position vector of the centre of mass of n-particle system. Also write the equations of motion which govern the motion of the centre of mass
View Solution
Knowledge Check
- Question 1 - Select One
The motion of the centre of mass of a system of two particles is unaffected by their internal forces.
Airrespective of the actual direction of the internal forces.Bonly if they are along the line joining the particlesConly if they are at right angles to the joining the particlesDonly if they are obliquely inclined to the line joining the particle. - Question 2 - Select One
The position of the centre of mass of a cube of uniform mass density will be at
Athe centre of one faceBthe centre of the interaction of diagonals of one face.Cthe geometric centre of the cubeDthe edge of a cube - Question 3 - Select One
Centre of mass is point
Awhich is the orign of refernece frameBwhich is the geometric centre of a bodyCwhere the whole mass of the body is supposed to be centredDfrom which distances of all particles are the same
To determine the motion of the centre of mass of a system, knowledge of internal forces of the system is required.
For this purpose we need not to know the external forces on the system.View SolutionThe motion of centre of mass depends upon the total
View SolutionList-I shows some arrangements in which motion of masses are described and list-II defines motion of centre of mass of the system (m + M). Match appropriate possible options in list-II
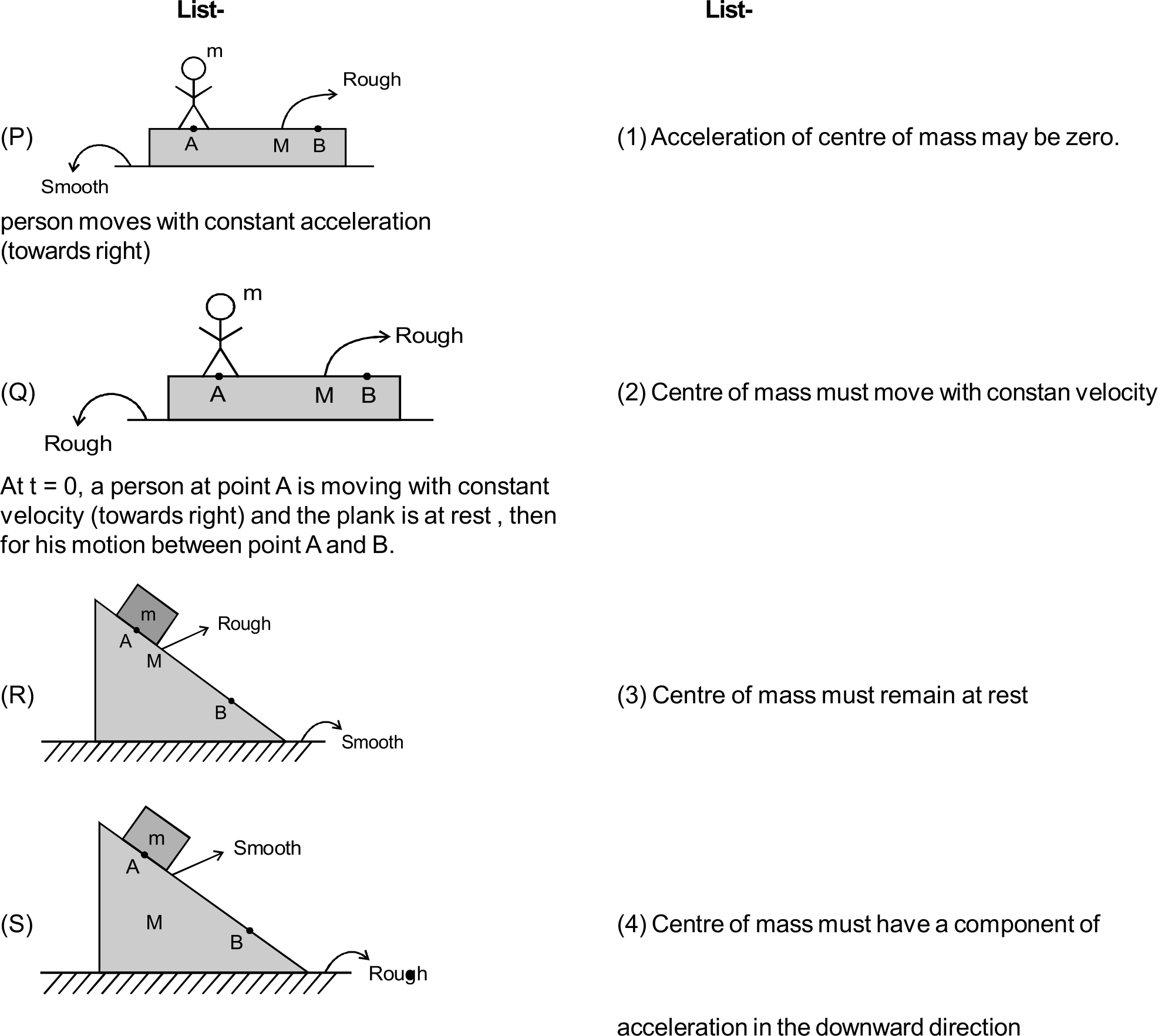 View Solution
View SolutionThe rate of change of angular momentum of a system of particles about the centre of mass is equal to the sum of external torque about the centre of mass when the centre of mass is :
View SolutionThe position of centre of mass does not depend upon the reference frame.
Centre of mass depends only upon the mass of the body.View Solution

