Two trains A and B are approaching each other on a straight track, the former with a uniform velocity of 25 m/s and other with 15m/s, when they are 225 m a part brakes are simultaneously applied to both of them. The deceleration given by the brakes to thetrain B increases linearly with time by 0.3m/s2 every second, while the train A is given a uniform deceleration, (a) What must be the minimum deceleration of the train A so that the trains do not collide ? (b) What is the time taken by the trains to come to stop ?
5m/s2
2.5m/s2
1.5m/s2
7.5m/s2
The correct Answer is:B
Step by step video, text & image solution for Two trains A and B are approaching each other on a straight track, the former with a uniform velocity of 25 m/s and other with 15m/s, when they are 225 m a part brakes are simultaneously applied to both of them. The deceleration given by the brakes to thetrain B increases linearly with time by 0.3 m//s^(2) every second, while the train A is given a uniform deceleration, (a) What must be the minimum deceleration of the train A so that the trains do not collide ? (b) What is the time taken by the trains to come to stop ? by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- KINEMATICS 1BOOK - DC PANDEYCHAPTER - KINEMATICS 1EXERCISE - MATCH THE COLUMN
10 Videos
- KINEMATICS 1BOOK - DC PANDEYCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
- KINEMATICS 1BOOK - DC PANDEYCHAPTER - KINEMATICS 1EXERCISE - MCQ_TYPE
27 Videos
- KINEMATICSBOOK - DC PANDEYCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
11 Videos
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Medical entrances gallery
39 Videos
Similar Questions
two particles A and B are at separation of 100m. They move towards each other with uniform speed, A with 5m/s and B with 15m/s. When and where the two particle meet?
View SolutionTwo identical balls are approaching towards each other on a straight line with velocity 2m/s and 4m/s, respectiely. Find the final velocities after elastic collision between them.
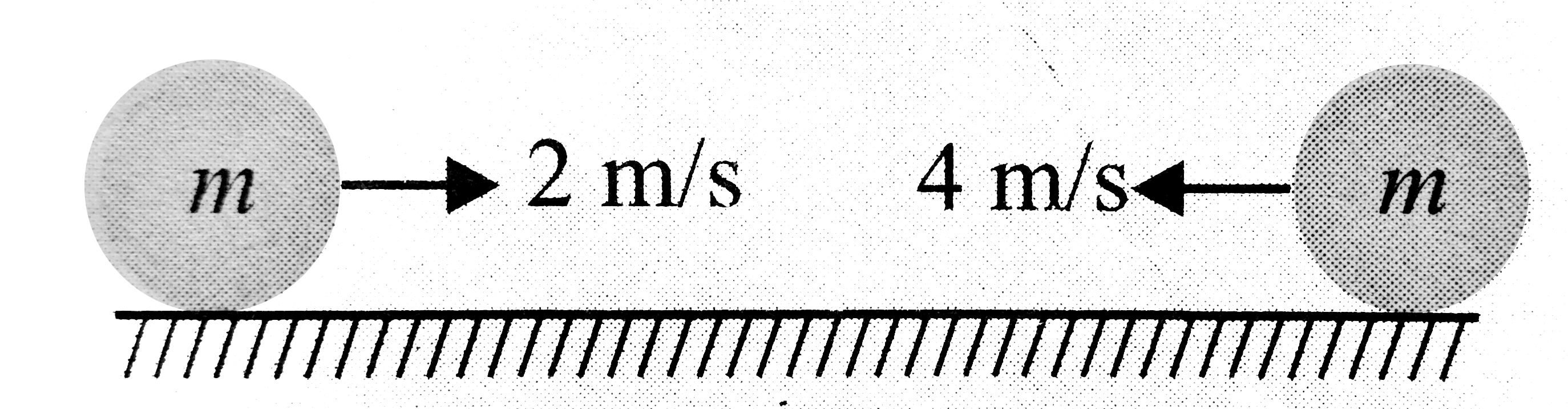
View Solution
Knowledge Check
Two particles are 100m apart and start approaching each other with constant velocities of 2m/sand3m/s. The particles will meet after.
A20 sB50 sC33.3 sD15 sTwo trains one 60 m long and other is 80 m long are travelling in opposite direction with velocity 10 m/s and 25 m/s . The time of crossing is :
A4 secondB5 secondC6 secondD3 secondA train crosses a pole in 15 seconds when it is moving at 20 m/s. Find the time taken by the train to cross a platform 600 m long.
A40sB1minC45sD3min
Two trains A and B are moving on same track in opposite direction with velocity 25m/s and 15,m/s" respectively.When separation between them becomes 225m drivers of both the trains apply brakes producing uniform retardation in train A while retardation of train B increases linearly with time at the rate of 0.3m/s^(3).The minimum retardation of train A to avoid collision will be
View SolutionTwo trans one t ravelling at 54 kmph and the other at 72 kmph are headed towards one another along a straingt track. When they are 1/2 km apart, both drivers simultaneously seee the other train and apply their brakes. If each train is decelerated at the rate of 1m/s2, will there be a collision ?
View Solutiontwo cars approach each other on a straight road. Car A moves at 16m/s and car B moves at 8m/s. When they are 45m apart both drivers apply their brakes. Car A slows down at .2m/s2 . While car B slows down at 4m/s2. Where and when do they collide?
View SolutionA motor car moving with a uniform speed of 20 m/sec comes to stop on the application of brakes after travelling a distance of 10m, its deceleration is
View SolutionTwo trains 100 m long are moving opposite to each other with a speed of 50 m/s each. Find the time taken by the train to cross each other.
View Solution
