Ariel enters a contest to sel advertisements in ther school's yearbook. To qualify for a prize. She has to sell at least $1,500 worth of advertisements consisting of no fewer than 15 invididual ads. Each full-page ad costs $110, each half-page and costs $70, and each quarter-page ad costs %50. Which of the following systems of inequalities represents this situation, whre x is the number of full-page ads she sells, y is the number of half-page ads she sells, and z is the number of quarter-page ads she sells?
110x+70y+50z≥1,500
x+y+z≤15
110x+70y+50z≤1,500
x+y+z≤15
110x+70y+50z≥1,500
x+y+z≥15
110x+70y+50z≤1,500
x+y+z≥15
The correct Answer is:C
To solve the problem, we need to formulate a system of inequalities based on the conditions provided.
1. Understanding the Variables:
- Let x be the number of full-page ads sold.
- Let y be the number of half-page ads sold.
- Let z be the number of quarter-page ads sold.
2. Setting Up the First Inequality:
- Each full-page ad costs $110, so the total revenue from full-page ads is 110x.
- Each half-page ad costs $70, so the total revenue from half-page ads is 70y.
- Each quarter-page ad costs $50, so the total revenue from quarter-page ads is 50z.
- Ariel needs to sell at least $1,500 worth of advertisements. Therefore, we can write the first inequality as:
110x+70y+50z≥1500
3. Setting Up the Second Inequality:
- Ariel must sell no fewer than 15 individual ads. This means the total number of ads sold (full-page, half-page, and quarter-page) must be at least 15. Thus, we can write the second inequality as:
x+y+z≥15
4. Combining the Inequalities:
- We now have two inequalities that represent the situation:
1. 110x+70y+50z≥1500
2. x+y+z≥15
5. Final System of Inequalities:
- The system of inequalities that represents the situation is:
110x+70y+50z≥1500x+y+z≥15
- Let x be the number of full-page ads sold.
- Let y be the number of half-page ads sold.
- Let z be the number of quarter-page ads sold.
- Each full-page ad costs $110, so the total revenue from full-page ads is 110x.
- Each half-page ad costs $70, so the total revenue from half-page ads is 70y.
- Each quarter-page ad costs $50, so the total revenue from quarter-page ads is 50z.
- Ariel needs to sell at least $1,500 worth of advertisements. Therefore, we can write the first inequality as:
110x+70y+50z≥1500
- Ariel must sell no fewer than 15 individual ads. This means the total number of ads sold (full-page, half-page, and quarter-page) must be at least 15. Thus, we can write the second inequality as:
x+y+z≥15
- We now have two inequalities that represent the situation:
1. 110x+70y+50z≥1500
2. x+y+z≥15
- The system of inequalities that represents the situation is:
110x+70y+50z≥1500x+y+z≥15
Topper's Solved these Questions
- INEQUALITIESBOOK - KAPLANCHAPTER - INEQUALITIESEXERCISE - LINEAR INEQUALITIES
1 Videos
- INEQUALITIESBOOK - KAPLANCHAPTER - INEQUALITIESEXERCISE - SYSTEM OF INEQUALITIES
1 Videos
- INEQUALITIESBOOK - KAPLANCHAPTER - INEQUALITIESEXERCISE - MODELING REAL-LIFE SITUATIONS WITH INEQUALITIES
1 Videos
- IMAGINARY NUMBERS BOOK - KAPLANCHAPTER - IMAGINARY NUMBERS EXERCISE - ARITHMETIC OPERATIONS WITH COMPLEX NUMBERS
1 Videos
- LINEAR EQUATIONS AND GRAPHSBOOK - KAPLANCHAPTER - LINEAR EQUATIONS AND GRAPHSEXERCISE - LINEAR GRAPH
1 Videos
Similar Questions
Knowledge Check
Allision is planting a garden with at least 15 trees. There will be a conbination of apple trees, which cost $120 each, and pear trees, which cost $145 each. Allision's budget for purchasing the trees is on more than $2,050. She must plane at least 5 people trees and at least 3 pear trees. Which of the following systems of inequaliteies represents the situation described if x is the number of apple trees and y is the number of pear trees ?
A120x+145y≥2,050
x+y≤15
x≥5
y≥3B120x+145y≥2,050
x+y≥15
x≤5
y≤3C120x+145≤2,050
x+y≥15
x≤5
y≤3D120x+145y≤2,050
x+y≥15
x≥5
y≥3Francine sells advertising time packages for a local television station. She is able to make up to 15 sales calls per week offering potentila advertisers either a prime time package for$12,000 or a non prime time package for $8,000. Her weekly sales goal is to sell more than $20,000 worth of advertising. Which of the following systems of inequalities represents this situation in terms of p, the number of prime time packages Francine sells in a week, n, the number of non prime time packages, and u, the number of unsuccessful sales calls for whihic she sells neither offering ?
Ap+n+u≤15
12,000p+8,000n>20,000Bp+n+u≥15
12,000p+8,000n>20,00Cp+n+u≤15
12,000p+8,000(n+u)>20,000Dp+n+u≤15
12,000p+8,000n<20,000Heinrich must buy at least 100 shares of stock for his portfolio. The shares he buys will be from stock X. Which costs $22 per share and Stock Y. Which costs $35 per share. His budget for buying stock is no more than $4,500. He must buy at least 20 shares of Stock X and 15 shares of Y. WHich of the following represents the situation described if a is the number of shares of Stock X purchased and b is the number of shares of stock Y purchased?
A22a+35b≤4,500
a+b≥100
a≤20
b≤15B22a+35b≤4,500
a+b≤100
a≤20
b≤15C22a+35b≤4,500
a+b≤100
a≥20
b≥15D22a+35b≤4,500
a+b≥100
a≥20
b≥15
Give reason for each of the following:
Gelatin is added to icecreams.
View SolutionAn online electronics store must sell at least $2,500 worth of printers and monitors per day. Each printer costs $125 and each monitor costs $225 and each monitors costs $125. The store can ship a maximum of 15 items per day. What is the maximum number of printers it can ship each day?
View SolutionAn electric cable costs Rs. 200. If the cable was 3m longer and each meter of cable cost Rs 2 less, the cost of the cable would remain unchanged. Represent the above situation in the form of quadratic equation.
View SolutionThe following table gives the number of pages written by Saria for completing her own book for 30 days.
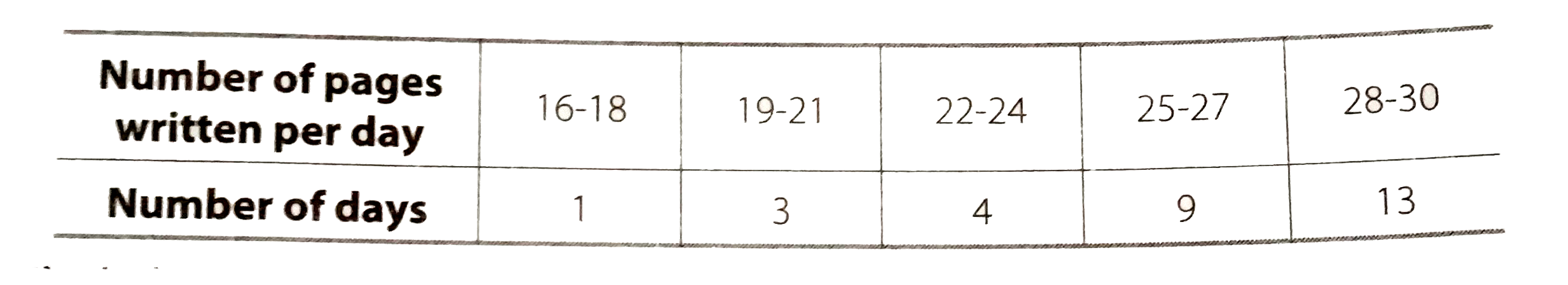
Find the mean number of pages written per day.
View SolutionLynne has $8.00 to spend on apples and oranges. Apples cost $0.65 each, and oranges cost $0.75 each. If there is no tax on this purchase and she buys 5 apples, what is the maximum number of whole oranges she can buy?
View Solution
