Assertion : A solid and a hollow sphere both of equal masses and radii are put on a rough surface after rotating with some angular velocity say $ω_{0}$ . Coefficient of friction for both the spheres and ground is same. Solid sphere will start pure rolling first
Reason : Radius of gyration of hollow sphere about an axis passing through its centre of mass is more.

A

If both Assertion and Reason are correct and Reason is the correct explanation of Assertion

B

If both Assertion and Reason are true but Reason is not the correct explanation of Assertion

C

If Assertion is true but Reason is fasle

D

If Assertion is false but Reason is true

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Pure rolling will start when

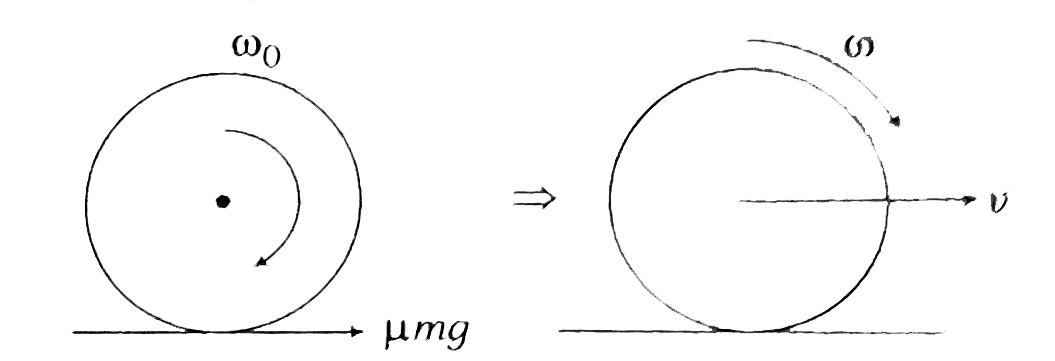
$v = R ω, μ g t = R [ω_{0} - \frac{μ_{0} g R}{I} t]$
$∴ t = \frac{R ω_{0}}{μ g + \frac{μ m R}{I}}, I_{solid} < I_{hollow} ∴ t_{solid} < t_{hollow}$ .

|

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A ring and a solid sphere of same mass and radius are rotating with the same angular velocity about their diameteric axes then :-
Ait is easier to stop the ring
Bit is easier to stop the solid sphere
Cit is equally difficult to stop both of them
Dit is not possible to stop a rotating body
Question 2 - Select One
A solid sphere and a hollow sphere of equal mass and radius are placed over a rough horizontal surface after rotating it about its mass centre with same angular velocity $ω_{0}$ . Once the pure rolling starts let $v_{1}$ and $v_{2}$ be the linear speeds of their centres of mass. Then
A $v_{1} = v_{2}$
B $v_{1} < v_{2}$
C $v_{1} > v_{2}$
Ddata is insufficient
Question 3 - Select One
In above problem, if coefficient of friction for both the spheres is same and let $t^{1}$ and $t^{2}$ be the times when pure rolling of solid sphere and of hollow sphere is started. Then :
A $t_{1} = t_{2}$
B $t_{1} < t_{2}$
C $t_{1} > t_{2}$
DNone of these

DC PANDEY-ROTATION-(B) Chapter Exercises

Home
Ask Doubt
Profile