In a distribution, the mean the mode are 46 and 10 respectively. Calculate the median. Solution in Tamil
Step by step video & image solution for In a distribution, the mean the mode are 46 and 10 respectively. Calculate the median. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- STATISTICSBOOK - SURA PUBLICATIONCHAPTER - STATISTICSEXERCISE - Additional Questions and Answers (Exercise 8.4
1 Videos
- STATISTICSBOOK - SURA PUBLICATIONCHAPTER - STATISTICSEXERCISE - Additional Questions and Answers (Exercise 8.5
1 Videos
- STATISTICSBOOK - SURA PUBLICATIONCHAPTER - STATISTICSEXERCISE - Additional Questions and Answers (Exercise 8.2)
4 Videos
- SET LANGUAGEBOOK - SURA PUBLICATIONCHAPTER - SET LANGUAGEEXERCISE - UNIT TEST
20 Videos
- TRIGONOMETRYBOOK - SURA PUBLICATIONCHAPTER - TRIGONOMETRYEXERCISE - UNIT TEST
16 Videos
Similar Questions
The mean of first 10 natural numbers.
View SolutionThe mean of a distribution is 23, the median is 24 and the mode is 25.5. It is most likely that this distribution is :
View SolutionFor the following up grouped data 8, 15, 14, 19, 11, 16, 10, 8, 17, 20. Find the median.
View SolutionThe set P={x∣x∈Z,−1<x<1} is a
View SolutionFrom the given figure, find all the trigonometric ratios of angle B.

View SolutionCalculate mean in an asymmetrical distribution if mode is 16 and median is 15.73.
View SolutionGiven median =10 and mean =12, calculate mode.
View SolutionIf the sum of the mode and mean of a certain frequency distribution is 129 and the median of the observations is 63, mode and median are respectively
View SolutionThe median and mode of a frequency distribution are 26 and 29 respectively. Then , the mean is
View SolutionIf the mode and median of a frequency distribution are 31 and 37 respectively, find the arithmetic mean.
View SolutionIf the mean and median of a frequency distribution are 35 and 33 respectively, find mode.
View SolutionThe mean and the median of a distribution are 45.9 and 46 respectively. The mode will be
View SolutionIf the mean and mode of a distribution is 5 and 4 respectively, then find the value of median.
View SolutionIf the median and mode for a moderate asymmetrical distribution are 8 and 5 respectively, then the value of mean is
View SolutionCalculate the mean, the median and the mode of the following distribution:
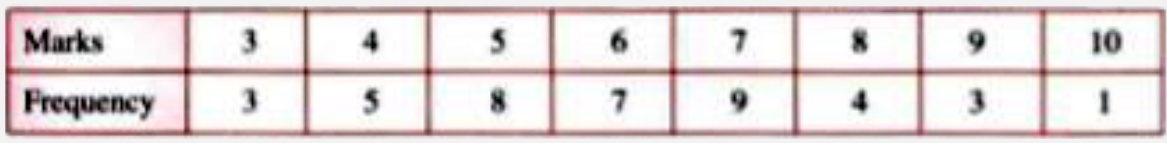
View Solution
