In Q.118, total distance travelled by the particle from $t = 0$ to $t = t_{0}$ is:

$\frac{A_{0}}{2 α}$

$\frac{A_{0}}{α}$

$\frac{2 A_{0}}{α}$

$\frac{4 A_{0}}{α}$

Text Solution

Verified by Experts

The correct Answer is:A

Updated on:21/07/2023

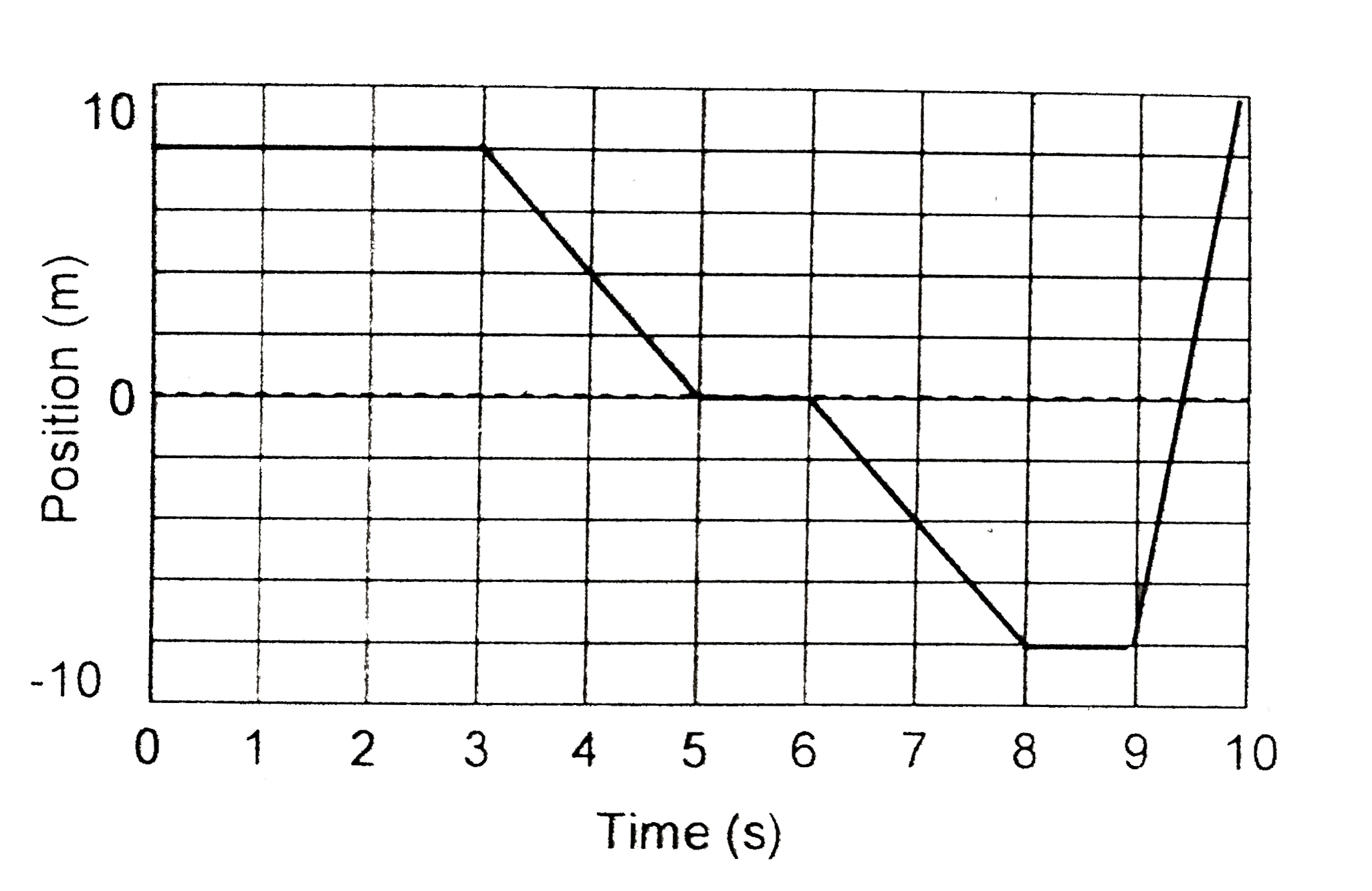
The position versus time graph of a particle moving along a straight line is shown. What is the total distance travelled by the particle from t=0s to t=10s?
A2m
B18 m
C26m
D34m
A particle starts its $S H M$ from mean position at $t = 0$ . If its time period is $T$ and amplitude $A$ then the distance travelled by the particle in the time from $t = 0 \to t = \frac{5 T}{A}$ is
A $A$
B $3 A$
C $4 A$
D $5 A$
Position of a particle moving along x-axis is given by $x = 2 + 8 t - 4 t^{2}$ . The distance travelled by the particle from $t = 0$ to $t = 2$ is:-
A0
B8
C12
D16