Consider a particle moving along x-axis. Its distance from origin O is described by the co-ordinate x which varies with time. At a time t1, the particle is at point P, where its co-ordinate is x1 and at time t2, the particle is at point Q, where its co-ordinate is x2. The displacement during the time interval from t1 to t2 is the vector from P to Q, the x-component of this vector is (x2−x1) and all other components are zero.
It is convenient to represent the quantity x2−x1 the change in x by means of a notation Δ, thus Δx=x2−x1 and Δt=t2−t1.
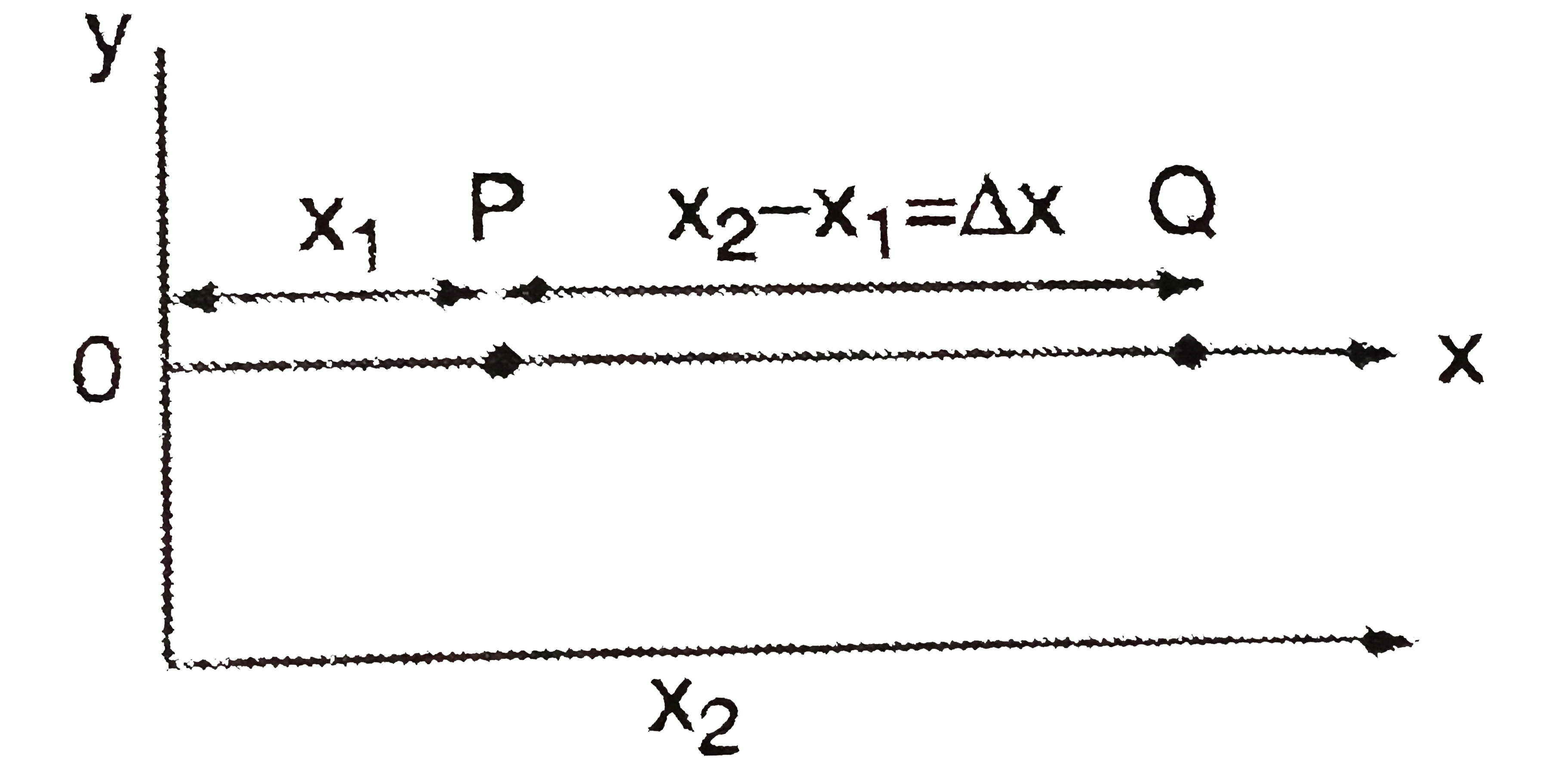
Consider a particle moving along x-axis. Its distance from origin O is described by the co-ordinate x which varies with time. At a time t(1), the particle is at point P, where its co-ordinate is x(1) and at time t(2), the particle is at point Q, where its co-ordinate is x(2). The displacement during the time interval from t(1) to t(2) is the vector from P to Q, the x-component of this vector is (x(2) - x(1)) and all other components are zero. It is convenient to represent the quantity x(2) - x(1) the change in x by means of a notation Delta, thus Delta x = x(2) - x(1) and Delta t = t(2) - t(1). The average velocity bar(V) = (x(2) - x(1))/(t(2) - t(1)) = (Delta x)/(Delta t) A particle moves half the time of its journey with u. The rest of the half time it moves with two velocities v(1) and v(2) such that half the distance it covers with v(1) and the other half with v(2). The net average velocity is: (Assume straight line motion)
The average velocity ¯¯¯V=x2−x1t2−t1=ΔxΔt
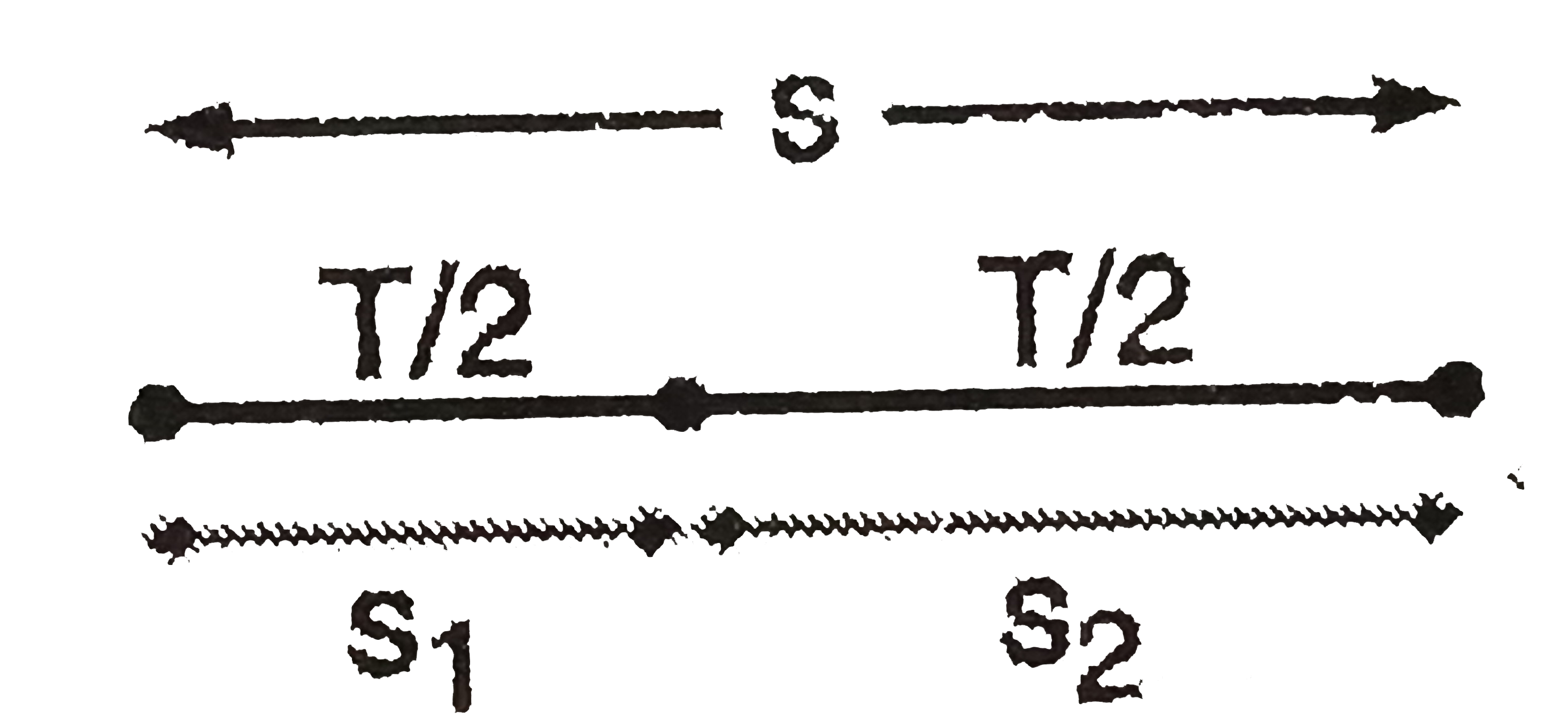
A particle moves half the time of its journey with u. The rest of the half time it moves with two velocities v1 and v2 such that half the distance it covers with v1 and the other half with v2. The net average velocity is: (Assume straight line motion)

A

u(v1+v2)+2v1v22(v1+v2)

B

2u(v1+v2)2u+v1+v2

C

u(v1+v2)2v1

D

2v1v2u+v1+v2

Text Solution
Verified by Experts

The correct Answer is:A

Let total time is T
s1=u×T2
s22=v1×t1
and s22=v2×t2
where t1+t2=T2
Av. Velocity =Total distanceTotal time
=(s1+s2T)
ocr_image

Updated on:21/07/2023
Doubtnut Promotions Banner
|
Class 11PHYSICSRECTILINEAR MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Consider a particle moving along x-axis. Its distance from origin O is described by the co-ordinate x which varies with time. At a time t1, the particle is at point P, where its co-ordinate is x1 and at time t2, the particle is at point Q, where its co-ordinate is x2. The displacement during the time interval from t1 to t2 is the vector from P to Q, the x-component of this vector is (x2−x1) and all other components are zero.
    It is convenient to represent the quantity x2−x1 the change in x by means of a notation Δ, thus Δx=x2−x1 and Δt=t2−t1.
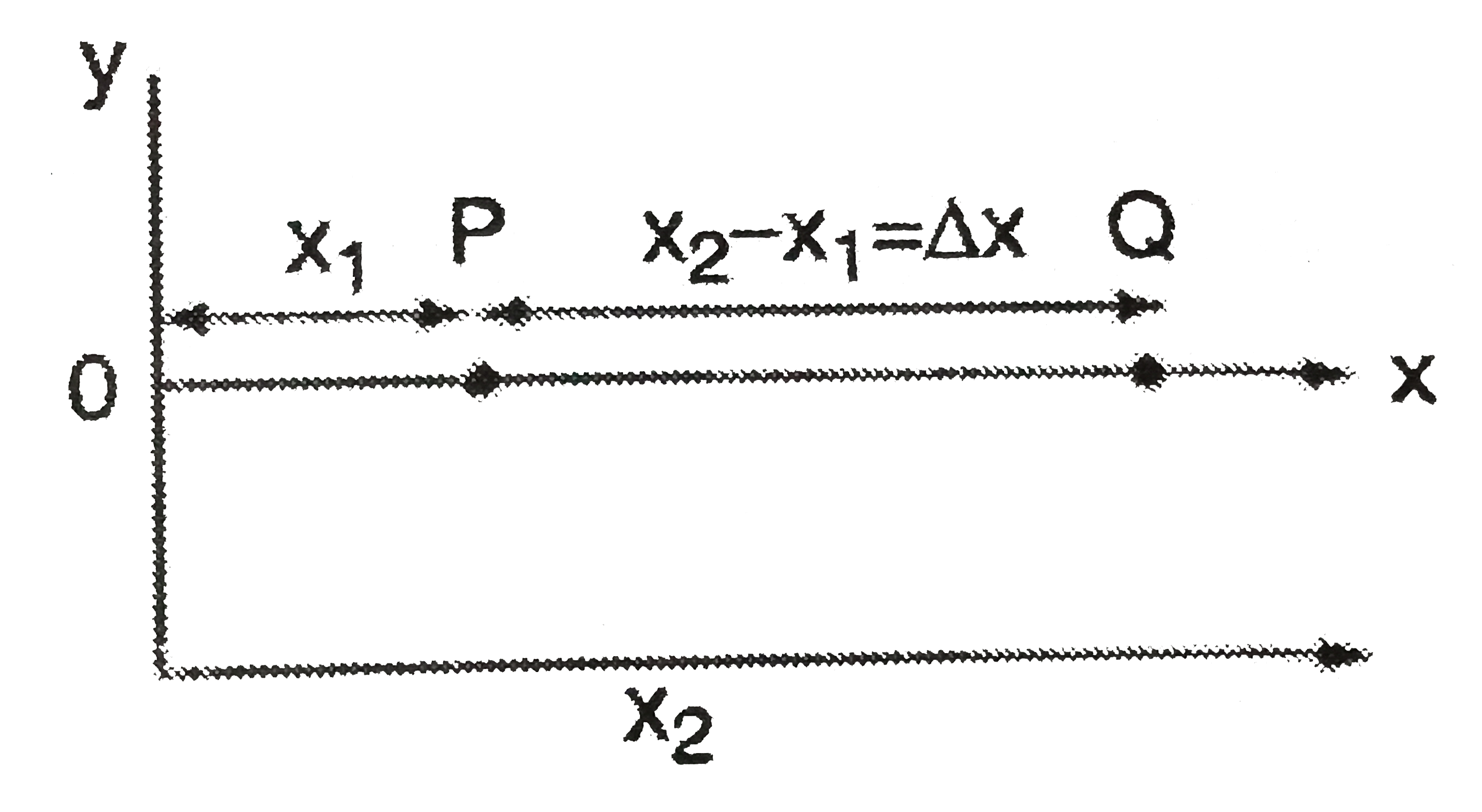
    ocr_image
    The average velocity ¯¯¯V=x2−x1t2−t1=ΔxΔt
    A particle moves according to the equation x=t2+3t+4. The average velocity in the first 5 s is:

    A8 m/s
    B7.6 m/s
    C6.4 m/s
    D5.8 m/s

  • Consider a particle moving along x-axis. Its distance from origin O is described by the co-ordinate x which varies with time. At a time t1, the particle is at point P, where its co-ordinate is x1 and at time t2, the particle is at point Q, where its co-ordinate is x2. The displacement during the time interval from t1 to t2 is the vector from P to Q, the x-component of this vector is (x2−x1) and all other components are zero.
    It is convenient to represent the quantity x2−x1 the change in x by means of a notation Δ, thus Δx=x2−x1 and Δt=t2−t1.
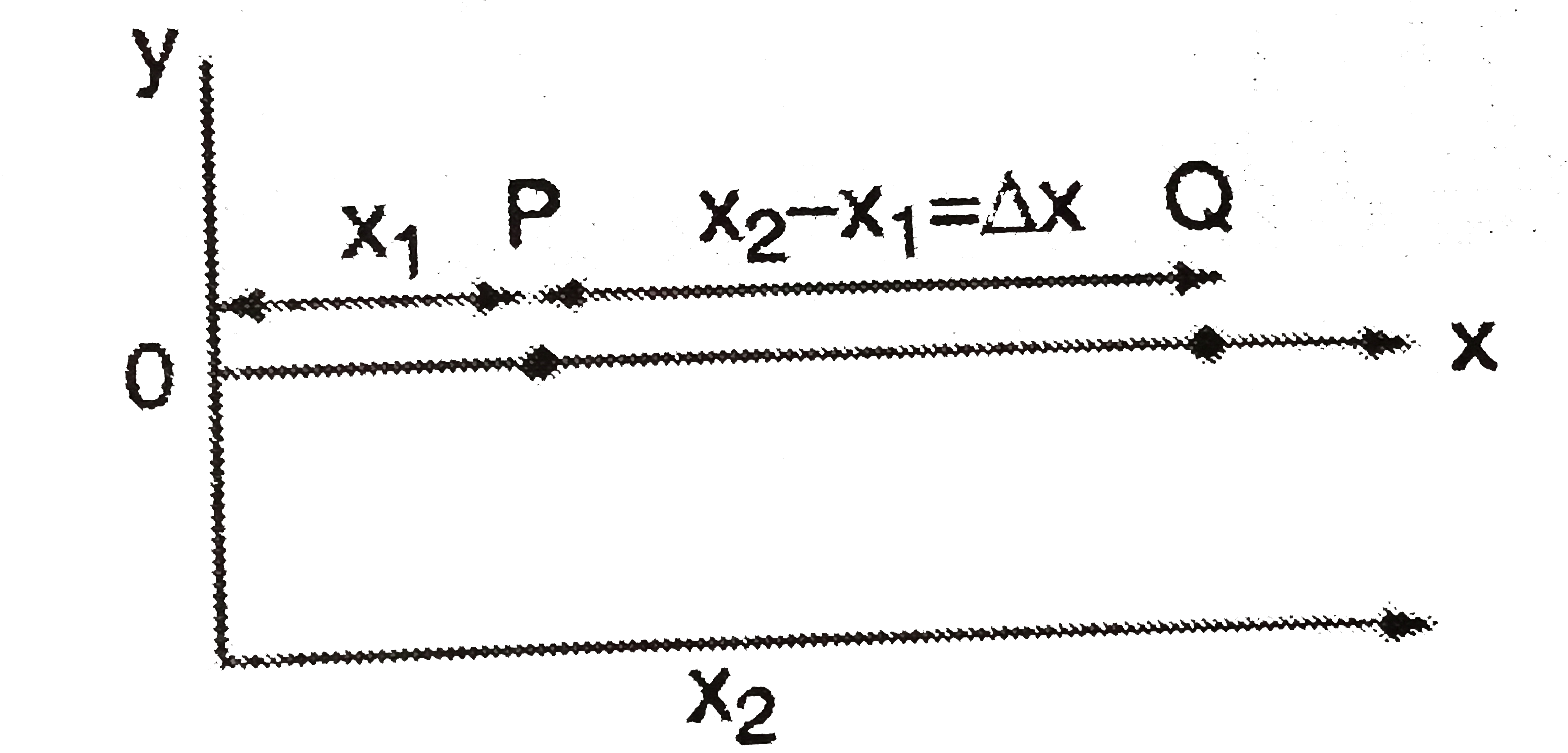
    ocr_image
    The average velocity ¯¯¯V=x2−x1t2−t1=ΔxΔt
    The resistive force suffered by a motor boat is proportional to v2, where v is instantaneous velocity. The engine was shut down when the velocity of the boat was v0. Find the average velocity at any time t.

    Av0+v2
    Bvv02(v0+v)
    Cvv0loge(v0v)(v0−v)
    D2vv0loge(v0v)(v0+v)

  • A particle is moving along x-axis. Its velocity v with x co-ordinate is varying as v=√x. Then

    Ainitial velocity of particle is zero
    Bmotion is non-uniformly accelerated
    Cacceleration of particle at x=2m is 12m/s2
    Dacceleration of particle at x=4m is 1m/s2