A man wishes to cross a river flowing with velocity u swims at an angle θ with the river flow.If the man swims with speed vand if the width of the river is d, then the drift travelled by him is
(u+vcosθ)dvsinθ
(u−cosθ)dvsinθ
(u−vcosθ)dvcosθ
(u+vcosθ)dvcosθ
The correct Answer is:a
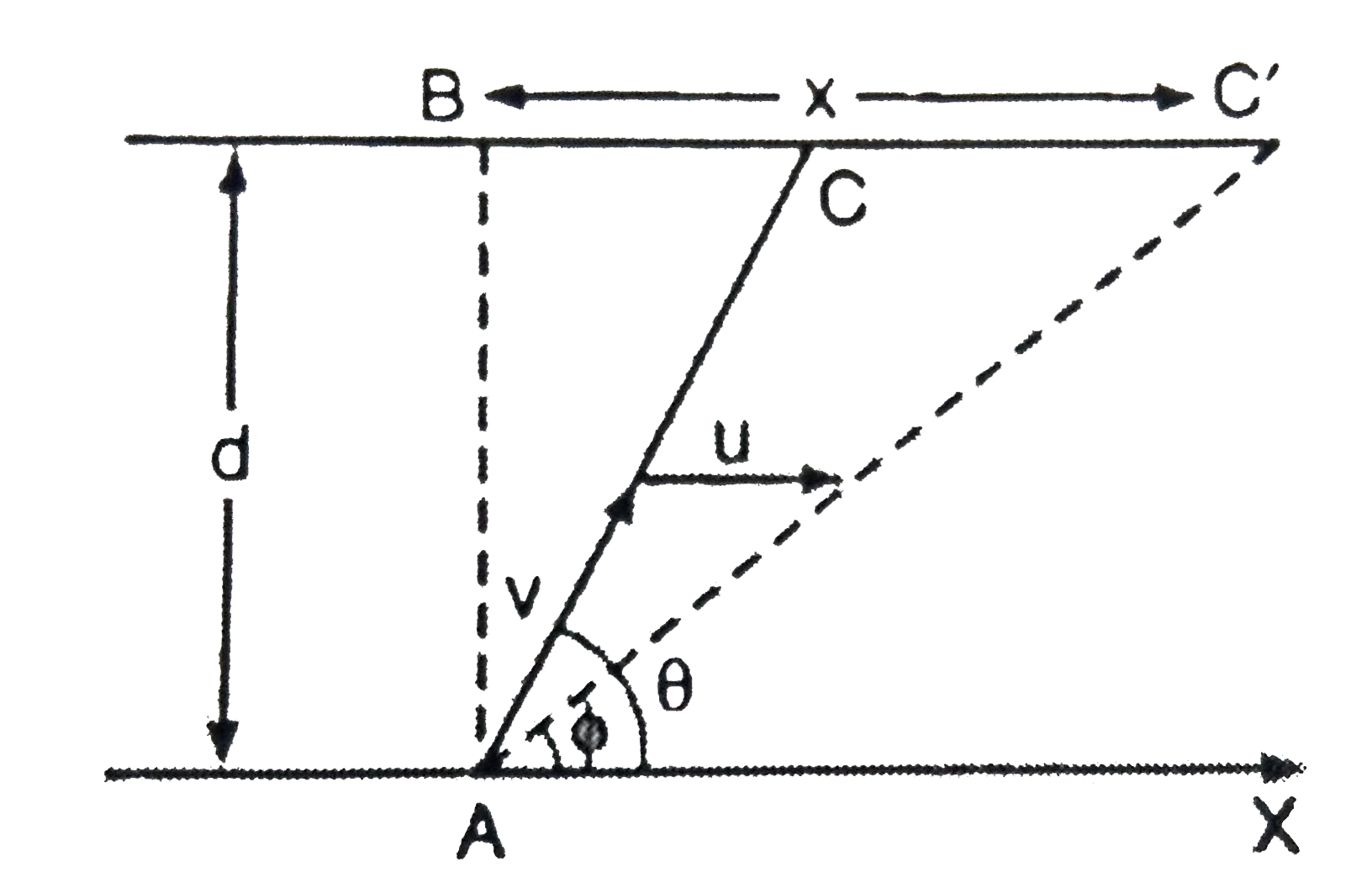
x=(u+vcosθ)t=(u+ucosθ)dvsinθ
Topper's Solved these Questions
Similar Questions
A ,man wishing to cross a river flowing with velocity u jumps at an angle θ with the river flow.
(a) Find the net velocity of the man with respect to ground if he can swim with speed v in still water.
(b) In what direction does the boat actually move ?
( c) Find how far from the point directly opposite to the starting point does the boat reach the opposite bank, if the width of the river is d.
View SolutionA man can swim at a speed of 5km/h in the still water. He wants to cross a 1600m wide river flowing at 4km/h. He keeps himself at an angle 127∘ with the river flow while swimming. (a) Find the time he takes to cross the river. (b) Find the distance traveled by him along the flow.
View Solution
Knowledge Check
A man can swim in still water at a speed of 6kmph and he has to cross the river and reach just opposite point on the other bank.If the river is flowing at a speed of 3kmph,and the width of the river is 2km, the time taken to cross the river is (in hours)
A227B2√27C23D2√45A man wishes to cross a river to an exactly opposite point on the other bank. if the can swim with twice the velocity of the current, the inclination to the current of the direction he should swim is
A30∘B45∘C60∘D120∘A man can swim at a speed of 3kmh−1 in still water. He wants to cross a 500−m wide river flowing at 2kmh−1. He keeps himself always at an angle to 120∘ with the river flow while swimming.
The drift of the man along the direction of flow, when he arrives at the opposite bank is.A16√3kmB6√3cmC3√3kmD13√3km
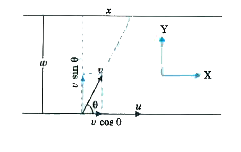
A man can swim in still water with a speed v . River flows at a speed u which is greater than the swimming speed (v) of the man . Explain that the man cannot move perpendicular to the flow . If man swims at an angle θ with the river flow , then find drift along the flow by the time he crosses the river . Also find the minimum value of the drift along the flow x by the time he crosses the river .
View SolutionA man can swim at a speed of 3 km/h in still water. He wants t cross a 500 m wide river flowing at 2 km/h. He flow at an angle of 120 with the river flow while swimming. A. Find the time he takes to cross the river. b.At what point on the opposite bank will he arrive?
View SolutionA swimmer wishes to reach directly opposite bank of a river, flowing with velocity 8 m/s. The swimmer can swim 10 m/s still water. The width of the river is 480 m. Time taken by him to do so:
View SolutionA man can swim with speed 5ms−1 in still river while the river is also flowing speed 10ms−1 If the width of the river is 100 m then minimum possible drift is
View SolutionA man can swim in still water at a speed of 4kmph. He desires to cross a river flowing at a speed of 3kmph in the shortest time interval. If the width of the river (in hours) and the horizontal distance travelled (in km) are respectively
View Solution
