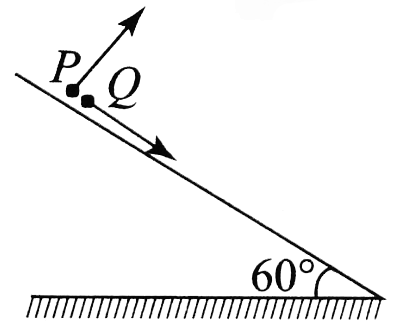
A particle P is projected from a point on the surface of smooth inclined plane (see figure). Simultaneously another particle Q is released on the smooth inclined plane from the same position. P and Q collide aftert=4. The speed of projection of P is
5m/s
10m/s
15m/s
20m/s
The correct Answer is:d
It can be obseved from figure that P and Q shall collide if the initial component of velocity 'P' on inclined plane i.e., along incline u=0i.e., particle is p rojected perpendicu lar to incline.
∴ Time of light tAB=usinθg
(time to reach the maximum height of projectile) particle rebound with ucosθ in vertical direction (as collision is elastic ).
Time required to reach maximum height
=tAB+tBC=usinθg+ucosθg
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
A ball is projected from point A with velocity 10ms−1 perpendicular to the inclined plane as shown in figure. Range of the ball on the inclined plane is
 A403mB203mC123mD603m
A403mB203mC123mD603m - Question 2 - Select One
A ball is projected from point A with velocity 10ms−1 perpendicular to the inclined plane as shown in figure. Range of the ball on the inclined plane is
 A403mB2013mC1320mD1340m
A403mB2013mC1320mD1340m - Question 3 - Select One
Figure shows that particle A is projected from point P with velocity u along the plane and simultaneously another particle B with velocity v at an angle α with vertical. The particles collide at point Q on the plane. Then
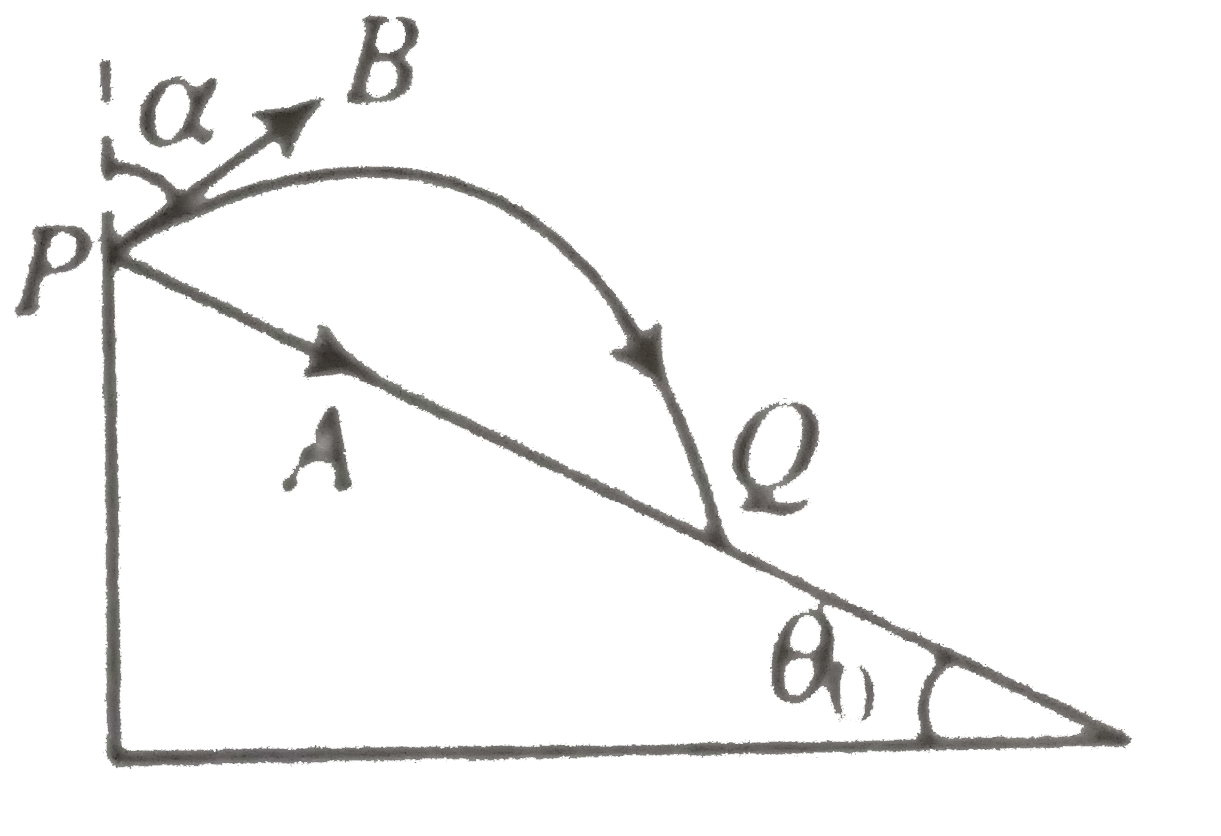 Avsin(α−θ0)=uBvcos(α−θ0)=uCv=uDNone of these
Avsin(α−θ0)=uBvcos(α−θ0)=uCv=uDNone of these
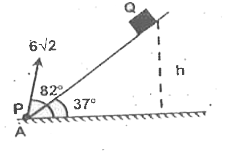
A particle (P) is projected at an angle of 82∘ from horizontal with a velocity 6√2m/s from the bottom of an inclined plane, having angle of inclination 37∘ At the same instant, another particle: Q is released from a heighth on a smooth incline plane. If after some time both particles collide, then find the
(a) value of time after which they collide,
(b) the height h
 View Solution
View Solution
A particle is projected from surface of the inclined plane with speed u and at an angle θ with the horizontal. After some time the particle collides elastically with the smooth fixed inclined plane for the first time and subsequently moves in vertical direction. Starting from projection, find the time taken by the particle to reach maximum height. (Neglect time of collision).View SolutionA particle is projected on an inclined plane with a speed u as shown in (Fig. 5.61). Find the range of the particle on the inclined plane.
 .View Solution
.View SolutionA particle is projected with a velocity u from the foot of an inclined plane whose inclination to the horizon is β. It strikes. the plane at right angles if
View SolutionA block slides down a smooth inclined plane when released from the top, while another falls freely from the same point :
View Solution


