A ball is projected from the ground with velocity v such that its ranege is maximum.

Text Solution

Verified by Experts

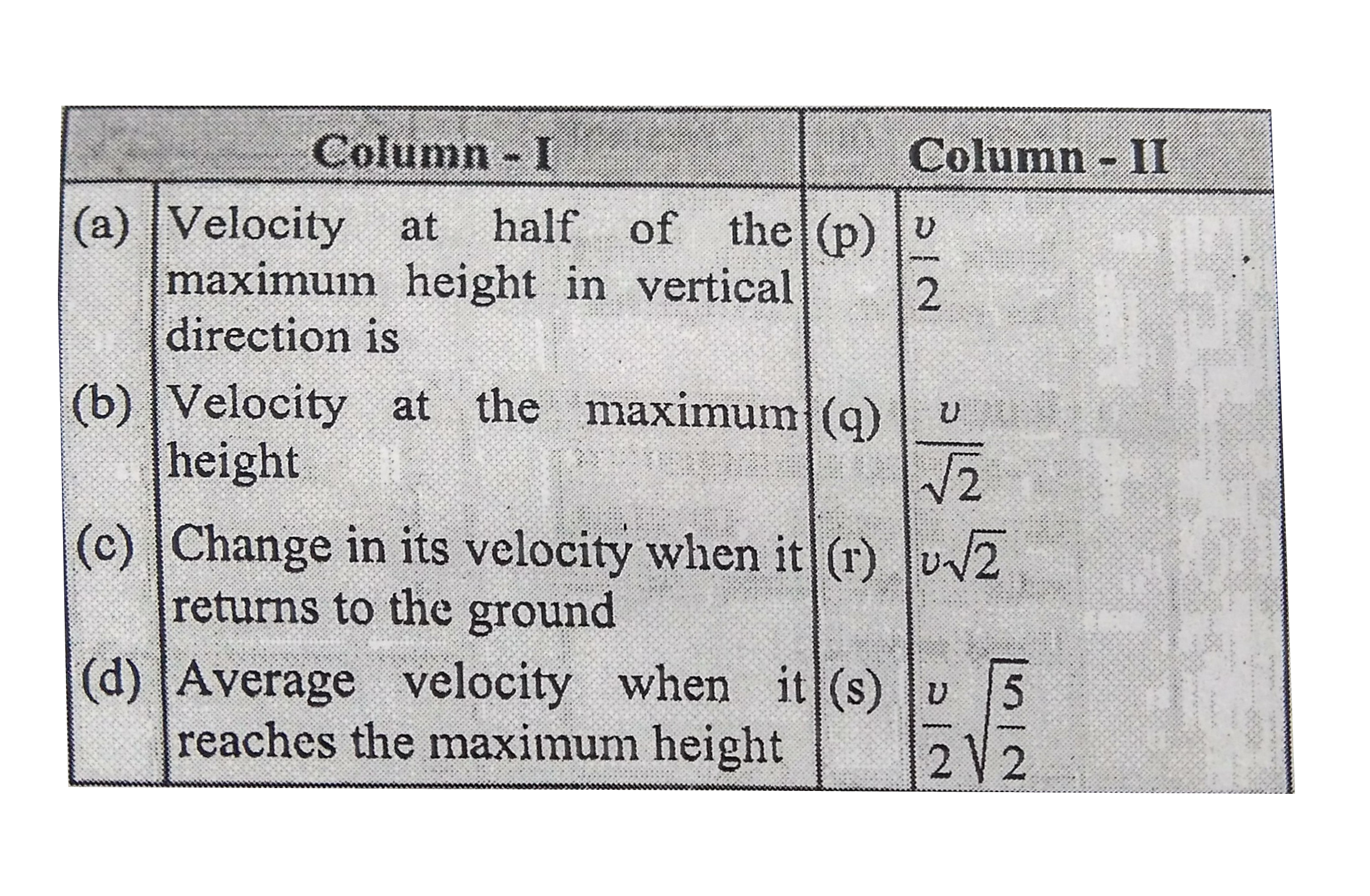
The correct Answer is: $a \to p; b \to q; c \to r; d \to s$

The range of projectile is maximum.
$∴ θ = 45^{\circ}$
and $H = \frac{v^{2} {sin}^{2} 45^{\circ}}{2 g} = \frac{v^{2}}{4 g}$
(a) Using, $v_{y}^{2} - u_{y}^{2} = 2 a_{y} Y$
$v_{y}^{2} - \frac{v^{2}}{2} = 2 (- g) \frac{H}{2}$
$= - g (\frac{v^{2}}{4 g}) = - \frac{v^{2}}{4}$
$∴ v_{y}^{2} = \frac{v^{2}}{4} \Rightarrow v_{y} = \frac{v}{2}$
$∴ (a) \to (p)$
(b) At the maximum height, the ball has only horizontal velocity.
$∴ v_{x} = \frac{v}{\sqrt{2}}$
$∴ (b) \to (q)$
(c) Horizontal velocity remains the same but the vertical velocity gets reversed.
$∴ Δ v_{y} = \frac{v}{\sqrt{2}} - (- \frac{v}{\sqrt{2}}) = \frac{2 v}{\sqrt{2}} = v \sqrt{2}$
$∴ (c) \to (r)$
(d) ${\to v}_{a v} = \frac{1}{2} ({\to v}_{1} + \to - - - (f))$
$= \frac{1}{2} [\frac{v}{\sqrt{2}} (ˆ i + ˆ j) + \frac{v}{\sqrt{2}} ˆ i]$
$= \frac{1}{2} [\frac{2 v}{\sqrt{2}} ˆ i + \frac{v}{\sqrt{2}} ˆ j]$
$∴ | {\to v}_{a v} | = \frac{1}{2} \sqrt{(\frac{4 v^{2}}{2} + \frac{v^{2}}{2})} = \frac{v}{2} \sqrt{\frac{5}{2}}$
$∴ (d) \to (s)$

|

Updated on:21/07/2023

Class 11 PHYSICS PROJECTILE MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A ball is projected from the ground with velocity $u$ such that its range is maximum Then
AIts velocity at half the maximum height is $\frac{\sqrt{3}}{2} u$
BIts velocity at the maximum height is $u$
CChange in its velocity when it returns to the ground is $u$
Dall the above are true
Question 2 - Select One
From a point on the ground a particle is projected with initial velocity $u$ ,such that its horizontal range is maximum. The magnitude of average velocity during its ascent.
A $\frac{\sqrt{5 u}}{2 \sqrt{2}}$
B $\frac{5 u}{4}$
C $\frac{\sqrt{3}}{2 \sqrt{2}}$
DNone
Question 3 - Select One
A body is projected obliquely from the ground such that its horizontal range is maximum.If the change in its maximum height to maximum height, is $P$ , the change in its linear momentum as it travels from the point of projection to the landing point on the ground will be
A $P$
B $\sqrt{P}$
C $2 P$
D $2 \sqrt{2} P$

GRB PUBLICATION-MOTION IN TWO AND THREE DIMENSIONS-All Questions

Home
Ask Doubt
Profile