In a fig. PA is a tangent to the circle. PBC is a secant & AD bisects angle BAC. Show that triangle PAD is an isosceles triangle.Also show that ∠CAD=12(∠PBA−∠PAB)
Step by step video & image solution for In a fig. PA is a tangent to the circle. PBC is a secant & AD bisects angle BAC. Show that triangle PAD is an isosceles triangle.Also show that /_CAD=1/2(/_PBA-/_PAB) by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
In the figure, AB is a diameter PA is a tangent to the circle and PCB is a secant. Show that △ABP~△CAP
View SolutionIn a triangle ABC, if acosA=bcosB, show that the triangle is either isosceles or right angled.
View SolutionIn Fig. 10.64, BC is a tangent to the circle with centre O.OE bisects AP. Prove that ΔABC~ΔAEO
View SolutionAs shown in the figure, PA is a tangent to a circle and PBC is a secant, so that PB = AB. Prove that PB.PC=AC2
View SolutionIn a triangle ABC , if cosA=sinB2sinC , show that the triangle is isosceles.
View SolutionIn the figure, ∠D=∠EandADDB=AEEC, prove that ΔBAC is an isosceles triangle
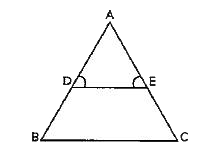
View SolutionD is a point on the side BC of a triangle ABC such that ∠ADC=∠BAC. Show that CA2=CB.CD.
View SolutionShow that the right triangle of maximum area that can be inscribed in a circle is an isosceles triangle
View SolutionIn the figure, ∠D=∠E and ADDB=AEEC, prove that BAC is an isosceles triangle.

View SolutionIn the adjoining figure, ABC is a triangle in which AD is the bisector of ∠A. If AD⊥BC, show that △ABC is isosceles.
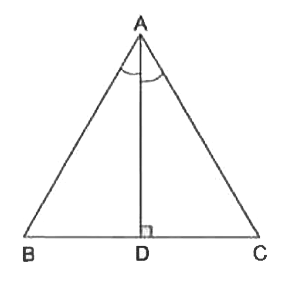
View Solution
