Find the equillibrium length for the system after increasing temperature by ΔT.
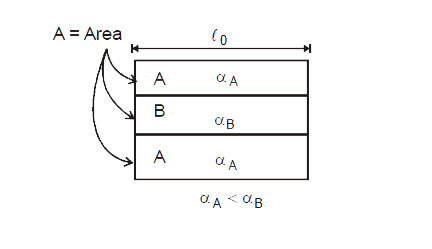
here lAandlB are the natural length of the rod A and B after increase in temperature by ΔT,andl0 is actual length after temperature increase by ΔT.
So strain in A =l'0−l'Al'A
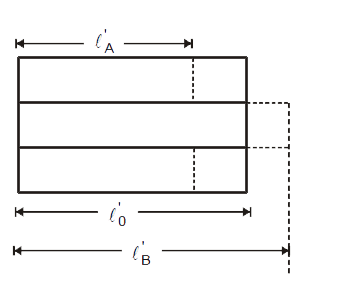
and in B=l'B−l'0l'B
Now force balance
Now FA=γA=l'0−l'Al'A ...(1)
and 2FA=γBl'B−l'0l'B ....(2)

(1) ÷ (2)
12=γAγB[l'0−l'0(1+αAΔT)]l0(1+αBΔT)l0(1+αAΔT)[l0(1+αBΔT)−l'0]
l'0=l0(γB+2γA)[1+(αB+αA)ΔT)2lA(1+αBΔT)+γB(1+αAΔT)
Topper's Solved these Questions
- BOOK - MOTIONCHAPTER - ELASTICITY AND THERMAL EXPANSIONEXERCISE - Exercise -1 (Section A - Stress & types, Strain & types + Types of Modulus + Energy Problems + Graphs)6videos
ELASTICITY AND THERMAL EXPANSION
- BOOK - MOTIONCHAPTER - ELASTICITY AND THERMAL EXPANSIONEXERCISE - Exercise -1 (Section B - Thermal Stress, Hookes Law)6videos
ELASTICITY AND THERMAL EXPANSION
- BOOK - MOTIONCHAPTER - ELASTICITYEXERCISE - EXERCISE -360videos
ELASTICITY
- BOOK - MOTIONCHAPTER - Electrical Instrument EXERCISE - EXERCISE -316videos
Electrical Instrument
Similar Questions
The coefficient of linear expansion varies linearly from α1 and α2 in a rod of length l. Find the increase in length when its temperature is increased by ΔT.
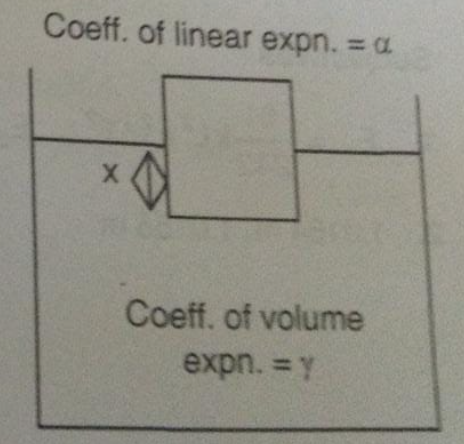
View SolutionA cubical block is floating inside a bath. The temperature of system is increased by small temperature δ(T). It was found that the depth of submerged portion of cube does not change. Find the relation between coefficient of linear expansion (α) of the cube and volume expansion of liquid (γ).
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
The temperature of the system increases during an
Aisothermal expansionBadiabatic compressionCadiabatic expansionDisothermal compression - Question 2 - Select One
The coefficient of linear expansion varies linearly from α1 and α2 in a rod of length l. Find the increase in length when its temperature is increased by ΔT.
A(α1+α22)lΔTB(α1+α2)lΔTC(α1+α22)lΔTD(α12+α2)lΔT - Question 3 - Select One
Consider the following statements
I. In general, the temperature dependence of the equilibrium constant depends on the sign of ΔH for the reaction.
II. The equilibrium constant for an exothermic reaction (negative ΔH) decreases as the temperature increases.
III. The equilibrium constant for an endothermic reaction (positive ΔH) increases as the temperature increases.
Choose the correct statement(s).AI and IIBOnly IICII and IIIDAll of these
A small amount of water and its saturated vapour are enclosed in a vessel at a temperature t=100∘C. How much (in per cent) will the mass of the saturated vapour increase if the temperature of the system goes up by ΔT=1.5K ? Assume that the vapour is an ideal gas and the specific volume of water is negligible as compared to that vapour.
View SolutionTwo rods of material X sandwich another rod of material Y as shown in figure. At temperature t, the three rods are in a state of zero strailn and of length L and riveted to each other in this state. If the temperature of the system increases to T+ΔT, find the final length of the system of the three rods. Given that the coefficients of linear expansions of the roods and their Young's modulus for material X and Y are alpnax,αy,Yx and Yy respectively. Consider αy>αx.
 View Solution
View SolutionThe ratio of specific heats (Cp and Cv) for an ideal gas is γ. Volume of one mole sample of the gas is varied according to the law V=aT2 where T is temperature and a is a constant. Find the heat absorbed by the gas if its temperature changes by ΔT.
View SolutionThe radious of a metal sphere at room temperature T is R, and the coefficient of linar expansion of the metal is α. The sphere is heated a little by a temperature ΔT so that its new temperature is T+ΔT. The increase in the volume of the sphere is approximately
View SolutionWhen the common salt dissolves in water, the entropy of the system increases. This means that the sign of ΔS is ______ and randomness of the system _______
View Solution

