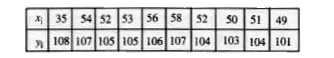
From the prices of shares X and Y given below: find out which is more stable value: X: 35 54 52 53 56 58 52 50 50 49 Y: 108 107 105 105 106 107 104 103 104 101

Text Solution

Verified by Experts

$b e g \in {a r r a y} {c} x_{i} & \leq f t | x_{i} - ¯ x r i g h t | & \leq f t {| x_{i} - ¯ x r i g h t |}_{i}^{2} 35 & 16 & 25654 & 3 & 952 & 1 & 153 & 2 & 456 & 5 & 2558 & 7 & 4952 & 1 & 150 & 1 & 151 & 0 & 049 & 2 & 4 Total = 510 & & e n d {a r r a y}$
Here, the number of observations $n = 10$ $ $ therefore text { Mean, } bar{x}=frac{1}{n} sum_{i=1}^{10} x_{i}=frac{1}{16} times 51 phi=51 $$ variance $(σ_{1}^{2}) = \frac{1}{n} 10 \sum i = 1 {(x_{i} - ¯ x)}_{i}^{2}$ =frac{1}{10} times 35 phi=35 $$ therefore $S tan d a r d d e v i a t i o n$ left(sigma_{1}right)= $\sqrt{3} 5 = 5.91 .$
$b e g \in {t a b a - - r} {c} h l \in e$ y i $&$ left|y_{i}-bar{y}right| $&$ left|y_{i}-bar{y}right|^{2} $108 & 3 & 4107 & 2 & 0105 & 0 & 0105 & 0 & 1106 & 1 & 4107 & 2 & 1104 & 1 & 4103 & 2 & 1104 & 1 & 16101 & 4 & 40 h l \in e T o t$ =1050 $& & 4 h l \in e e n d {t a b a - - r} H e r e, t h e ν m b e r a f o b s e r v a t i o n s$ n=10therefore $M e a n,$ bar{y}=frac{1}{n} sum_{i=1}^{10} y_{i}=frac{1}{10} times 105 phi^{10}=105 $v a r i a n c e$ left(sigma_{2}^{2}right)=frac{1}{n} sum_{i=1}^{10}left(y_{i}-bar{y}right)^{2} $= \frac{1}{10} \times 40 = 4 .$
therefore $S tan d a r d d e v i a t i o n$ left(sigma_{2}right)=sqrt{4}=2 $ Sin c e, t h e v a r i a n c e o f p r i c e s o f s h a r e x i s g r e a t e r t h a n t ˆ o f p r i c e s a f s h or e y . S o, p r i c e s a f s h a r e$ X $a r e m or e v a r i a b \leq t h a n p r i c e s a f s h a r e$ y $. S o, t h e p r i c e s a f s h a r t s$ y $a r e m or e s t a b \leq t h a n t h e p r i c e s a f s h a r e$ x.

Updated on:21/07/2023

When a number arrangment machine is given an input line of numbers, it arranges them following is a particular rule. The following is an illustration of an input and its rearrangement. $\begin{matrix} Input: 33 91 79 23 29 60 12 35 Step I: 99 273 223 67 120 24 105 Step II: 174 36 168 8 43 121 81 Step III: 28 18 48 8 12 2 8 Step IV: 14 9 24 4 6 1 4 \end{matrix}$ Step IV, is the last step of the above arragement as the intended arrangement is obtained. As per the rules followed in the given steps find out appropriate steps for the given input Input : $39 36 47 52 68 71 91 77$ What is the addition of the numbers which is $2^{n d}$ from the left end and $4^{t h}$ from the right end in step IV of the given arrangment ?
A109
B29
C17
D7