A rigid bodyI=CMR2 is set into a motion on a rough horizontal surface with a linear speed v0 in the forward direction at time t = 0 as shown in figure. After what time slipping finally stop and pure rolling starts. Find the linear speed of the body after it starts pure rolling on the surface.
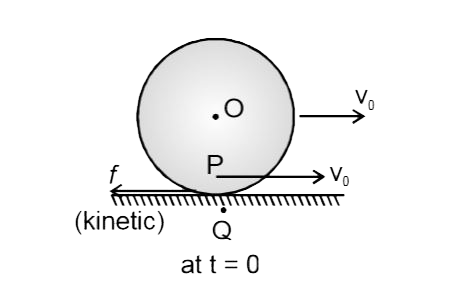
According to the given condition in problem the point P in the body move with speed v0 while the point Q on the ground is at rest. So the friciton acts on the body is in backward direction which gives the resultant torque on the body and increase the angualr speed ω as shown in figure.
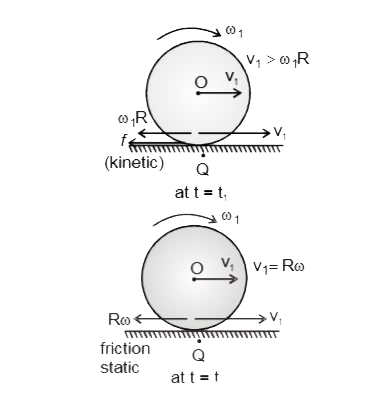
As shown in above figure initially v>Rω so forward slipping takes place. After introducing the friciton speed decreases and omrga increases and at time t = t the relation v = r ω is satisfied. Therefore pure rolling starts. Initially the friciton is kinetic untill the motion is in slipping condition. Afterwards at v = rω fricition is static. We divide the above problem in two parts.
(1) Translational Motion :
Linear acceleration a = μg
So after time t,v=v0−μgt ...(1)
(2) Rotational Motion :
From τnet=Iα
Only friction force is responsible for providing torque. So torque about O is
f.R=Iα
μmgR=CmR2α ...(2)
α is angular acceleration of the body
From eq.(2) αμgCR
∵ω=VR at pure rolling condition.
So, v=μgtC ...(3)
from eq. (1) & (3)
⇒v0−μgt=μgtC⇒t=v0Cμg(1+C) ...(4)
Equation (4) gives the time after the pure rolling starts.
Put the value from eq. (4) to eq. (1)
v=v0−v0C(1+C)⇒v=v01+C ...(5)
Equation (5) gives the linear speed at pure rolling situation.
Alternate solution :
Net torque on the body about the bottom most point A is zero. Therefore angular momentum of the body will remain conserved about the bottom most point
Net torque about AτA=0
⇒ from Angular momentum conservation Li=Lf
mv0R=Iomrga+mvR
mv0R=CmR2vR+mvR
v0=Cv+v⇒v=v01+C
Topper's Solved these Questions
Similar Questions
A solid sphere is set into motion on a rough horizontal surfce with a linar speed v in the forward direction and an angular speed v/R in the anticlockwise direction as shown infigure . Find the linear speed of the sphere a. where it stops rotating and b. when slipping finally ceases sand pure rolling starts.

View SolutionA solid sphere of radius 2.45m is rotating with an angular speed of 10rad/s. When this rotating sphere is placed on a rough horizontal surface then after sometime it starts pure rolling. Find the linear speed of the sphere after it starts pure rolling.
View Solution
Knowledge Check
A solid sphere is set into motion on a rough horizontal surface with a linear velocity v in the forward direction and an angular velocity v/R in the anticlockwise direction as shown in the figure. Find the linear speed of the sphere, when it stops rotating.
 Av5Bv7C3v5D3v7
Av5Bv7C3v5D3v7A symmetrical body of mass M and radius R is rolling without slipping on a horizontal surface with linear speed v. Then its angular speed is
Av/RBContinuously increasingCDependent on mass MDIndependent of radius (R)A sphere is moving on a smooth surface with linear speed v0 and angular velocity ω0. It finds a rough inclined surface and it starts climbing up:
 Aif v0>Rω0 friction force will act downwardsBif v0<Rω0, frictio force will act upwardsCif v0=Rω0 no friction force will actDif all above case friction will act upwards
Aif v0>Rω0 friction force will act downwardsBif v0<Rω0, frictio force will act upwardsCif v0=Rω0 no friction force will actDif all above case friction will act upwards
A uniform wheel of radius R is set lying on a rough horizontal surface is hit by as cue is such a way that the line of action passes thruogh the centre of the shell. As a result the shelll starts movign with a linear speed v without any initial angular velocity. Find the linear speed of the shell after it starts pure rolling on the surface.
View SolutionA thin spherical shell lying on a rough horizontal surface is hit by as cue in such a way that the line of action passes through the centre of the shell. As a result the shell starts moving with a linear speed v without any initial angualr velocity. Find the linear speed of the shell after it starts pure rolling on the surface.
View SolutionA solid sphere of radius R is set into motion on a rough horizontal surface with a linear speed v0 in forward direction and an angular with a linear speed v0 in forward direction and an angular velocity ω0=v0/2R in counter clockwise direction as shown in figure. If coefficient of friction μ then find
a. the time after which sphere starts pure rolling,
b. the linear speed of sphere when it starts rolling and
c. the work down by friction over a long time.
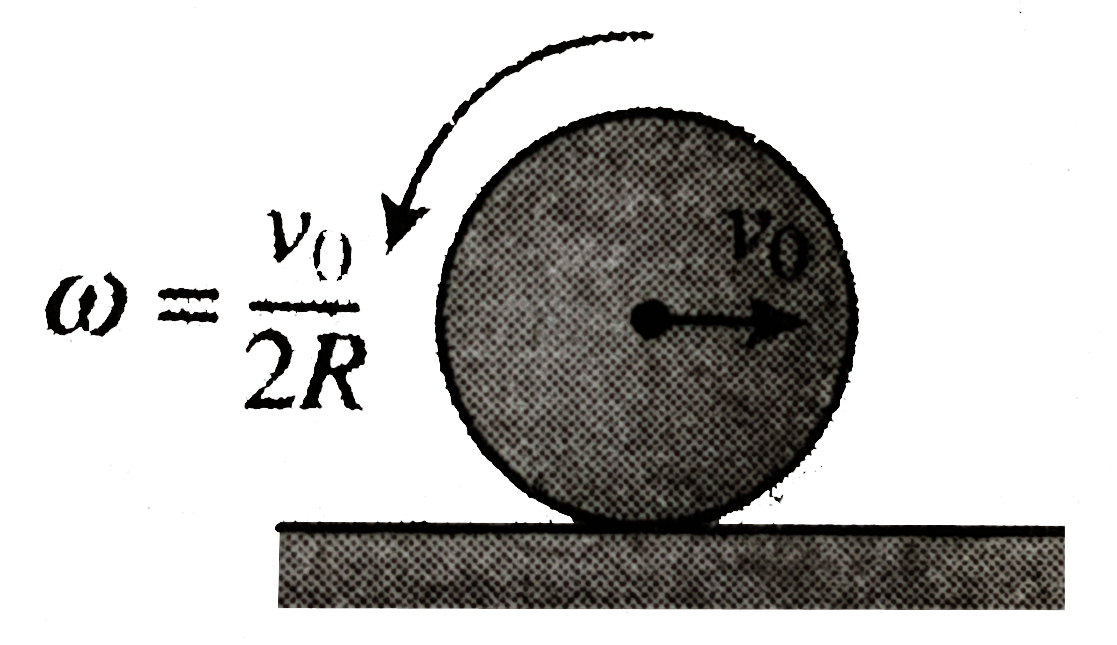
View SolutionA solid sphere rolling on a rough horizontal surface with a linear speed v collides elastically with a fixed, smooth, vertical wall. Find the speed of the sphere after it has started pure rolling in the backward direction.
View Solution
A cicular disc of mass m and radius R is set into motion on a horizontal floor with a linear speed v in the forward direction and an angular speed ω=vR in clockwise direction as shown in figure. Find the magnitude of the total angular momentum of the disc about bottom most point O of the disc.
View Solution
