Three concentric metallic spherical shells A,B and C of radii a,b and c(a<b<c) have surface charge densities −σ,+σand −σ respectively ,the potential of shell A is
(σ/ε0)[a+b−c]
(σε0)[a−b+c]
(σ/ε0)[b−a−c]
none
The correct Answer is:C
Step by step video, text & image solution for Three concentric metallic spherical shells A,B and C of radii a,b and c(a lt blt c) have surface charge densities -sigma ,+sigma and -sigma respectively ,the potential of shell A is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ELECTROSTATICS-IIBOOK - MOTIONCHAPTER - ELECTROSTATICS-IIEXERCISE - Exercise 1 (Section D - Plates)
2 Videos
- ELECTROSTATICS-IIBOOK - MOTIONCHAPTER - ELECTROSTATICS-IIEXERCISE - Exercise - 2 (Level - I) Section A - Questions Based on electric flux for various configuration, Question on solid angle concept, Gauss law Based Questions
12 Videos
- ELECTROSTATICS-IIBOOK - MOTIONCHAPTER - ELECTROSTATICS-IIEXERCISE - Exercise 1 (Section B - Properties of conductors)
15 Videos
- ELECTROSTATICS - I BOOK - MOTIONCHAPTER - ELECTROSTATICS - I EXERCISE - EXERCISE - 4 (Level -II) PREVIOUS YEAR - JEE ADVANCED
13 Videos
- ERRORS , THEORY BOOK - MOTIONCHAPTER - ERRORS , THEORY EXERCISE - EXERCISE 4 (LEVEL -II)
10 Videos
Similar Questions
Three concentric spherical metallic shells A,B and C of radii a,b and c (a<b<c) have surface charge densities σ,−σandσ respectively.
If the potenital of sheel B is VB=σ∈0(anb−b+c) and the potential of shell C is VC=σ∈0(anc−bnc+c) then n is .
View SolutionThree concentric spherical metallic shells A, B and C of radii a, b and c (a lt b ltc) have surface charge densities σ, −σ and σ respectively.
(i) Find the potential of the three shells A, B and C.
(ii) If the shells A and C are at the same potential, obtain the relation between the radii a, b and c.
View Solution
Knowledge Check
Three concentric metallic spherical shell A,B and C or radiin a,b and C (a<b>C) have surface charge densities −singama,+σ, and −aigba respectively .
The potential of shell A is :A(σ/ɛ0)[a+b−c]B(σ/ɛ0)[a+b−c]C(σ/ɛ0)[a+b−c]DnoneThree concentric spherical metallic spheres A,B and C of radii a,b and c(a<b<c) have surface charge densities σ,−σ and σ respectively.
AThe potential of shell A:
VA=σε0(a+b+c)BThe potential of shell B:
VB=σε0(a2b−b+c)CThe potential of shell C:
VC=σε0(a2−b2c+c)DAll options are correctThree concentric spherical shells have radii a,b and c(a<b<c) and have surface charge densities σ,−sigam and σ respectively. If VA,VB and VC denote the potentials of the three shells, then for c=q+b, we have
AVC=VB≠VABVC≠V≠VACVC=VB=VADVC=VA≠VB
Three concentric spherical metallic shells A, B and C of radii a, b and c (a lt b ltc) have surface charge densities σ, −σ and σ respectively.
(i) Find the potential of the three shells A, B and C.
(ii) If the shells A and C are at the same potential, obtain the relation between the radii a, b and c.
View SolutionThree concentric metallic shells A, B and C of radii a, b and c(a<b<c) have surface charge densities +σ,−σand+σ respectively as shown. Obtain the expressions for the potential of three shells A, B and C. If shells A and C are at the same potential, obtain the relation between a, b and c.
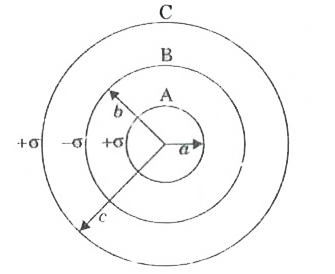
View SolutionThree concentric spherical metallic shells A,B,andC of radii a,b,andc(a<b<c) have surface charge densities σ,−σ and σ, respectively.
If VA,VB and VC are potential of shells A, B and C, respectively, match the columns
Column AColumn Ba.VAi.σε0[a2−b2+c2c]b.VBii.σ2ε0[a2b−b+c]c.VCiii.σε0[a−b+c]
View SolutionThree concentric spherical metallic shells A, B and C of radii a, b and c (a lt b ltc) have surface charge densities σ, −σ and σ respectively.
(i) Find the potential of the three shells A, B and C.
(ii) If the shells A and C are at the same potential, obtain the relation between the radii a, b and c.
View SolutionThree concentric spherical shells have radii a,b and c(a<b<c) and have surface charge densities σ,−σandσ respectively. If VA,VB and VC denote the potentials of the three shells, then for c=a+b, we have
View Solution
