The class mean score on a test was 60, and the standard deviation was 15. If Elena's score was within 2 standard deviations of the mean, what is the lowest score she could have received?
The correct Answer is:30
To find the lowest score Elena could have received, we will follow these steps:
Step 1: Understand the given information.
- Mean score (μ) = 60
- Standard deviation (σ) = 15
- We need to find the lowest score within 2 standard deviations of the mean.
Step 2: Calculate the range of scores within 2 standard deviations of the mean.
- The formula for the lower limit within 2 standard deviations is:
Lower limit=Mean−2×Standard Deviation
Step 3: Substitute the values into the formula.
- Substitute the mean and standard deviation into the formula:
Lower limit=60−2×15
Step 4: Perform the multiplication.
- Calculate 2×15:
2×15=30
Step 5: Subtract the result from the mean.
- Now, subtract 30 from 60:
60−30=30
Step 6: Conclusion.
- Therefore, the lowest score Elena could have received is 30.
- Mean score (μ) = 60
- Standard deviation (σ) = 15
- We need to find the lowest score within 2 standard deviations of the mean.
- The formula for the lower limit within 2 standard deviations is:
Lower limit=Mean−2×Standard Deviation
- Substitute the mean and standard deviation into the formula:
Lower limit=60−2×15
- Calculate 2×15:
2×15=30
- Now, subtract 30 from 60:
60−30=30
- Therefore, the lowest score Elena could have received is 30.
Similar Questions
Find the standard deviation by using 20 as assumed mean.
 View Solution
View SolutionThe standard deviation of 7 scores 1,2,3,4,5,6,7 is
View SolutionOn a test that has normal distribution of scores, a score of 58 falls two standard deviation below the mean, and a score of 85 is one standard deviation above the mean. What is the mean score of this test?
View SolutionFind the mean , mean deviation about the mean , variance and standard deviation for the following data
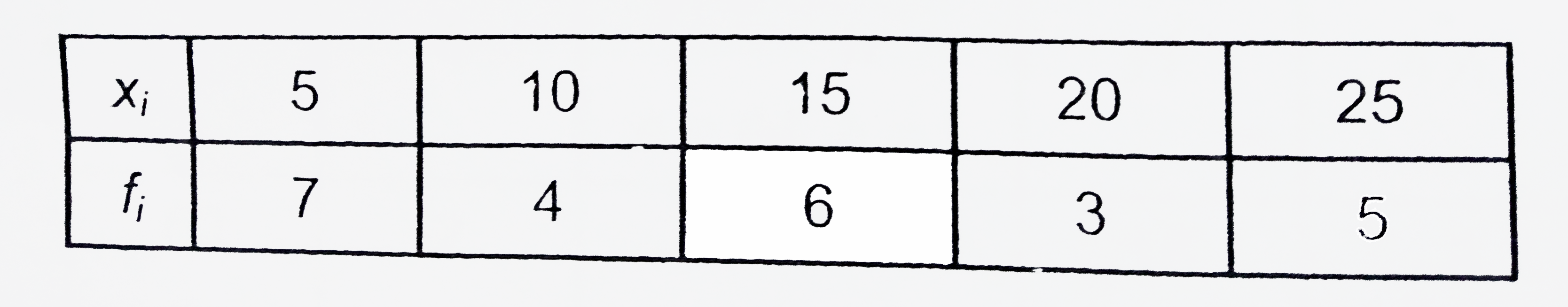 View Solution
View SolutionFind the mean and standard deviation using short cut method
 View Solution
View SolutionOn a test that has a normal distribution of scores of 59 falls two standard deviations below the mean, and score of 74 is one standard deviation above the mean. If x is an integer score that lies between 2.5 and 3.0 standard deviations above the means. What is a possible value of x?
View SolutionThe standard deviation is ….. To the mean deviation taken from the arithmetic mean
View SolutionThe standard deviation of a poisson distribution is 4 then its mean is
View SolutionIf the mean of a binomial distribution is 20 and its standard deviation is 4, find p .
View SolutionIn a binomial distribution the mean is 12 and the standard deviation is √3. Then the distribution is
View Solution

