G is a point inside the plane of the triangle ABC,→GA+→GB+→GC=0, then show that G is the centroid of triangle ABC.
Step by step video & image solution for G is a point inside the plane of the triangle ABC, vecGA + vecGB + vecGC=0, then show that G is the centroid of triangle ABC. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
IF G is the centroid of a triangle ABC. Then →GA+→GB+→GC equals
A→0B3→GAC3→GBD3→GCFind the ratio of the areas of an equilateral triangle ABC and square EFGC, if G is the centroid of the triangle ABC.
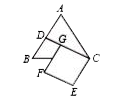 A27:16B1:4C4:3√3D3√3:4
A27:16B1:4C4:3√3D3√3:4If G is the centroid of a triangle ABC, then GA + GB + GC equals to
A0B3 GAC3 GBD3 GC
G is the centroid of the triangle ABC. Prove that the area of the triangle ABG is one third of the area of the triangle ABC.
View SolutionIf G is the centroid of a triangle ABC, prove that →GA+→GB+→GC=→0.
View SolutionIn a ΔABC .G is centroid AG = BC find angle BGC
View SolutionABC is an equilateral triangle with side 12cm and AD is the median. Find the length of GD if G is the centroid of △ABC.
ABC एक समबाहु त्रिभुज है जिसके भुजा 12cm और AD मध्यिका है। GD की लंबाई ज्ञात करें यदि G,△ABC का केन्द्रक है।
View SolutionLet G be the centroid of triangle ABC and the circumcircle of triangle AGC touches the side AB at A
If AC = 1, then the length of the median of triangle ABC through the vertex A is equal to
View Solution
