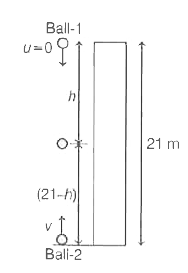
Ball-1 is dropped from the top of a building from rest. At the same moment, ball-2 is throuwn upward toward ball-1 with a speed 14 m/s from a point 21 m below the top of building. How far will the ball-1 have dropped when it passes ball-2. (Assume acceleration due to gravity, $g = 10 m / s^{2}$ ).Solution in Telugu

A

$\frac{45}{4}$ m

B

$\frac{52}{6}$ m

C

$\frac{37}{2}$ m

D

$\frac{25}{2}$ m

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Suppose that both the balls meet at a distance h from ball-1 after time t from the start as shown in the figure.
For downward motion of ball-1 from second equation of the motion,
$h = u t + \frac{1}{2} g t^{2}$ ...(i)
$h = 0 \times t + \frac{1}{2} \times 10 t^{2} [∵ u = 0]$
$h = 5 t^{2}$ ...(ii)
For upward motion of ball-2, from second equation of the motion,
$h = v t - \frac{1}{2} g t^{2}$ ...(iii)
Given, speed of ball-2, $v = 14$ m/s, acceleration due to gravity, $g = 10 m / s^{2}$
Substituting these values in Eq. (iii), we get
$21 - h = 14 t - \frac{1}{2} \times 10 t^{2}$
$\Rightarrow 21 - h = 14 t - 5 t^{2}$ ...(iv)
Adding Eq. (ii) and (iv), we get
$21 = 14 t$
$\Rightarrow t = \frac{3}{2} s = 1.5 s$
$∴$ From Eq. (ii), we get
$h = 5 \times {(1.5)}^{2} = 11.25 m$
$= \frac{45}{4} m$
Therefore, the ball-1 will have dropped $\frac{45}{4}$ m when it passes ball-2.

|

Updated on:21/07/2023

Class 12 PHYSICS TS EAMCET 2019 (3 MAY SHIFT 1)

Topper's Solved these Questions

Similar Questions

TS EAMCET PREVIOUS YEAR PAPERS-TS EAMCET 2019 (3 MAY SHIFT 1)-Physics

Home
Ask Doubt
Profile