Question

Consider a sphere of mass M and radius R centered at origin. The density of material of the sphere is ${\displaystyle \rho =A{r}^{\alpha },}$ where r is the radial distance, ${\displaystyle \alpha \text{and}}$ A are constants. If the moment of inertia of the sphere about the axis passing through centre is ${\displaystyle \frac{6}{7}M{R}^2,}$ then the value of ${\displaystyle \alpha }$ is

Options

A. 3
B. 6
C. 9
D. 12

Answer

A

Explanation

`(**)` Given, density of sphere, `rho = Ar ^(alpha )`
(where, r= radial distance and A and `alpha` are constants)
Consider an elemental spherical shell of radius r and thickness dr

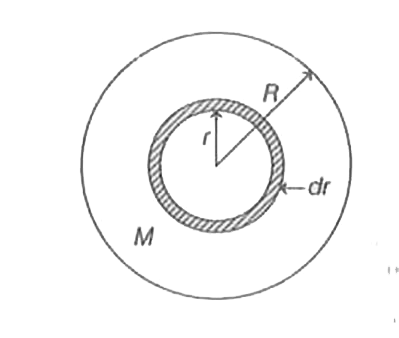
Mass of elemental spherical shell,
dm= Volume `xx` Density
`dm = (4pi r ^(2)) dr. Ar ^(alpha ) = 4pi a r ^(2+ alpha ) dr" "...(i)`
Mass of entire solid sphere,
`M = 4pi A int _(0)^(R) r ^(2+ alpha ) dr`
`M = 4piA [(R ^(3+alpha ))/(3 + alpha )]_(0 ^(R)) = (4piA)/(3 + alpha ) . R ^(3 + alpha)" "...(ii)`
Now, moment of inertia of elemental spherical shell is
`dI = 2/3 (dm). r ^(2) = 2/3 (4pi A r ^(2+alpha ) dr) r ^(2)`
Moment of inertia of entire solid sphere,
`I = int _(0) ^(R) dI = 2/3 4 pi A int _(0) ^(R) r^(A + alpha). dr`
`I = 2/3 4piA [(r ^(5+ alpha ))/(5 + alpha )]_(0)^(R)implies I = 2/3 4pi A [(R ^(5 + alpha ))/(5 + alpha ) ]`
`I = 2/3 ((4piA)/(3 + alpha ) . R ^(3 + alpha )) . (R ^(2) (3 + alpha ))/(5 + alpha )`
From Eq. (ii), we get
`I = 2/3 MR ^(2) . ((3+ alpha )/( 5 + alpha )) " "...(iii)`
It is given in the question.
`I = 6/7 MR^(2) " "(iv)`
On comparing Eqs (iii) and (ov), we get
`2/3 MR^(2). ((3 + alpha )/(5 + alpha ))= 6/7 MR^(2) implies (3 +alpha)/(5 + alpha ) = 9/7`
`21+ 7 alpha = 45 + 9 alpha`
`implies alpha =-12`