A light rigid wire of length 1 m is attached to a ball A of mass 500 g to one end. The other end of the wire is fixed, so that the wire can rotate freely in vertical plane about its fixed end. At the lowest point of the circular motion, the ball is given a horizontal velocity 6 m/s. Determinje the radial component of the acceleration of the ball, when this rigid wire makes an angle 60∘ with the qpward vertical. (Assume g=10m/s2)

10m/s2
6m/s2
18m/s2
25m/s2
The correct Answer is:D
Givne,
Length of a light rigid wire, 1=1m
Mass of ball A, m=500g=0.5kg
Horizontal velocity of ball = 6 m/s
Angle with upward vertical =60∘

From ΔAOB,
cos60∘=OBOA
12=OB1
OB=12
∵AB=OA+OB
=1+12=32m
According to law of conservatin of energy
(KE+PE)x=(KE+PE)A
12mu2+0=12mv2mg(AB)
12mu2=12m(v2+2g(AB))
u2=v2+2g(AB)
62=v2+2(10)×(32)
30+v2=36
v2=36−30
v2=6
v=√6
Radial component of the acceleration of ball,
a=v2l=(√6)21=6m/s2
Topper's Solved these Questions
- BOOK - SIA PUBLICATIONCHAPTER - QUESTION PAPER EXERCISE - CHEMISTRY8videos
QUESTION PAPER
- BOOK - SIA PUBLICATIONCHAPTER - QUESTION PAPER EXERCISE - CHEMISTRY8videos
QUESTION PAPER
- BOOK - SIA PUBLICATIONCHAPTER - QUADRATIC EXPRESSIONS AND THEORY OF EQUATIONS EXERCISE - Problems71videos
QUADRATIC EXPRESSIONS AND THEORY OF EQUATIONS
- BOOK - SIA PUBLICATIONCHAPTER - SAMPLE PAPER 2017EXERCISE - CHEMISTRY3videos
SAMPLE PAPER 2017
Similar Questions
A particle of mass m is attached to an end of a light rigid rod of length a. The other end of the rod is fixed, so that the rod can rotate freely in vertical plane about its fixed end. The mass m is given a horizontal velocity u at the lowest point. (a) Prove that when the radius to the mass makes an angle θ with the upward vertical the horizontal component of the acceleration of the mass (measured in direction of u) is [g(2+3cosθ)−u2/a]sinθ (b) If 4ag ltu2 lt5ag, show that there are four points at which horizontal component of acceleration is zero. locate the points.
View SolutionA small ball of mass m is attached to the end of the string of length l=1 m whose other end is fixed. From its lowest position, the ball is given a kinetic energy ml/5. the net acceleration (in m/s2) of the ball at the instant when the string makes an angle θ of 37∘C with the downwards vertical is___________
View Solution
Knowledge Check
- Question 1 - Select One
A particle of mass m is fixed to one end of a light rigid rod of length l and rotated in a vertical circular path about its other end. The minimum speed of the particle at its highest point must be
AzeroB√glC√1.5glD√2gl - Question 2 - Select One or More
A body of mass M is attached to the lower end of a metal wire, whose upper end is fixed . The elongation of the wire is l.
ALoss in gravitational potential energy ofM is MglBThe elastic potential energy stored in the wire is MglCThe elastic potential energy stored in the wire is 12MglDHeat produced is 12Mgl - Question 3 - Select One
A particle of mass m is fixed to me one end of a light right rod of length l and rotated in a vertical circular path about its other end. The minmum speed of the particle at its highest point must be
AzeroB√glC√1.5glD√2gl
A particle of mass m is fixed to one end of a light rigid rod of length l and rotated in a vertical ciorcular path about its other end. The minimum speed of the particle at its highest point must be
View SolutionA body of mass M is attached to the lower end of a metal wire, whose upper end is fixed . The elongation of the wire is l.
View SolutionA light rigid rod has a small ball of mass m attached to its one end. The other end is hinged on a table and the rod can rotate freely in vertical plane. The rod is released from vertical position and while falling the ball at its end strikes a hemisphere of mass m lying freely on the table. The collision between the ball and the hemisphere is elastic. The radius of hemisphere and length of the rod are R and 2R respectively. Find the velocity of the hemisphere after collision.
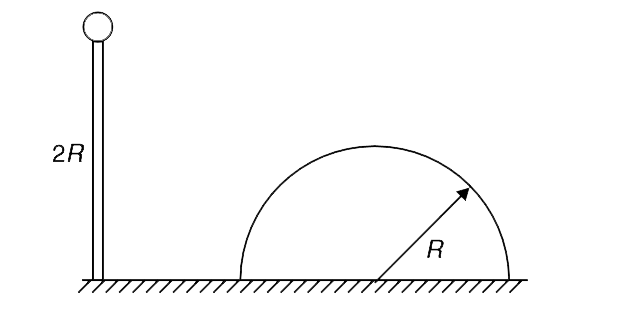 View Solution
View SolutionA particle of mass m is attached to one end of a light inextensible string and the other end of the string is fixed in vertical plane as shown. Particle is given a horizontal velocity u=√52gl
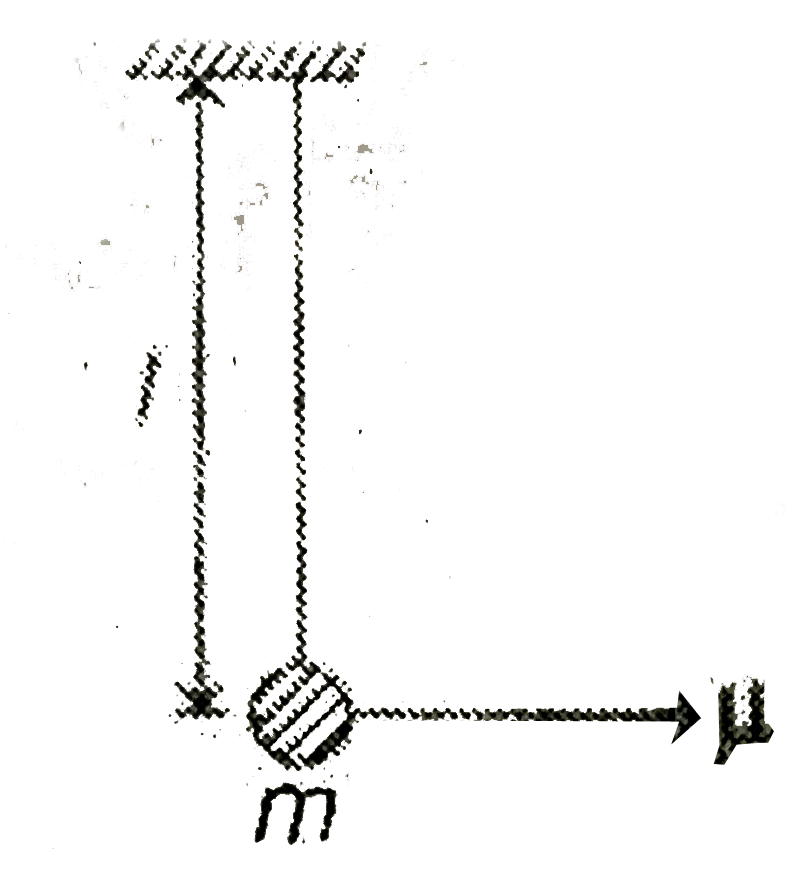
The tension in the string at an instant when acceleration of the particle is horizontal and √3g isView SolutionA sphere of mass m attached with the free end of a steel wire of length l swings in the veritical plane form the horizontal positon.
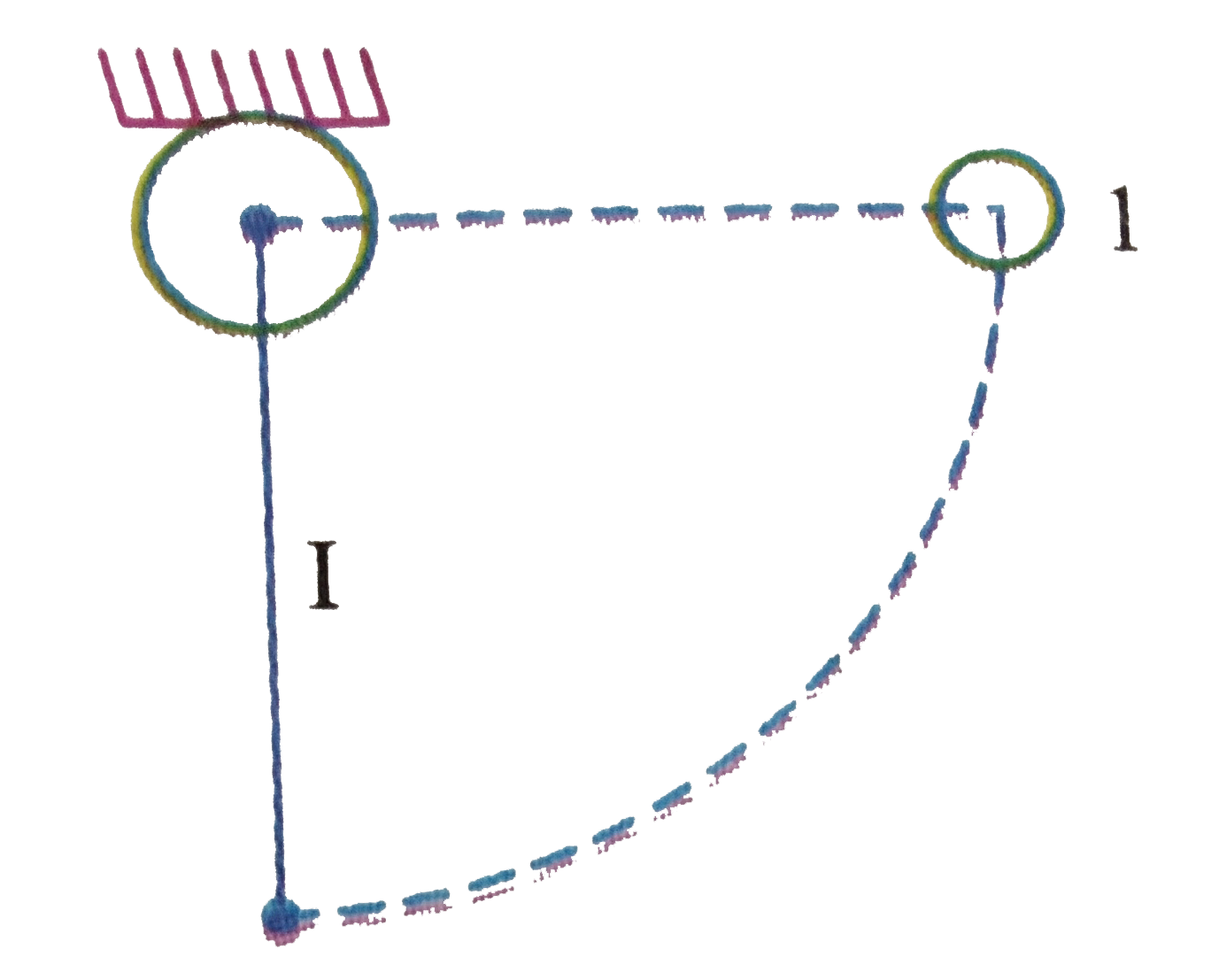
Elongation of the wire in the vericle positon isView Solution

