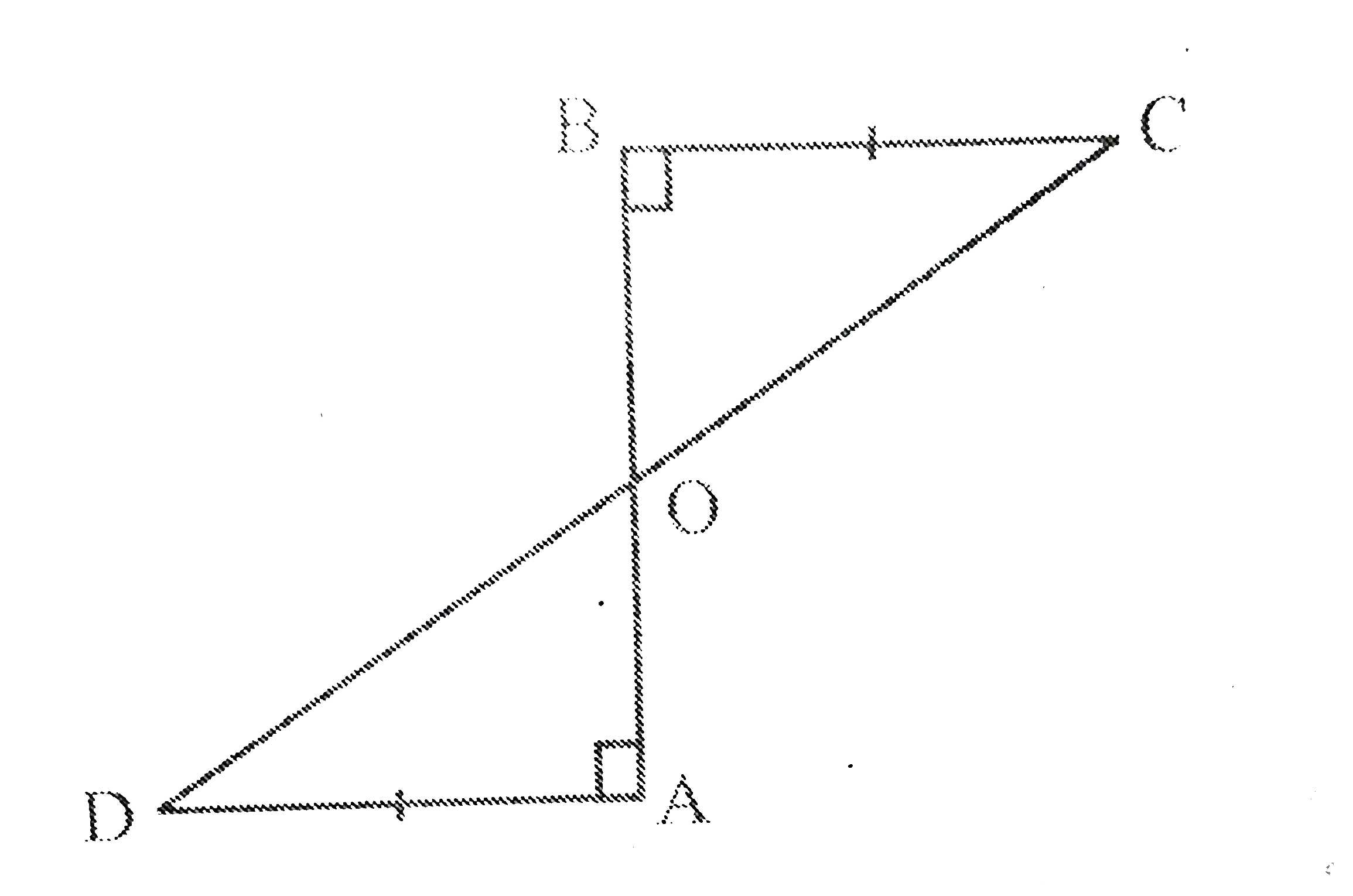
AD and BC are equal perpendiculars to a line segment AB . Show that CD bisects AB.
Given that
AD and BC are equal perpendiculars to a line segment AB
To prove CD bisects AB
proof
Two triangles △AOD and △BOC
∠AOD=abg≤BOC {Vertically opposite angles}………………. (i)
∠OAD=∠OBC {Given that they are perpendiculars}…. (ii)
AD=BC {As given}………… (iii)
From above three equation both the triangle satisfies “AAS” congruency criterion
So,△AOD≅△BOC
AO andBOwill be equal as they are corresponding parts of congruent triangles(CPCT).
So, AO=BO
Hence, CDbisects AB at O.
Topper's Solved these Questions
Similar Questions
AD and BC are equal perpendiculars to a line segment AB . Show that CD bisects AB.
View SolutionAD and BC are equal perpendiculars to a line segment AB . Show that CD bisects AB.
View SolutionAD and BC are equal perpendiculars to a line segment AB (see Fig. 7.18). Show that CD bisects AB.
View SolutionIn Figure, BM and DN are both perpendiculars to the segments AC and BM=DN . Prove that AC bisects BD
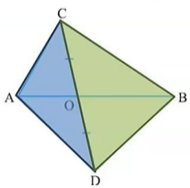
View SolutionBD is one of the diagonals of a quadrilateral ABCD. AM and CN are the perpendiculars from A and C , respectively, on BD. Show That ar (qua..ABCD)=12BD.AM+CN
View SolutionIf →a,→b,→care mutually perpendicular vectors of equal magnitudes, show that the vector →a+→b+→cis equally inclined to →a,→b and →c.
View Solutionp AND q are any two points lying on the sides DC and AD respectively of a parallelogram ABCD. Show that ar(APB)=ar (BQC).
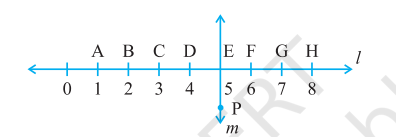
View SolutionStudy the diagram. The line l is perpendicular to line m (a) Is CE=EG (b) Does PE bisect CG = (c) Identify any two line segments for which PE is the perpendicular bisector. (d) Are these true? (i) AC>EG (ii) CD=GH (iii) BC<EH
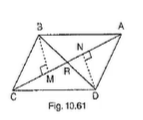
View SolutionIn Figure, ABCD is a parallelogram. E and F are the mid-points of the sides AB and CD respectively. Prove that the line segments AF and CE trisect (divide into three equal parts) the diagonal BD .
View SolutionABCD is a parallelogram and line segments AX,CY bisect the angles AandC, respectively. Show that AX∣∣CY
View Solution
