Following are four different relations about displacement, velocity and acceleration for the motion of a particle in general. Choose the incorrect one (s)
A
vav=12[v(t1)+v(t2)]
B
vav=r(t2)−r(t1)t2−t1
C
r=12(v(t2)−v(t1))(t2−t1)
D
aav=v(t2)−v(t1)t2−t1
Video Solution
Text Solution
The correct Answer is:A, C
If an object undergoes a displacement Δr in time Δt, its average velocity is given by
v=ΔrΔt=r2−r1t2−t1, where r1 and r2 are position vectors corresponding to time t1 and t2.
It the velocity of an object changes from v1 to v2 in time Δt. Average acceleration is given by
aav=ΔvΔt=v2−v1t2−t2
But , when acceleration is non-uniform
vav≠v1+v22
We can write Δv=ΔrΔt
Hence, Δr=r2−r1=(v2−v1)(t2−t1)
|
Updated on:21/07/2023
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Which of the following diagrams correctly relate displacement velocity and acceleration with time for a particle executing SHM
A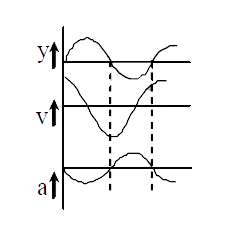 B
B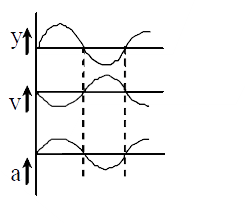 C
C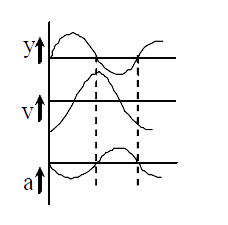 D
D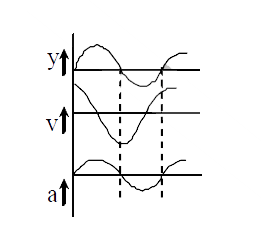
- Question 2 - Select One
The diplacement , velocity and acceleration in a simple harmonic motion are related as the
Adisplacement , velocity and acceleration all act in the same directionbBdisplacement and velocity act in the same direction but acceleration in the opposite direction.Cvelocity and acceleration are parallel and both are perpendicular to the displacementDdisplacement and acceleration are anti-parallel and both perpendicular to the velocity - Question 3 - Select One
The phase difference between displacement and acceleration of a particle in a simple harmonic motion is:
A3π2 radBπ2 radCZeroDπ rad
Discuss the general relations for velocity and acceleration for motion of an one dimension in a plane.
View SolutionThe phase difference between displacement and acceleration of particle in a simple harmonic motion is
View SolutionDeduce the relations for (a) particle velocity (b) particle acceleration
View SolutionThe phase difference between the instantaneous velocity and acceleration of a particle executing simple harmonic motion is
View SolutionThe phase difference between the instantaneous velocity and acceleration of a particle executing simple harmonic motion is
View Solution

